You place a particle of charge q at the origin and another of -2q at x = -d m. a. Write an expression for the potential at some arbitrary distance xp from the origin on the x axis. b. Similar to a), write an expression for the electric field anywhere along x axis.
You place a particle of charge q at the origin and another of -2q at x = -d m. a. Write an expression for the potential at some arbitrary distance xp from the origin on the x axis. b. Similar to a), write an expression for the electric field anywhere along x axis.
Related questions
Question
100%

Transcribed Image Text:**Charges and Potentials**
**Problem 1:** You place a particle of charge \( q \) at the origin and another of \( -2q \) at \( x = -d \) m.
a. Write an expression for the *potential* at some arbitrary distance \( x_p \) from the origin on the x-axis.
b. Similar to a), write an expression for the *electric field* anywhere along the x-axis.
---
**Problem 2:** A thin wire carries uniform charge \( q \) and is shaped into a circle of radius \( R \).
a. What is the magnitude of the electric field at the center of the circle? (*Hint: this one should be quick!*)
b. What is the value of the potential (referenced to 0 at infinity) at the center?
---
**Problem 3:** Consider an infinitely long cylinder with radius \( R \) and uniform surface charge density \( \sigma \).
a. Find the magnitude of the electric field at a distance \( s \) from the axis of the cylinder for \( s < R \).
b. Find the magnitude of the electric field at a distance \( s \) from the axis of the cylinder for \( s > R \).
c. *Using your answer to part b, find the potential difference between two points: \( s = a \) and \( s = b \).*
---
**Problem 4:** A thin rod of length \( l \) carries a uniformly distributed charge \( q \). The rod lies on the x-axis with its near end at \( x = +d \) and the far end of the rod at \( x = d + l \).
a. What is the magnitude of the electric field at the origin?
b. What is the electrostatic potential at the origin?
c. What is the potential at the origin if the rod is far away, i.e., \( d \gg l \)? If you know it, just write it down!
**Diagram Description:**
The diagram shows the x-axis with markers labeled: \( x = 0 \) for the origin, \( x = d \) for the starting point of the rod, and \( x = d + l \) for the end of the rod. The rod is depicted as a shaded rectangle along the x-axis from \( d \) to \( d + l \).
Expert Solution
Step 1
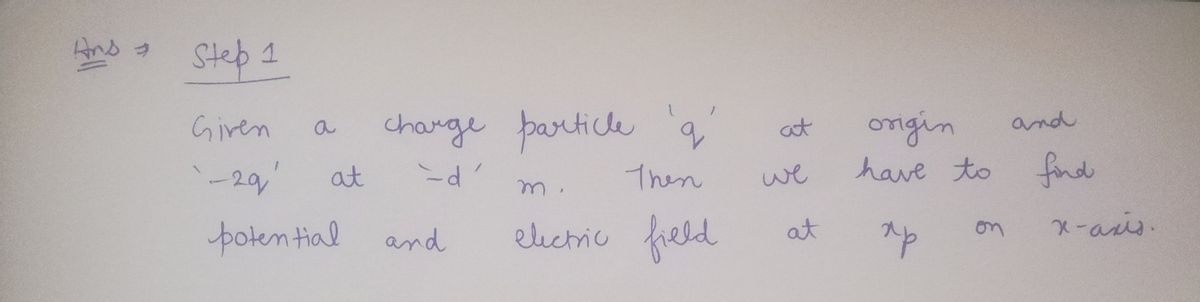
Step by step
Solved in 4 steps with 4 images
