Transformation of Graphs
The word ‘transformation’ means modification. Transformation of the graph of a function is a process by which we modify or change the original graph and make a new graph.
Exponential Functions
The exponential function is a type of mathematical function which is used in real-world contexts. It helps to find out the exponential decay model or exponential growth model, in mathematical models. In this topic, we will understand descriptive rules, concepts, structures, graphs, interpreter series, work formulas, and examples of functions involving exponents.
Use the graph of y = f(x) to graph the given function g:
g(x) = 2f(x + 2) - 1

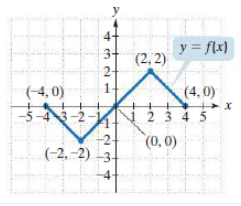
The graph of y = f(x) is
g(x) = 2f(x + 2) - 1
h(x+c) is the horizontally translated graph of h(x) to the left by c units.
The coordinates of point (x, y) of h(x) changes to (x+c, y).
ah(x) is the vertically stretched graph of h(x) if |a|>1.
If 0<|a|<1, then ah(x) is the vertically compressed graph of h(x)
The coordinates of point (x, y) of h(x) changes to (x, ay).
h(x)-d is the vertically translated graph of h(x) down by d units.
The coordinates of point (x, y) of h(x) changes to (x, y-d).
The points on the graph of y=f(x) are
(-4, 0), (-2, -2), (0, 0), (2, 2), (4, 0)
Let p(x)=f(x+2)
p(x)=f(x+2) is the horizontally translated graph of f(x) to the left by 2 units.
The coordinates of point (x, y) of h(x) changes to (x+2, y).
The point (-4, 0) changes to (-4+2, 0)=(-2, 0)
The point (-2, -2) changes to (-2+2, -2)=(0, -2)
The point (0, 0) changes to (0+2, 0)=(2, 0)
The point (2, 2) changes to (2+2, 2)=(4, 2)
The point (4, 0) changes to (4+2, 0)=(6, 0)
Step by step
Solved in 7 steps with 2 images









