X3.2.39 Question Help ▼ Compute the right-hand and left-hand derivatives as limits and check whether the function is differentiable at the point P. What is the right-hand derivative of the given function? f(4 + h) – f(4) lim = 1 Av 8- h→0* (Type an integer or a simplified fraction.) y = f(x) What is the left-hand derivative of the given function? y =x P(4,4) f(4 + h) – f(4) lim h h→0 y = Vx +2 (Type an integer or a simplified fraction.)
X3.2.39 Question Help ▼ Compute the right-hand and left-hand derivatives as limits and check whether the function is differentiable at the point P. What is the right-hand derivative of the given function? f(4 + h) – f(4) lim = 1 Av 8- h→0* (Type an integer or a simplified fraction.) y = f(x) What is the left-hand derivative of the given function? y =x P(4,4) f(4 + h) – f(4) lim h h→0 y = Vx +2 (Type an integer or a simplified fraction.)
Calculus: Early Transcendentals
8th Edition
ISBN:9781285741550
Author:James Stewart
Publisher:James Stewart
Chapter1: Functions And Models
Section: Chapter Questions
Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...
Related questions
Concept explainers
Rate of Change
The relation between two quantities which displays how much greater one quantity is than another is called ratio.
Slope
The change in the vertical distances is known as the rise and the change in the horizontal distances is known as the run. So, the rise divided by run is nothing but a slope value. It is calculated with simple algebraic equations as:
Question
Compute the right-hand and left-hand derivatives as limits and check whether the function is differentiable at the point P.
What is the left-hand derivative of the given function?

Transcribed Image Text:Math 181 Calculus I Spring 2021
Homework: Section 3.2 Assignment
Save
Score: 0 of 1 pt
11 of 13 (12 complete)
HW Score: 84.62%, 11 of 13 pts
3.2.39
Question Help ▼
Compute the right-hand and left-hand derivatives as limits
and check whether the function is differentiable at the point P.
What is the right-hand derivative of the given function?
f(4 + h) – f(4)
lim
= 1
h
8-
h→0*
(Type an integer or a simplified fraction.)
y = f(x)
What is the left-hand derivative of the given function?
y = x
P(4,4)
f(4 + h) – f(4)
lim
y= x +2
(Type an integer or a simplified fraction.)
I,
(1,1)
More
Enter your answer in the answer box and then click Check Answer.
1 part
remaining
Clear All
Final Check
Expert Solution
Step 1
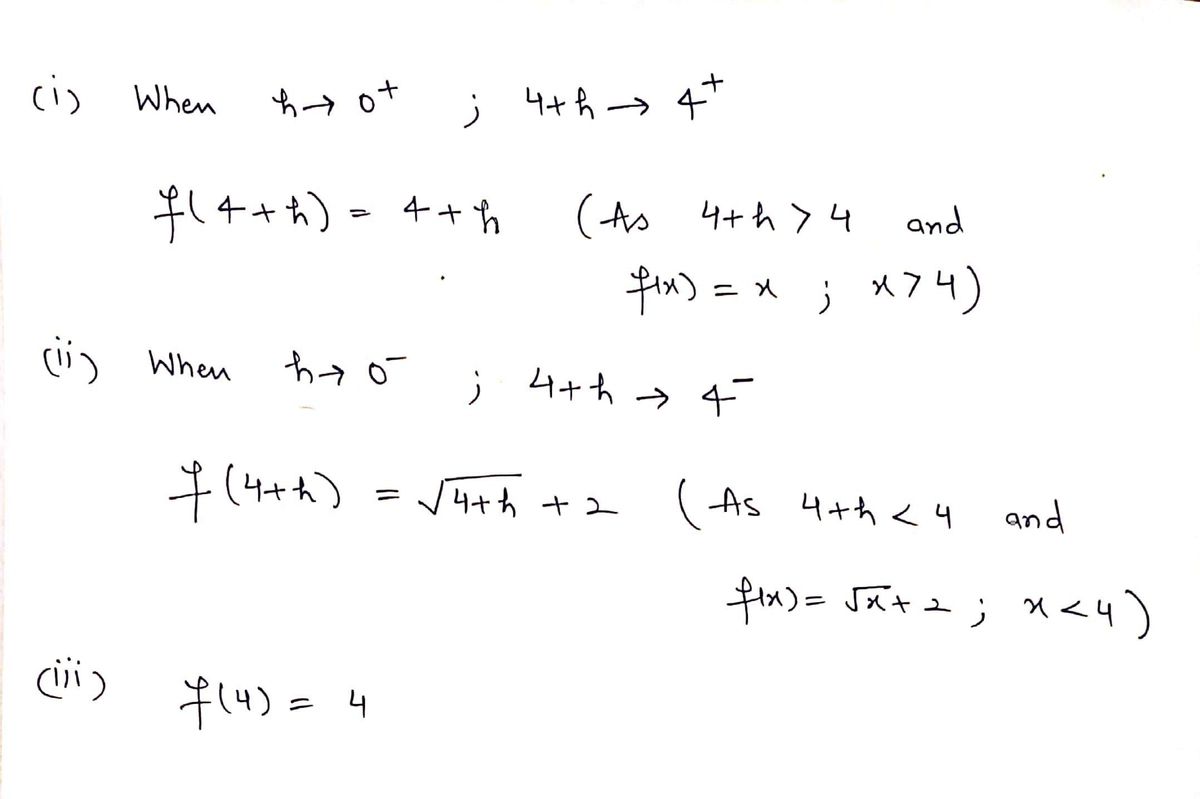
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Recommended textbooks for you

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781319050740
Author:
Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:
W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:
9781337552516
Author:
Ron Larson, Bruce H. Edwards
Publisher:
Cengage Learning