Calculus: Early Transcendentals
8th Edition
ISBN:9781285741550
Author:James Stewart
Publisher:James Stewart
Chapter1: Functions And Models
Section: Chapter Questions
Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...
Related questions
Question
![**Title: Simplifying Exponential Expressions**
**Objective:**
Learn how to write expressions in the form \(2^{kx}\) or \(3^{kx}\) by finding a suitable constant \(k\).
---
**Problem Statement:**
**Write each expression in the form \(2^{kx}\) or \(3^{kx}\) for a suitable constant \(k\).**
**(a)** \(\left(2^{-6x} \cdot 2^{-5x}\right)^{6/11}\)
---
**Procedure:**
1. **Simplifying Base Expressions:**
- Recall the law of exponents: \(a^m \cdot a^n = a^{m+n}\).
- For expression (a), combine the exponents of the base 2:
\[
2^{-6x} \cdot 2^{-5x} = 2^{(-6x) + (-5x)} = 2^{-11x}
\]
2. **Applying the Power of a Power Property:**
- Use the power of a power property: \((a^m)^n = a^{m \cdot n}\).
- Simplify the expression:
\[
\left(2^{-11x}\right)^{6/11} = 2^{-11x \cdot \frac{6}{11}} = 2^{-6x}
\]
3. **Determine the Suitable Constant \(k\):**
- The expression is now in the form \(2^{kx}\), where \(k = -6\).
**Final Expression for (a):**
\(\left(2^{-6x} \cdot 2^{-5x}\right)^{6/11} = 2^{-6x}\).
---
**(b)** \(\left(3^{1/3} \cdot 3^4\right)^{x/13}\)
*Note: The procedures for simplifying expression (b) would follow similar steps—starting with combining the exponents and then applying the power of a power property. However, as the problem is ungiven, approach it by combining exponents and simplifying accordingly.*
---
**Graph/Diagram Explanation:**
There are no graphs or diagrams in this image. The focus is on algebraic manipulation of exponential expressions.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fa8713e9b-73ac-4e4f-a322-df330d573c40%2F18083220-7395-4865-b298-3effcba67e34%2Fq0nsj54_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Title: Simplifying Exponential Expressions**
**Objective:**
Learn how to write expressions in the form \(2^{kx}\) or \(3^{kx}\) by finding a suitable constant \(k\).
---
**Problem Statement:**
**Write each expression in the form \(2^{kx}\) or \(3^{kx}\) for a suitable constant \(k\).**
**(a)** \(\left(2^{-6x} \cdot 2^{-5x}\right)^{6/11}\)
---
**Procedure:**
1. **Simplifying Base Expressions:**
- Recall the law of exponents: \(a^m \cdot a^n = a^{m+n}\).
- For expression (a), combine the exponents of the base 2:
\[
2^{-6x} \cdot 2^{-5x} = 2^{(-6x) + (-5x)} = 2^{-11x}
\]
2. **Applying the Power of a Power Property:**
- Use the power of a power property: \((a^m)^n = a^{m \cdot n}\).
- Simplify the expression:
\[
\left(2^{-11x}\right)^{6/11} = 2^{-11x \cdot \frac{6}{11}} = 2^{-6x}
\]
3. **Determine the Suitable Constant \(k\):**
- The expression is now in the form \(2^{kx}\), where \(k = -6\).
**Final Expression for (a):**
\(\left(2^{-6x} \cdot 2^{-5x}\right)^{6/11} = 2^{-6x}\).
---
**(b)** \(\left(3^{1/3} \cdot 3^4\right)^{x/13}\)
*Note: The procedures for simplifying expression (b) would follow similar steps—starting with combining the exponents and then applying the power of a power property. However, as the problem is ungiven, approach it by combining exponents and simplifying accordingly.*
---
**Graph/Diagram Explanation:**
There are no graphs or diagrams in this image. The focus is on algebraic manipulation of exponential expressions.
Expert Solution
Step 1: introduce the given problem
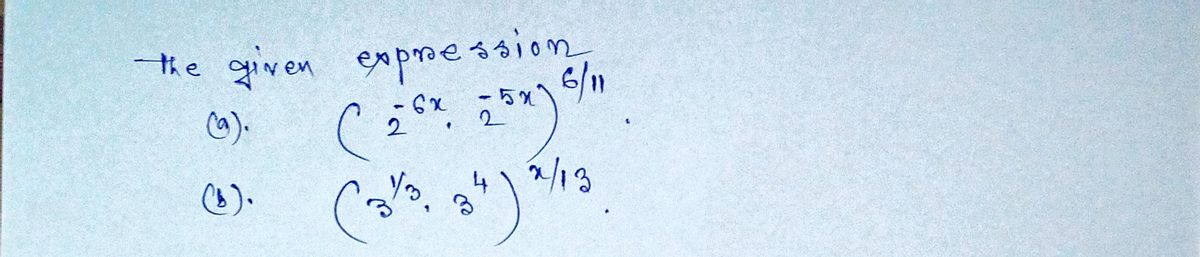
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781319050740
Author:
Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:
W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:
9781337552516
Author:
Ron Larson, Bruce H. Edwards
Publisher:
Cengage Learning