2. According to a survey taken by an agency in a rural area, it has been observed that 75% of population treats diseases through self-medication without consulting a physician. Among the 12 residents surveyed on a particular day, find the probability that, (a) At least two of them treat diseases through self-medication without consulting a physician. (b) Exactly 10 of them consults physician before taking medication. (c) None of them consults physician before taking medication. (d) Less than 10 residents consult physician before taking medication. (e) All of them treat diseases through self-medication without consulting a physician.
2. According to a survey taken by an agency in a rural area, it has been observed that 75% of population treats diseases through self-medication without consulting a physician. Among the 12 residents surveyed on a particular day, find the probability that, (a) At least two of them treat diseases through self-medication without consulting a physician. (b) Exactly 10 of them consults physician before taking medication. (c) None of them consults physician before taking medication. (d) Less than 10 residents consult physician before taking medication. (e) All of them treat diseases through self-medication without consulting a physician.
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question

Transcribed Image Text:2. According to a survey taken by an agency in a rural area, it has been observed that 75% of population
treats diseases through self-medication without consulting a physician. Among the 12 residents
surveyed on a particular day, find the probability that,
(a) At least two of them treat diseases through self-medication without consulting a physician.
(b) Exactly 10 of them consults physician before taking medication.
(c) None of them consults physician before taking medication.
(d) Less than 10 residents consult physician before taking medication.
(e) All of them treat diseases through self-medication without consulting a physician.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 7 steps with 15 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
I can see that you are changing the value of p and q depending on the question , is it ok to change them based on the question even though they are at the end from the same question just a different branch
in a. P was 0.75
in d . P was 0.25
![↑
P(x-x) = S(12²) (0-75)* (0.25)/2-X
Lo
Let
x~ bin (n=12, p=0.75)
pmf of x
a) P(x>2) = 1- P(X<2)
= 1- [P(x=0] +P(X=1)]
Yes, you can
=
this method also
use
--[Rc (0-15) (0+ 167¹ +1 (0-153(1-0155]
[126 (-0.75
12G
= 1- [(0.25) ¹²¹ + 12 (0-75) (0-25)" ]
=defeat
| P(x=2) = 0-9999978(
Hoxe x~ Bin (n=12, p=0·25)
P(x=x) = [(1/²) (025) * (0.75 ) ²-
or
P(x<10) = 1- P(x=10)
JX=0, J₁2, 12
JO.W.
P(x ≤ 10) = 0.999962
1x=0, J₁ 2₁ 12
10W.
= |- [P(X=10] +P(X=11] +P(x=12]]
= 1-[(1/2) (0251) (0 7514 (17)(0+25 1075 14+(12)(025 075)
Ext
=
Yes, you can do both method.
P(x < 10) = 1- P(x = 10)
= 1- [P(x=10) + P(X=11)+P(X=12)
-|- P(x = 10) - P(x= 11 ) - P(x=R)
=
Step 2
So you can do both methods, getting a same
answers from both types (methods).
So, do any one of them.
Your thinking is also correct
you do like
add them and then subtract them from one.](https://content.bartleby.com/qna-images/question/c04bb467-a0b2-4d57-9f91-8049096ec4e2/063dd0de-2eef-4b2f-86ca-bf5d5008b078/iabqkq_thumbnail.jpeg)
Transcribed Image Text:↑
P(x-x) = S(12²) (0-75)* (0.25)/2-X
Lo
Let
x~ bin (n=12, p=0.75)
pmf of x
a) P(x>2) = 1- P(X<2)
= 1- [P(x=0] +P(X=1)]
Yes, you can
=
this method also
use
--[Rc (0-15) (0+ 167¹ +1 (0-153(1-0155]
[126 (-0.75
12G
= 1- [(0.25) ¹²¹ + 12 (0-75) (0-25)" ]
=defeat
| P(x=2) = 0-9999978(
Hoxe x~ Bin (n=12, p=0·25)
P(x=x) = [(1/²) (025) * (0.75 ) ²-
or
P(x<10) = 1- P(x=10)
JX=0, J₁2, 12
JO.W.
P(x ≤ 10) = 0.999962
1x=0, J₁ 2₁ 12
10W.
= |- [P(X=10] +P(X=11] +P(x=12]]
= 1-[(1/2) (0251) (0 7514 (17)(0+25 1075 14+(12)(025 075)
Ext
=
Yes, you can do both method.
P(x < 10) = 1- P(x = 10)
= 1- [P(x=10) + P(X=11)+P(X=12)
-|- P(x = 10) - P(x= 11 ) - P(x=R)
=
Step 2
So you can do both methods, getting a same
answers from both types (methods).
So, do any one of them.
Your thinking is also correct
you do like
add them and then subtract them from one.
Solution
Follow-up Question
Sorry but, I am confused to why you subtract p( x= 0 ) - p(x=1)
why not add them and then subtract them from one like this:
1- p( p(x=0) + p(x=1))
? Can you elaborate
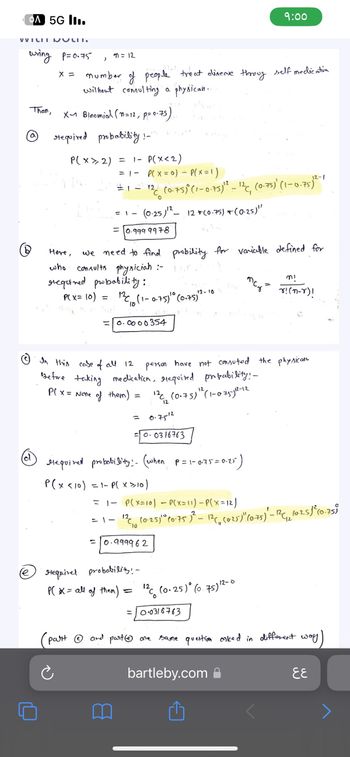
Transcribed Image Text:A 5G III.
VVICII POLII.
wring p=0.75
Than,
(@)
X =
)
n=12
X₁ Binomial (n=12, p=0-75)
stequired probability !_!!
1-
number of people treat disease throug self medication
without consulting a physical..
P(x > 2) = 1- P(X<2)
= 1 -
P(x = 0) - P(x = 1)
±1 - 126 (0.75) (1-0.75)¹2 - 12c, (0.75)' (1-0.75) 2-1
Ⓒ stequirel
= 0.999 9978
Here,
we need to find probility for variable defined for
who consults physician :-
required probability:
P(x= 10)
(0.25/¹² 12 * (0.75) + (0.25)"
12C 10 (1-0-75)¹⁰ (0.75)
0.0000354
Ⓒ In this case of all 12
person
Before taking medication, required probability! -
P(x = None of them)
126₂ (0.75) ¹² (1-0-75-112-12
0.75-12
0.0316763
=
12-10
siequired probability:- (when p=1-0.75= 0.25)
P ( x < 10) = 1- P(x >10)
stequired probability!
= 0.999962
-
9:00
mcr
= 1- P(x=10) - P(X=11) - P(X=12)
"
=1- 12₁ (0:25)¹0 (0.75 )² - 126, (025) " (075) - 12₁₂ (0.25) ² (0.75)
have not consuted the physican
P ( X = all of them) = 126 (0.25) ⁰ (0.75) 12-0
= 0·0316763
part Ⓒ and part are same question orked in
©
bartleby.com
mi
r! (n-r)!
different
way
६६
Solution
Follow-up Question
Why did you say p = 1-0.75 rather than p =0.75?
Solution
Follow-up Question
Is this right? I didn't understand your solution in a can elaborate
![Cie
2. p=0.75
8
(9) P(XX²)
de xto
• P. (X=0) =X)
• P(x=1)
=
P (X-72)
12
(1²)
$19 = 0.25
pise)
=
perfet
That
(12)
1-
x 0.75
1 X 1 X 0.25
2-145 x 10-
120 X (ose
Sieles
(P(X=0) + P(X= 1))
x 0.75
-6
12
X 0.12
1
O
(12-1)
X
x 10.25 (01 5X) CA
12
5.96X10-8
n=
11
10
x 0.25
53-5
= 0.999 299.9% 0
625
12
NOT
STY
1- (5.96× 10 -8 + 2+145 x 10-6)
1
10
0535 +
X
(b) Since the probabilty is exactly 10 of the 12 recidents
Consult the physican we can etter say say
q=0-45 Faiture
2103104
(0=x) ()
(1
2 reciclents don't
P don't consult: 0.750.09
00112-10
0.75
x (0.25)
(X=0
(X=10]
11=X) (
(01) *) q
92.0=1
@nsult
1677](https://content.bartleby.com/qna-images/question/c04bb467-a0b2-4d57-9f91-8049096ec4e2/014106a4-efac-48e1-8b57-3f687f9d2d51/6cgon2s_thumbnail.jpeg)
Transcribed Image Text:Cie
2. p=0.75
8
(9) P(XX²)
de xto
• P. (X=0) =X)
• P(x=1)
=
P (X-72)
12
(1²)
$19 = 0.25
pise)
=
perfet
That
(12)
1-
x 0.75
1 X 1 X 0.25
2-145 x 10-
120 X (ose
Sieles
(P(X=0) + P(X= 1))
x 0.75
-6
12
X 0.12
1
O
(12-1)
X
x 10.25 (01 5X) CA
12
5.96X10-8
n=
11
10
x 0.25
53-5
= 0.999 299.9% 0
625
12
NOT
STY
1- (5.96× 10 -8 + 2+145 x 10-6)
1
10
0535 +
X
(b) Since the probabilty is exactly 10 of the 12 recidents
Consult the physican we can etter say say
q=0-45 Faiture
2103104
(0=x) ()
(1
2 reciclents don't
P don't consult: 0.750.09
00112-10
0.75
x (0.25)
(X=0
(X=10]
11=X) (
(01) *) q
92.0=1
@nsult
1677
Solution
Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman