Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
Which of these statements never can be true? A) tanx = 500 B) Cosx =0,999 () sinxcosx 5,000 = Q) Sinx = cosx

Which of these statements never can be true? A) tanx = 500 B) Cosx =0,999 () sinxcosx 5,000 = Q) Sinx = cosx

Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN:9781133382119
Author:Swokowski
Publisher:Swokowski
Chapter6: The Trigonometric Functions
Section6.3: Trigonometric Functions Of Real Numbers
Problem 35E
See similar textbooks
icon
Related questions
Question
Which of these statements never can be true? A) tanx = 500 B) (05x = 0,999977 () sinxcosx= 5,000 Sinx = COSX D
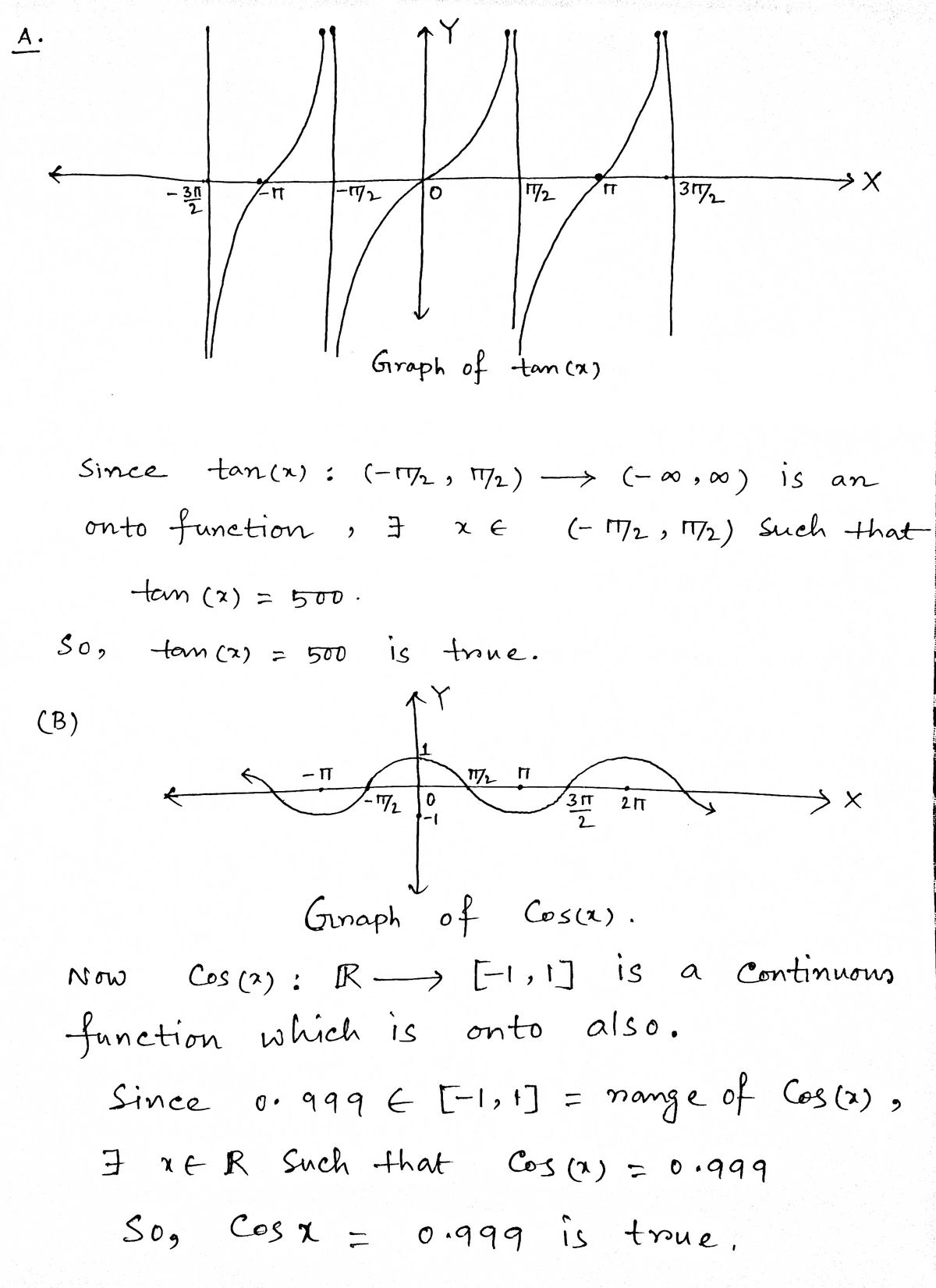
Transcribed Image Text:Which of these statements never can be true? A) tanx = 500 B) (05x = 0,999977 () sinxcosx= 5,000 Sinx = COSX D
Expert Solution
steps

Step by step

Solved in 3 steps with 2 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Recommended textbooks for you
Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:
9781133382119
Author:
Swokowski
Publisher:
Cengage
Trigonometry (MindTap Course List)
Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781305652224
Author:
Charles P. McKeague, Mark D. Turner
Publisher:
Cengage Learning
Algebra and Trigonometry (MindTap Course List)
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:
9781305071742
Author:
James Stewart, Lothar Redlin, Saleem Watson
Publisher:
Cengage Learning
Mathematics For Machine Technology
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
Trigonometry (MindTap Course List)
Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781337278461
Author:
Ron Larson
Publisher:
Cengage Learning
SEE MORE TEXTBOOKS