For the following problems, fill out as much information as possible. Statement Notation 1. If AABC is an isosceles triangle, then angle A is congruent to angle C. Given: Conclusion: Conclusion: Given: 2. If AB is an angle bisector Given: of ZMAT, then the angles created by the bisector are congruent. Given: Conclusion: Conclusion: Conclusion: 3. If AB is a perpendicular bisector of MN at point B, then MB is congruent to BN. Given: Given: Conclusion: A Picture B C

For the following problems, fill out as much information as possible. Statement Notation 1. If AABC is an isosceles triangle, then angle A is congruent to angle C. Given: Conclusion: Conclusion: Given: 2. If AB is an angle bisector Given: of ZMAT, then the angles created by the bisector are congruent. Given: Conclusion: Conclusion: Conclusion: 3. If AB is a perpendicular bisector of MN at point B, then MB is congruent to BN. Given: Given: Conclusion: A Picture B C

Elementary Geometry For College Students, 7e

7th Edition

ISBN:9781337614085

Author:Alexander, Daniel C.; Koeberlein, Geralyn M.

Publisher:Alexander, Daniel C.; Koeberlein, Geralyn M.

ChapterP: Preliminary Concepts

SectionP.CT: Test

Problem 1CT

See similar textbooks

Related questions

Q: Original A Original B

A: Given,Two figures.Aim: To draw different triangles that are congruent to each original by rotating…

Q: 1. Find the measure of the complement and supplement of an angle whose measure is: a. 78° b. A c.…

A: We are going to solve

Q: Find the measure of each angle in the problem. 43) Supplementary angles with measures 3x + 1 and 2x…

A: Supplementary angles are angles whose sum is equal to 180∘. An linear equation in one variable can…

Q: Find the measure of the smallest angle of AABC with a = = 15, b= 13, c 11.

A: Given query is to find the smallest angle.

Q: Quadrilateral ABCD and quadrilateral GHIJ are similar. If angle B=103, DA =28, CB=14, and IH=24,…

Q: A. Illustrate the two congruent triangle and mark its congruent parts based on the given. 1. AB a DE…

Q: How is the inscribed angle theorem used in the first step to prove that opposite angles of a…

Q: c m2A = a, mzB = 2a d m2A=60° + a, m2B=60° -α.

Q: In a right triangle ABC, <B = 17° , b = 31 feet and <C is a right angle. a.) REPRESENT this…

A: You have posted multiple questions. We will be answering only first question. If you need an answer…

Q: Question number 157

Q: in equilateral triangle CME, MB is an altitude and DE is an angle bisector. if BE=27-5x and DM=5x-3,…

Q: A-28. 3. |A| = 3, B = 8, and the angle formed by A and B is 45°. Determine A - 2B

A: We have to solve above question

Q: Find a positive and a negative coterminal angle for each given angle. 7) -45° 8) 270° 9) 365° 10)…

A: As per our guidelines at BartlebyGiven some angles in degrees. We have to find their positive and…

Q: Can you please answer all of the questions (they are pretty simple) and show work, highlight the…

Q: 3) classify the trangle based on its sides. 4) Classify the same triangle by its angles 1,2 23° 129…

Question

Statement
If two lines intersect,
creating vertical angles,
then the vertical angles are
congruent.
Given:
Conclusion:
If two lines intersect,
creating vertical angles,
then the vertical angles are
congruent.
Given: Two lines intersect,
creating vertical angles.
Conclusion: The vertical
angles are congruent.
Conclusion:
Conclusion:
Given: Two lines intersect,
creating vertical angles.
For the following problems, fill out as much information as possible.
Statement
Notation
1. If AABC is an isosceles
triangle, then angle A is
congruent to angle C.
Given:
Given:
Notation
Conclusion: ZNMA =
ZRMT
Conclusion:
Given:
2. If AB is an angle bisector Given:
of ZMAT, then the angles
created by the bisector are
congruent.
Given:
Conclusion:
3. If AB is a perpendicular
bisector of MN at point B,
then MB is congruent to BN.
Conclusion:
Given:
N
Conclusion:
N
Picture
A
M
Picture
B
M
R
C

Expert Solution

This question has been solved!

Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.

SEE SOLUTION Check out a sample Q&A here

Step 1: GIVEN DATA:

VIEW

Step 2: Explanation:1, 2

VIEW

Step 3: EXPLANATION-3

VIEW

Solution

VIEW

Step by step

Solved in 4 steps with 2 images

SEE SOLUTION Check out a sample Q&A here

Follow-up Questions

Read through expert solutions to related follow-up questions below.

Follow-up Question

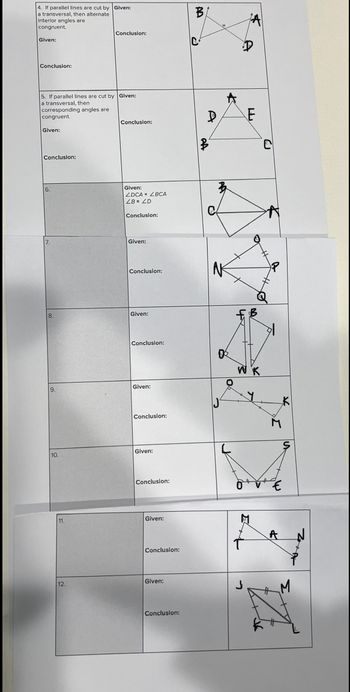
can you do this one like the one on top? Someone else did it but i dont understand it.

4. If parallel lines are cut by Given:
a transversal, then alternate
Interior angles are
congruent.
Given:
Conclusion:
5. If parallel lines are cut by Given:
a transversal, then.
corresponding angles are
congruent.
Given:
Conclusion:
6.
7.
8.
9.
10.
11.
Conclusion:
12.
Conclusion:
Given:
ZDCA ZBCA
ZB= 2D
Conclusion:
Given:
Conclusion:
Given:
Conclusion:
Given:
Conclusion:
Given:
Conclusion:
Given:
Conclusion:
Given:
Conclusion:
B
D
A
0
J₁
A
A
E
FB
W K
C
74
€
A

Solution

by Bartleby Expert

SEE SOLUTION

Follow-up Question

what do i put on the given and conclusion of the statement

Solution

by Bartleby Expert

SEE SOLUTION

Similar questions

3. The top and bottom of a five-foot high snowplow are to be attached to two steel braces of different lengths that meet at an angle of 44 degrees. If the length of one of the braces is 4.2 feet, at what angle does the shorter brace meet the blade? 4.2 Plaw SOLVE TrtE TRIAbrE, nteN ANSwrR TtE QUESTION
1. Given the diagram below, determine the angle made by the diagonals AD and BC. B
1. Explain why the triangles are similar and write a similarity statement. B A ZA = ZBED and ZC = ZBDE by the Alternate Interior Angles Theorem. AABC ~ AEBD by AA Similarity. b. a. ZA = ZBDE and ZC = ZBED by the Corresponding Angles Postulate. AABC ~ ADBE by AA Similarity. c. ZA = ZBDE and ZC = ZBED by the Corresponding Angles Postulate. AABC ~ AEBD by AA Similarity. d. ZA = ZBDE and 2C = ZBED by the Alternate Interior Angles Theorem. AABC ~ ADBE by AA Similarity.
Match the following items. Label the triangle using angle B. B 5 13 12 1. adjacent side 2. hypotenuse 3. opposite side 5 13 12