Verify the divergence theorem using the following vector field and volume. F = 4xi - 2y¹j + z²k and the volume V is the cylinder defined by the surfaces x² + y² = 4, z = 0 and z = 3.
Verify the divergence theorem using the following vector field and volume. F = 4xi - 2y¹j + z²k and the volume V is the cylinder defined by the surfaces x² + y² = 4, z = 0 and z = 3.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
100%
![**Title: Verification of the Divergence Theorem**
**Objective:**
Verify the divergence theorem using the given vector field and specified volume.
**Vector Field:**
\[ \mathbf{F} = 4x\mathbf{i} - 2y\mathbf{j} + z^2\mathbf{k} \]
**Volume:**
The volume \( V \) is defined as the cylinder bounded by the surfaces:
\[ x^2 + y^2 = 4, \, z = 0, \, \text{and} \, z = 3. \]
**Explanation:**
The divergence theorem relates the flux of a vector field through a closed surface to the divergence of the field within the volume enclosed by the surface. In this example, the vector field \(\mathbf{F}\) and the cylindrical volume \(V\) are provided. The goal is to compute both the surface integral and the volume integral to verify the theorem.
The cylinder has a circular base of radius 2 and extends vertically from \(z = 0\) to \(z = 3\). The top and bottom surfaces are the planes \(z = 3\) and \(z = 0\), respectively. The lateral surface is defined by the circular boundary \(x^2 + y^2 = 4\).
**Steps for Verification:**
1. Calculate the divergence of the vector field \(\mathbf{F}\).
2. Evaluate the volume integral of the divergence over \(V\).
3. Calculate the surface integral of the vector field \(\mathbf{F}\) over the closed surface of the cylinder.
4. Compare the results to verify the divergence theorem.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F3bf1c5b3-57c2-46ee-9184-9ffa514a30cb%2F8065c694-dfa6-45eb-ac66-5365ced5c7c7%2Fr8h80td_processed.png&w=3840&q=75)
Transcribed Image Text:**Title: Verification of the Divergence Theorem**
**Objective:**
Verify the divergence theorem using the given vector field and specified volume.
**Vector Field:**
\[ \mathbf{F} = 4x\mathbf{i} - 2y\mathbf{j} + z^2\mathbf{k} \]
**Volume:**
The volume \( V \) is defined as the cylinder bounded by the surfaces:
\[ x^2 + y^2 = 4, \, z = 0, \, \text{and} \, z = 3. \]
**Explanation:**
The divergence theorem relates the flux of a vector field through a closed surface to the divergence of the field within the volume enclosed by the surface. In this example, the vector field \(\mathbf{F}\) and the cylindrical volume \(V\) are provided. The goal is to compute both the surface integral and the volume integral to verify the theorem.
The cylinder has a circular base of radius 2 and extends vertically from \(z = 0\) to \(z = 3\). The top and bottom surfaces are the planes \(z = 3\) and \(z = 0\), respectively. The lateral surface is defined by the circular boundary \(x^2 + y^2 = 4\).
**Steps for Verification:**
1. Calculate the divergence of the vector field \(\mathbf{F}\).
2. Evaluate the volume integral of the divergence over \(V\).
3. Calculate the surface integral of the vector field \(\mathbf{F}\) over the closed surface of the cylinder.
4. Compare the results to verify the divergence theorem.
Expert Solution
Step 1
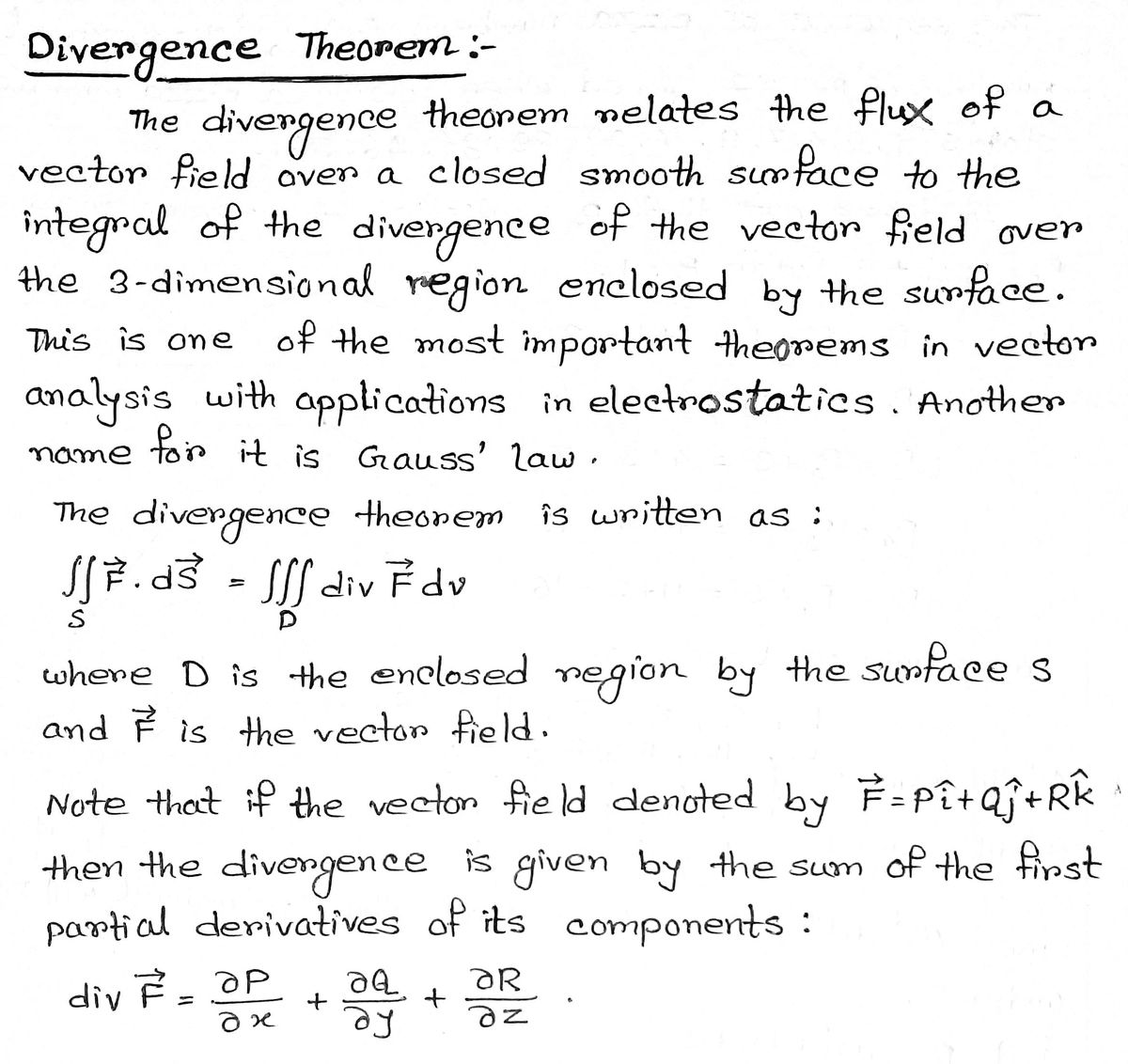
Step by step
Solved in 3 steps with 5 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

