var(Â), the mean square error (MSE) in estimating λ, and its exact sampling distribution? (e) Compute the Fisher information In in the sample data. Accordingly, obtain the asymp- totic distribution of Â.
var(Â), the mean square error (MSE) in estimating λ, and its exact sampling distribution? (e) Compute the Fisher information In in the sample data. Accordingly, obtain the asymp- totic distribution of Â.
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question
100%
Just E please
![2. Suppose that \( X_1, \ldots, X_n \) form a random sample from an Exponential(\(\lambda\)) distribution, with PDF given by
\[
f(x|\lambda) = \lambda e^{-\lambda x} \quad \text{for } x > 0, \, \lambda > 0.
\]
In the above form, \(\lambda\) is a rate parameter and \(E(X) = 1/\lambda\) and \(\text{var}(X) = 1/\lambda^2\).
(a) Find a MOM (method of moment) estimator \(\tilde{\lambda}\) of \(\lambda\).
(b) Write down the likelihood function \(L_n(\lambda)\) and obtain the maximum likelihood estimator (MLE) \(\hat{\lambda}\) of \(\lambda\).
(c) Let \(\tau\) denote the population median. Obtain the MLE of \(\tau\). (*Hint:* First find the specific form of the median in terms of \(\lambda\) and utilize the invariance property of MLE)
(d) Is it easy to study the finite-sample distributional property of the MLE \(\hat{\lambda}\), such as \(E(\hat{\lambda})\), \(\text{var}(\hat{\lambda})\), the mean square error (MSE) in estimating \(\lambda\), and its exact sampling distribution?
(e) Compute the Fisher information \(I_n\) in the sample data. Accordingly, obtain the asymptotic distribution of \(\hat{\lambda}\).
(f) Obtain a sufficient statistic \(T(X_1, \ldots, X_2)\) for \(\lambda\) via the factorization theorem. Verify that the MLE \(\hat{\lambda}\) is a function of \(T\).](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fc58b2eee-5b79-4c9d-923b-d4e913d44dbe%2F6a90cd4e-1449-4225-8e35-83b75267ad81%2Fx32cuq_processed.png&w=3840&q=75)
Transcribed Image Text:2. Suppose that \( X_1, \ldots, X_n \) form a random sample from an Exponential(\(\lambda\)) distribution, with PDF given by
\[
f(x|\lambda) = \lambda e^{-\lambda x} \quad \text{for } x > 0, \, \lambda > 0.
\]
In the above form, \(\lambda\) is a rate parameter and \(E(X) = 1/\lambda\) and \(\text{var}(X) = 1/\lambda^2\).
(a) Find a MOM (method of moment) estimator \(\tilde{\lambda}\) of \(\lambda\).
(b) Write down the likelihood function \(L_n(\lambda)\) and obtain the maximum likelihood estimator (MLE) \(\hat{\lambda}\) of \(\lambda\).
(c) Let \(\tau\) denote the population median. Obtain the MLE of \(\tau\). (*Hint:* First find the specific form of the median in terms of \(\lambda\) and utilize the invariance property of MLE)
(d) Is it easy to study the finite-sample distributional property of the MLE \(\hat{\lambda}\), such as \(E(\hat{\lambda})\), \(\text{var}(\hat{\lambda})\), the mean square error (MSE) in estimating \(\lambda\), and its exact sampling distribution?
(e) Compute the Fisher information \(I_n\) in the sample data. Accordingly, obtain the asymptotic distribution of \(\hat{\lambda}\).
(f) Obtain a sufficient statistic \(T(X_1, \ldots, X_2)\) for \(\lambda\) via the factorization theorem. Verify that the MLE \(\hat{\lambda}\) is a function of \(T\).
Expert Solution
Step 1
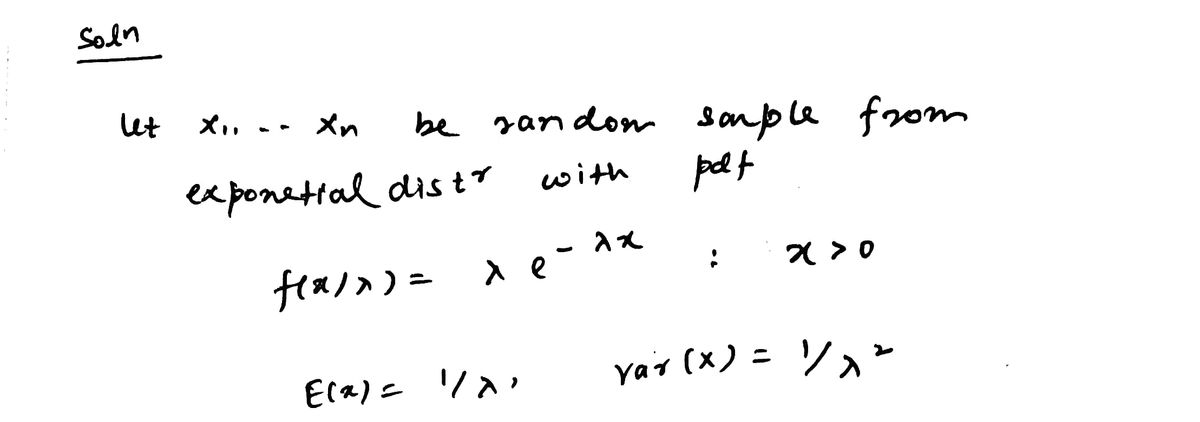
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman