Use the first derivative test to locate the relative extrema of the function in the given domain, and determine the intervals of g(x) = x³ 12x with domain [-4, 4] (a) Find the coordinates of the critical points and endpoints for the following function on the given interval. (Order your answers so the x-coordinates are in order from least to greatest.) - g has an end point at (x, y) = g has a critical point at (x, y) = g has a critical point at (x, y) = ( g has an end point (b) List the intervals on which f is increasing or decreasing, in order. g is increasing ✔ on the interval g is decreasing on the interval g is increasing on the intervall ✓ ✓ ✔at (x, y) = (c) Classify the critical points and end points. (Order your answers so the x-coordinates are in order from least to greatest.) g has a relative minimum ✔✔✔ at (x, y) = v g has a relative maximum v ✓at (x, y) = g has a relative minimum✔✔ at (x, y) = | g has a relative maximum at (x, y)= ( Submit Answer v
Use the first derivative test to locate the relative extrema of the function in the given domain, and determine the intervals of g(x) = x³ 12x with domain [-4, 4] (a) Find the coordinates of the critical points and endpoints for the following function on the given interval. (Order your answers so the x-coordinates are in order from least to greatest.) - g has an end point at (x, y) = g has a critical point at (x, y) = g has a critical point at (x, y) = ( g has an end point (b) List the intervals on which f is increasing or decreasing, in order. g is increasing ✔ on the interval g is decreasing on the interval g is increasing on the intervall ✓ ✓ ✔at (x, y) = (c) Classify the critical points and end points. (Order your answers so the x-coordinates are in order from least to greatest.) g has a relative minimum ✔✔✔ at (x, y) = v g has a relative maximum v ✓at (x, y) = g has a relative minimum✔✔ at (x, y) = | g has a relative maximum at (x, y)= ( Submit Answer v
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![**Educational Website Transcription**
---
**Topic: Analyzing the Function \( g(x) = x^3 - 12x \) on the Interval [-4, 4]**
### Instructions:
Use the first derivative test to locate the relative extrema of the function in the given domain, and determine the intervals of increase and decrease.
### Problem:
1. **Find the coordinates of the critical points and endpoints for the following function on the given interval**. (Order your answers so the x-coordinates are in order from least to greatest.)
- \( g \) has **an end point** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a critical point** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a critical point** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **an end point** at \((x, y) = ( \ \ \ , \ \ \ )\).
2. **List the intervals on which \( f \) is increasing or decreasing, in order.**
- \( g \) is **increasing** on the interval \([ \ \ \ , \ \ \ ]\).
- \( g \) is **decreasing** on the interval \([ \ \ \ , \ \ \ ]\).
- \( g \) is **increasing** on the interval \([ \ \ \ , \ \ \ ]\).
3. **Classify the critical points and end points.** (Order your answers so the x-coordinates are in order from least to greatest.)
- \( g \) has **a relative minimum** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a relative maximum** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a relative minimum** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a relative maximum** at \((x, y) = ( \ \ \ , \ \ \ )\).
### Submission:
- Once completed, please submit your answers using](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fc3182f1c-7375-4a50-8088-f06a8a8e9532%2F3bdc96d8-fd1f-460c-93ec-072357c84d2a%2Fmnugwk9_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Educational Website Transcription**
---
**Topic: Analyzing the Function \( g(x) = x^3 - 12x \) on the Interval [-4, 4]**
### Instructions:
Use the first derivative test to locate the relative extrema of the function in the given domain, and determine the intervals of increase and decrease.
### Problem:
1. **Find the coordinates of the critical points and endpoints for the following function on the given interval**. (Order your answers so the x-coordinates are in order from least to greatest.)
- \( g \) has **an end point** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a critical point** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a critical point** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **an end point** at \((x, y) = ( \ \ \ , \ \ \ )\).
2. **List the intervals on which \( f \) is increasing or decreasing, in order.**
- \( g \) is **increasing** on the interval \([ \ \ \ , \ \ \ ]\).
- \( g \) is **decreasing** on the interval \([ \ \ \ , \ \ \ ]\).
- \( g \) is **increasing** on the interval \([ \ \ \ , \ \ \ ]\).
3. **Classify the critical points and end points.** (Order your answers so the x-coordinates are in order from least to greatest.)
- \( g \) has **a relative minimum** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a relative maximum** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a relative minimum** at \((x, y) = ( \ \ \ , \ \ \ )\).
- \( g \) has **a relative maximum** at \((x, y) = ( \ \ \ , \ \ \ )\).
### Submission:
- Once completed, please submit your answers using
Expert Solution
Step 1: Definitions
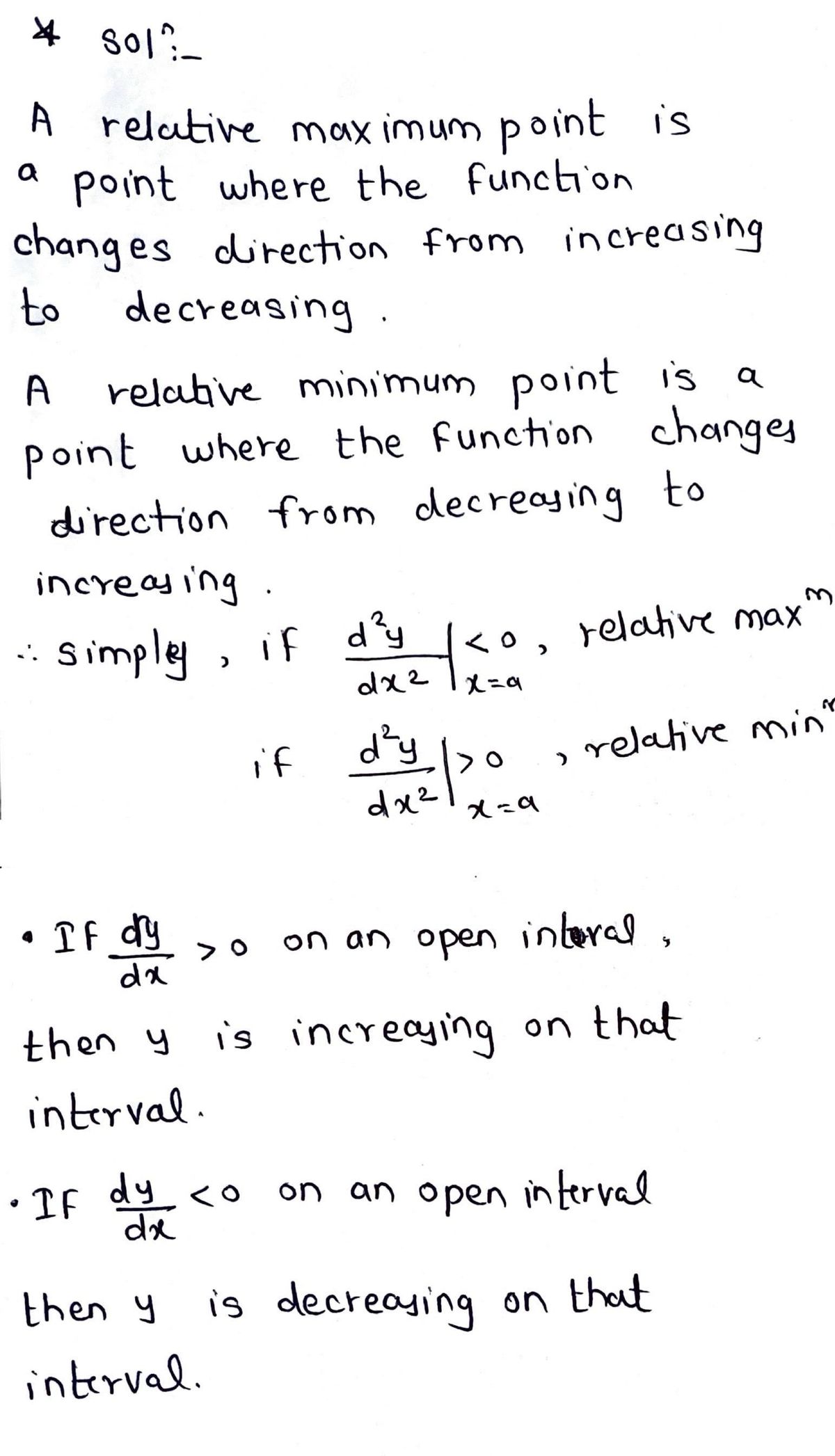
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

