Calculus: Early Transcendentals
8th Edition
ISBN:9781285741550
Author:James Stewart
Publisher:James Stewart
Chapter1: Functions And Models
Section: Chapter Questions
Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...
Related questions
Question
100%
Use Epsilon Delta Definition to prove lim x goes to 2, x^3 = 8
![**Using the ε-δ definition to prove the limit:**
**Problem Statement:**
Use the ε-δ definition to prove \(\lim_{{x \to 2}} x^3 = 8\).
**Explanation:**
The ε-δ definition of a limit states that for every \(\epsilon > 0\), there exists a \(\delta > 0\) such that if \(0 < |x - c| < \delta\), then \(|f(x) - L| < \epsilon\). Here, \(c = 2\) and the function \(f(x) = x^3\) with the limit \(L = 8\).
**Proof:**
To show:
\[
\forall \epsilon > 0, \exists \delta > 0 \text{ such that } 0 < |x - 2| < \delta \Rightarrow |x^3 - 8| < \epsilon
\]
1. **Start with the goal:**
\[
|x^3 - 8| < \epsilon
\]
Since:
\[
|x^3 - 8| = |(x - 2)(x^2 + 2x + 4)|
\]
We need this to be:
\[
|x - 2| |x^2 + 2x + 4| < \epsilon
\]
2. **Finding Bounds for \( |x^2 + 2x + 4| \):**
Choose a small enough \(|x - 2| < 1\), hence, \(1 < x < 3\).
3. **Bounding \( |x^2 + 2x + 4| \) when \(1 < x < 3\):**
When \(1 < x < 3\):
\[
x^2 < 9, \quad 2x < 6, \quad \text{and} \quad 4 \text{ is constant}
\]
So,
\[
|x^2 + 2x + 4| < 9 + 6 + 4 = 19
\]
Thus:
\[
|x^2 + 2x + 4| \leq 19
\]
4. **Relating \(|x-2|\) and \(\epsilon\):**
Given:
\[
|x -](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fc88a8d3c-414f-44f0-8ef5-044fbd549dcd%2Fe58b0274-ea31-46aa-8fe6-ff96e1286679%2F6p2bio.png&w=3840&q=75)
Transcribed Image Text:**Using the ε-δ definition to prove the limit:**
**Problem Statement:**
Use the ε-δ definition to prove \(\lim_{{x \to 2}} x^3 = 8\).
**Explanation:**
The ε-δ definition of a limit states that for every \(\epsilon > 0\), there exists a \(\delta > 0\) such that if \(0 < |x - c| < \delta\), then \(|f(x) - L| < \epsilon\). Here, \(c = 2\) and the function \(f(x) = x^3\) with the limit \(L = 8\).
**Proof:**
To show:
\[
\forall \epsilon > 0, \exists \delta > 0 \text{ such that } 0 < |x - 2| < \delta \Rightarrow |x^3 - 8| < \epsilon
\]
1. **Start with the goal:**
\[
|x^3 - 8| < \epsilon
\]
Since:
\[
|x^3 - 8| = |(x - 2)(x^2 + 2x + 4)|
\]
We need this to be:
\[
|x - 2| |x^2 + 2x + 4| < \epsilon
\]
2. **Finding Bounds for \( |x^2 + 2x + 4| \):**
Choose a small enough \(|x - 2| < 1\), hence, \(1 < x < 3\).
3. **Bounding \( |x^2 + 2x + 4| \) when \(1 < x < 3\):**
When \(1 < x < 3\):
\[
x^2 < 9, \quad 2x < 6, \quad \text{and} \quad 4 \text{ is constant}
\]
So,
\[
|x^2 + 2x + 4| < 9 + 6 + 4 = 19
\]
Thus:
\[
|x^2 + 2x + 4| \leq 19
\]
4. **Relating \(|x-2|\) and \(\epsilon\):**
Given:
\[
|x -
Expert Solution
Step 1
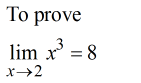
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 4 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Recommended textbooks for you

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781319050740
Author:
Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:
W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:
9781337552516
Author:
Ron Larson, Bruce H. Edwards
Publisher:
Cengage Learning