Calculus: Early Transcendentals
8th Edition
ISBN:9781285741550
Author:James Stewart
Publisher:James Stewart
Chapter1: Functions And Models
Section: Chapter Questions
Problem 1RCC: (a) What is a function? What are its domain and range? (b) What is the graph of a function? (c) How...
Related questions
Question
![**Title: Using Lagrange Multipliers to Find the Shortest Distance**
**Objective:**
Find the shortest distance from the point \((7, 0, -9)\) to the plane \(x + y + z = 1\).
**Method: Lagrange Multipliers**
Lagrange multipliers are a powerful technique for finding the local maxima and minima of a function subject to equality constraints.
### Explanation:
1. **Function to Minimize:**
We want to minimize the distance from the point \((x, y, z)\) to \((7, 0, -9)\). The distance squared is:
\[
f(x, y, z) = (x - 7)^2 + y^2 + (z + 9)^2
\]
2. **Constraint:**
The constraint given by the plane is:
\[
g(x, y, z) = x + y + z - 1 = 0
\]
3. **Lagrange Function:**
Introduce a Lagrange multiplier, \(\lambda\), and define the Lagrangian:
\[
\mathcal{L}(x, y, z, \lambda) = (x - 7)^2 + y^2 + (z + 9)^2 + \lambda(x + y + z - 1)
\]
4. **Set Up Equations:**
Take the partial derivatives and set them equal to zero to find critical points:
\[
\frac{\partial \mathcal{L}}{\partial x} = 2(x - 7) + \lambda = 0
\]
\[
\frac{\partial \mathcal{L}}{\partial y} = 2y + \lambda = 0
\]
\[
\frac{\partial \mathcal{L}}{\partial z} = 2(z + 9) + \lambda = 0
\]
\[
\frac{\partial \mathcal{L}}{\partial \lambda} = x + y + z - 1 = 0
\]
5. **Solve System of Equations:**
Solve these equations simultaneously to find the values of \(x\), \(y\), \(z\), and \(\lambda\) that minimize the](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fe040b111-4b10-4c24-8337-c45ed461242d%2F7ceb40f9-fefd-4c25-aa56-6392d7eb2d09%2Fbtb86f6_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Title: Using Lagrange Multipliers to Find the Shortest Distance**
**Objective:**
Find the shortest distance from the point \((7, 0, -9)\) to the plane \(x + y + z = 1\).
**Method: Lagrange Multipliers**
Lagrange multipliers are a powerful technique for finding the local maxima and minima of a function subject to equality constraints.
### Explanation:
1. **Function to Minimize:**
We want to minimize the distance from the point \((x, y, z)\) to \((7, 0, -9)\). The distance squared is:
\[
f(x, y, z) = (x - 7)^2 + y^2 + (z + 9)^2
\]
2. **Constraint:**
The constraint given by the plane is:
\[
g(x, y, z) = x + y + z - 1 = 0
\]
3. **Lagrange Function:**
Introduce a Lagrange multiplier, \(\lambda\), and define the Lagrangian:
\[
\mathcal{L}(x, y, z, \lambda) = (x - 7)^2 + y^2 + (z + 9)^2 + \lambda(x + y + z - 1)
\]
4. **Set Up Equations:**
Take the partial derivatives and set them equal to zero to find critical points:
\[
\frac{\partial \mathcal{L}}{\partial x} = 2(x - 7) + \lambda = 0
\]
\[
\frac{\partial \mathcal{L}}{\partial y} = 2y + \lambda = 0
\]
\[
\frac{\partial \mathcal{L}}{\partial z} = 2(z + 9) + \lambda = 0
\]
\[
\frac{\partial \mathcal{L}}{\partial \lambda} = x + y + z - 1 = 0
\]
5. **Solve System of Equations:**
Solve these equations simultaneously to find the values of \(x\), \(y\), \(z\), and \(\lambda\) that minimize the
Expert Solution
Step 1
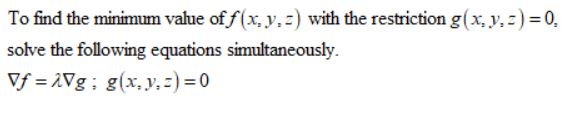
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781285741550
Author:
James Stewart
Publisher:
Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:
9780134438986
Author:
Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:
PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:
9780134763644
Author:
William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:
PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:
9781319050740
Author:
Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:
W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:
9781337552516
Author:
Ron Larson, Bruce H. Edwards
Publisher:
Cengage Learning