Following the usual notation, derive a formula for the 3rd central moment in terms of the raw moments. The moment generating function (mgf) of the Negative Binomial distribution with parameters p and k is given as M(t)= p 1-(1-p)e' ¬k Use this mgf to derive general formulae for the mean and variance of the Negative Binomial distribution Show all the stens involved in your derivation.

Following the usual notation, derive a formula for the 3rd central moment in terms of the raw moments. The moment generating function (mgf) of the Negative Binomial distribution with parameters p and k is given as M(t)= p 1-(1-p)e' ¬k Use this mgf to derive general formulae for the mean and variance of the Negative Binomial distribution Show all the stens involved in your derivation.

MATLAB: An Introduction with Applications

6th Edition

ISBN:9781119256830

Author:Amos Gilat

Publisher:Amos Gilat

Chapter1: Starting With Matlab

Section: Chapter Questions

Problem 1P

See similar textbooks

Related questions

Q: Suppose you are given the moment generating function M(t) =- for t< In 3 4-3e Find the mean and…

A: The first derivative of moment generating function is, The mean of the distribution will be: Thus,…

Q: Under some circumstances the waiting time X for an event could be modeled with the following pdf:…

A: Given information: f(x)=θ801-x80θ-1, 0<x<80 Given θ=4 a. CDF of X: F(X)=∫0xf(t)dt…

Q: 3(x+ x³) for Let f(x) = for 0< x < 2, zero otherwise be the pdf for a random variable X. 14 d) Find…

A: Introduction: The median is the value below which, the probability of obtaining an observation is…

Q: Let X be a discrete random variable with moment generating function given by: Mx (1) = (0.21+0.79e¹)…

A: Given that Moment generating function of X is Mx(t)=(0.21+0.79et)18.

Q: Assume that the entire sample has 4 positive observations and 2 negatives observations. Variable…

A: Given Number of positive observations=4 Number of negative observations=2 Left branch : P=9, N=4…

Q: Let X₁, X₂, X3,..., Xn be a random Sample from N (μ,0²) Use maximum likelihood estimation to find…

A: Xi ~N(μ, σ2); i=1,2,...,n

Q: A survey was conducted to examine social media usage of males versus females. The results are…

A: The following information has been provided. The row and column total have been calculated and they…

Q: Q6. Let us assume that the lifetime of light bulbs follows an exponential distribution with the pdf:…

A: Given, The exponential distribution pdf is given, f(x)=λ×e(-λx) We test 10 bulbs and their lifetimes…

Q: Based on an analysis of sample data, an article proposed the pdf f(x) = 0.55e-0.55(x - 1) when x 2 1…

A: Let X be the time spent at the median line.Given that, the pdf of X is

Q: *An actuary determines that the claim size for a certain class of ac- cidents is a random variable,…

A: it can be solved by

Q: Identify the distributions (and their parameters) whose moment-generating functions are given below.…

A: Moment Generating Function (MGF): The moment generating function (MGF) of a random variable X is…

Q: When a man observed a sobriety checkpoint conducted by a police department, he saw 676 drivers were…

Q: f(x, y): ) = {K(x² + x²) [K(x² + y²) 20 ≤ x ≤ 30, 20 ≤ y ≤ 30 otherwise (a) Determine the…

A: Given: The front tire on a particular type of vehicle is filled with the pressure of 26 psi .…

Q: How many tails are involved in the determination of the critical z value in the marketing research…

A: According to the given information in this question We need to identify how many tails involved in…

Q: Please answer all part : Let rt be a log return. Suppose that r0, r1, . . . are i.i.d. N(0, 0.01^2).…

A: Let a random variable rt be a log return.The r0, r1, r2,......... are independent identical normal…

Q: Let Let Y₁, Y2, Y3 be the order statistics of a random sample of size 3 from distribution having p…

Q: If X ∼ Gamma(2, 5), find E(X^2 ) andE(X^4 )

A: Solution: Let X follows gamma distribution with the parameters 2 and 5.

Q: I model the time at work as a Gamma distribution with parameters r = 20, = 2. What is the average…

Q: Let X be the arrival time in minutes of the next red traffic light on a certain delivery route. We…

Q: F(144) Generation Y has been defined as those individuals who were born between 1981 and 1991. A…

A: Population proportion : P=0.60 Sample size : n=190 Sample proportion : p p follows normal…

Q: 4. Let X₁ and X₂ be independent identically distributed random variables both having exponential…

A: Let X1 and X2 be independent and identically distributed random variables both having an exponential…

Question

Define the rth central moment (ur) and rth raw moment (ar).
Following the usual notation, derive a formula for the 3rd central moment in
terms of the raw moments.
The moment generating function (mgf) of the Negative Binomial distribution
with parameters p and k is given as
M(t)
p
1-(1 - p)e'
k
Use this mgf to derive general formulae for the mean and variance of the
Negative Binomial distribution. Show all the steps involved in your derivation.

Expert Solution

This question has been solved!

Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.

SEE SOLUTION Check out a sample Q&A here

Step 1: Mention the given data in the question

VIEW

Step 2: Find the third central moment

VIEW

Step 3: Calculate mean and variance

VIEW

Solution

VIEW

Step by step

Solved in 4 steps with 11 images

SEE SOLUTION Check out a sample Q&A here

Follow-up Questions

Read through expert solutions to related follow-up questions below.

Follow-up Question

this is a follow up question

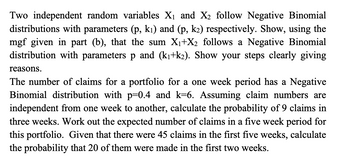
Two independent random variables X1 and X2 follow Negative Binomial
distributions with parameters (p, k₁) and (p, k2) respectively. Show, using the
mgf given in part (b), that the sum X₁+X2 follows a Negative Binomial
distribution with parameters p and (k₁+k2). Show your steps clearly giving
reasons.
The number of claims for a portfolio for a one week period has a Negative
Binomial distribution with p=0.4 and k=6. Assuming claim numbers are
independent from one week to another, calculate the probability of 9 claims in
three weeks. Work out the expected number of claims in a five week period for
this portfolio. Given that there were 45 claims in the first five weeks, calculate
the probability that 20 of them were made in the first two weeks.

Solution

by Bartleby Expert

SEE SOLUTION

Similar questions

The moment generating function can be used to find the mean and variance of the normal distribution. Use derivatives of Mx(t) to verify that E(X) = µ and V(X) = 0².
Assume that the entire sample has 8 positive observations and 4 negatives observations. Variable X1: at the left branch has 9 positive observations and 3 negatives observations;at the right branch has 8 positive observations and 8 negative observations. What is the information gain or reduction in uncertainty of X1 using the Gini index? (round to two decimal spaces)
The hypotenuse, Y, of the isosceles right triangle shown 0 is a random variable having a uniform pdf over the interval [5, 10]. Calculate the expected value of the triangle's area. Do not leave the answer as a function of a. (round to 3 decimal places)
Q5 Find the variance for the PDF px(x) = e-«/2, x > 0.
Let X ~ Uniform(a, b). Find Expected value EX.