Two different brands of milk are randomly sampled, and the fat content in each bottle is determined. Twenty-six bottles of Brand A yielded an average fat content of 26 grams with a standard
Two different brands of milk are randomly sampled, and the fat content in each bottle is determined. Twenty-six bottles of Brand A yielded an average fat content of 26 grams with a standard
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question
Two different brands of milk are randomly sampled, and the fat content in each bottle is determined. Twenty-six bottles of Brand A yielded an average fat content of 26 grams with a standard deviation of 5 grams, and 31 bottles of Brand B yielded an average fat content of 25.8 grams with a standard deviation of 7 grams. Test the hypothesis at the 10% level of significance that the variances are different.

Transcribed Image Text:6) Two different brands of milk are randomly sampled, and the fat content in each bottle
of milk is determined. Twenty six bottles of Brand A yielded an average fat content
of 26 grams with a standard deviation of 5 grams, and 31 bottles of Brand B yielded
an average fat content of 25.8 grams with a standard deviation of 7 grams. Test the
hypothesis at the 10% level of significance that the variances are different.
Expert Solution
Step 1
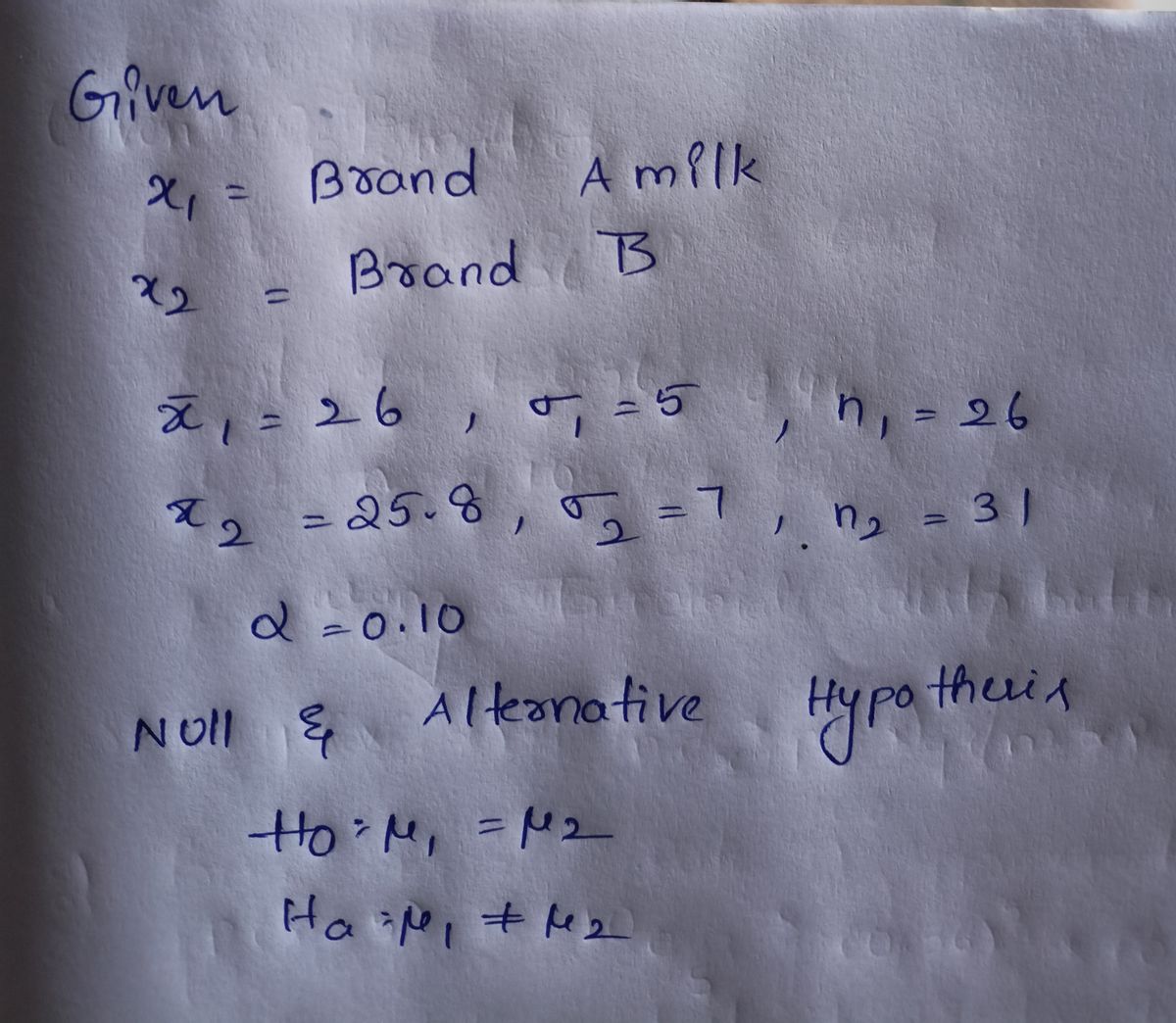
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman