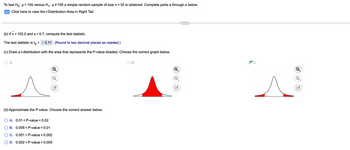
To test H9: u= 105 versus H,: u# 105 a simple random sample of size n= 35 is obtained. Complete parts a through e below. Click here to view the t-Distribution Area in Right Tail. (a) Does the population have to be normally distributed to test this hypothesis? Why? A. No, because n2 30. O B. Yes, because n2 30. OC. No, because the test is two-tailed. O D. Yes, because the sample is random. (b) If x= 102.0 and s= 5.8, compute the test statistic. The test statistic is to (Round to two decimal places as needed.)
Continuous Probability Distributions
Probability distributions are of two types, which are continuous probability distributions and discrete probability distributions. A continuous probability distribution contains an infinite number of values. For example, if time is infinite: you could count from 0 to a trillion seconds, billion seconds, so on indefinitely. A discrete probability distribution consists of only a countable set of possible values.
Normal Distribution
Suppose we had to design a bathroom weighing scale, how would we decide what should be the range of the weighing machine? Would we take the highest recorded human weight in history and use that as the upper limit for our weighing scale? This may not be a great idea as the sensitivity of the scale would get reduced if the range is too large. At the same time, if we keep the upper limit too low, it may not be usable for a large percentage of the population!

Trending now
This is a popular solution!
Step by step
Solved in 2 steps