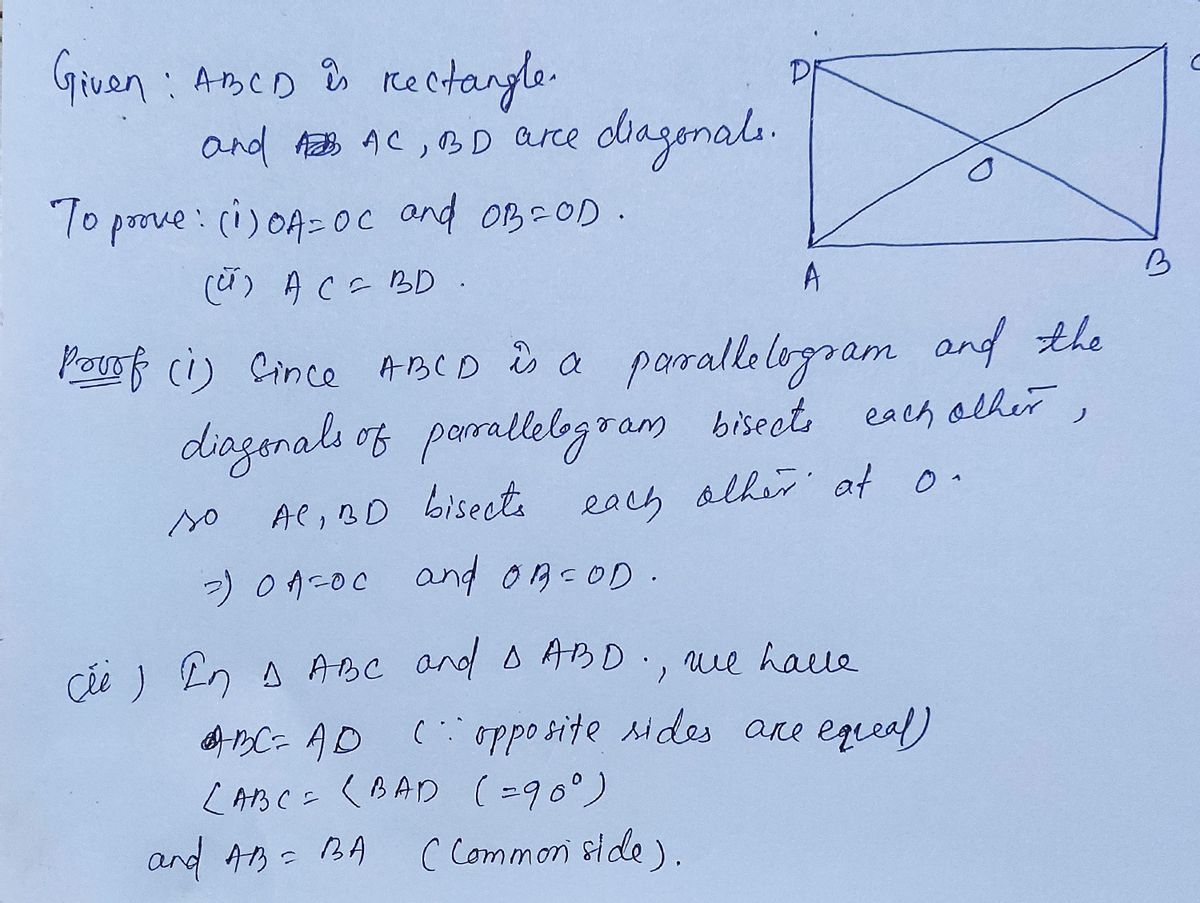
Theorem. The diagonals of a rectangle bisect each other and have the same length.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
draw the diagrams
file in the blanks

Transcribed Image Text:**Theorem.** *The diagonals of a rectangle bisect each other and have the same length.*
**Proof.** Let \( \square ABCD \) be a rectangle, i.e., a quadrilateral that has insert defining characteristic. Draw diagonals \( \overline{AC} \) and \( \overline{BD} \).
*Insert figure that illustrates the setup*
Explain why the diagonals bisect each other. (Do not work too hard. Make use of a combination of theorems we have already proven.)
Next we will show the diagonals have the same length. Note that \( \overline{AC} \) is a side of triangle blah and \( \overline{BD} \) is a side of triangle blah. Thus it suffices to show triangles blah and blah are congruent because then their corresponding sides will be congruent.
*Insert figure highlighting the two triangles of interest*
Write an argument for why the triangles you identified in the previous step are congruent.
*Insert a diagram that uses color or line style to mark the segments or angles you are using to justify congruence of the triangles*
Therefore the corresponding sides \( \overline{AC} \) and \( \overline{BD} \) are congruent. Thus the diagonals of a rectangle bisect each other and have the same length.
□
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

