The values in the column for the degrees of freedom are based on the number of population means, or treatments, and the total number of observations, N. In the sum of squares column, SST represents a measure of differences among the sample means. The value of SSE is a measure of variability within the samples. Note that the values in the mean square column are calculated based on the sum of squares and degrees freedom for the treatments row and emorrow. These mean square values are then used to calculate the test statistic. The value is based on the best statistic with&- 1 numerator degrees of freedom and N-& denominator degrees of freedom. The given data are below Treatment 1 Treatment 2 Treatment 3 Treatment 4 • D . 12 There are treatments, so we have and t A total of 18 men underwent Treatment 1 giving 18. Treatment 2 was made up of 25 men, giving m degrees of freedom for Treatments Continuing in this fashion, the sample size of Treatment 3 in, and the sample sue of Treatment is Thus, the degrees of freedom for Error is N-k The total number of men in the experiment is N, ₂₂₂
The values in the column for the degrees of freedom are based on the number of population means, or treatments, and the total number of observations, N. In the sum of squares column, SST represents a measure of differences among the sample means. The value of SSE is a measure of variability within the samples. Note that the values in the mean square column are calculated based on the sum of squares and degrees freedom for the treatments row and emorrow. These mean square values are then used to calculate the test statistic. The value is based on the best statistic with&- 1 numerator degrees of freedom and N-& denominator degrees of freedom. The given data are below Treatment 1 Treatment 2 Treatment 3 Treatment 4 • D . 12 There are treatments, so we have and t A total of 18 men underwent Treatment 1 giving 18. Treatment 2 was made up of 25 men, giving m degrees of freedom for Treatments Continuing in this fashion, the sample size of Treatment 3 in, and the sample sue of Treatment is Thus, the degrees of freedom for Error is N-k The total number of men in the experiment is N, ₂₂₂
A First Course in Probability (10th Edition)
10th Edition
ISBN:9780134753119
Author:Sheldon Ross
Publisher:Sheldon Ross
Chapter1: Combinatorial Analysis
Section: Chapter Questions
Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...
Related questions
Question
4. Please answer the blanks ty!!

Transcribed Image Text:The values in the column for the degrees of freedom are based on the number of k population means, or treatments, and the total number of observations, N. In the sum of squares column, SSTr represents a measure of differences among the sample means. The value of SSE is a measure of variability within the k samples. Note that the values in the mean square column are calculated based on the sum of squares and degrees of
freedom for the treatments row and error row. These mean square values are then used to calculate the F test statistic. The P-value is based on the F test statistic with k- 1 numerator degrees of freedom and N-k denominator degrees of freedom.
The given data are below.
Treatment 1
9
7
3
14
2
0
9
12
0
2
5
6
3
15
7
5
11
Treatment 2
5
9
0
3
8
9
5
1
6
9
9
13
8
0
5
4
11
7
5
10
9
5
1
10
Treatment 3
2
0
4
0
4
3
3
6
6
5
9
0
4
Treatment 4
There are 4 treatments, so we have k = 4 and k-1 = |
A total of 18 men underwent Treatment 1 giving n, = 18.
Treatment 2 was made up of 25 men, giving n₂ =
Continuing in this fashion, the sample size of Treatment 3 is n =
Thus, the degrees of freedom for Error is N - k =
5
1
5
6
5
1
0
0
4
2
1
2
3
3
degrees of freedom for Treatments.
, and the sample size of Treatment 4 is n₂ =
The total number of men in the experiment is N = n
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 4 steps

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
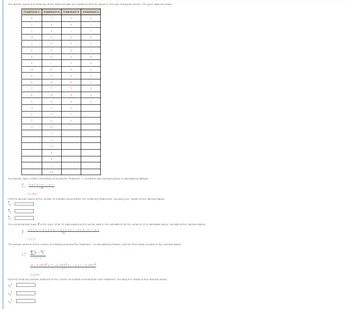
Transcribed Image Text:The sample means and variances of the observed data are needed to find the values in the sum of squares column. The given data and below.
Treatment
9
7
3
}
24
4
2
0
9
12
O
2
S
6
3
15
7
S
21
Treatment 2 Treatment 3
LA
S
9
D
n-1
1000
3
B
9
S
4
4
9
7
13
R
D
S
A
44
7
5
D
S
1
2
4
4
74
(9 – 6.1667)² + (7
6
4
S
3
6712 + (7 = 6.166712 +
– 6.1667)²
Treatment +
4
The sample mean number of pretzels consumed for Treatment 1, rounded to four decimal places, is calculated as follows.
S
D
6.1667
Find the sample means of the number of pretzale consumed for the remaining treatments, rounding your results to four decimal places.
72
5₂
4
The overall sample means the mean of all 74 observations and it will be used in the calculations for the variance. It is calculated below, rounded to four decimal places.
2
4
2
The sample variance of the number of pretzels consumed for Treatment is calculated as follows, with the final result rounded to four decimal places.
+---+ (11 – 6.166732
22.0294
find the remaining sample variances of the number of pretzale consumed for each treatment, rounding the results to four decimal places
Solution
Recommended textbooks for you

A First Course in Probability (10th Edition)
Probability
ISBN:
9780134753119
Author:
Sheldon Ross
Publisher:
PEARSON


A First Course in Probability (10th Edition)
Probability
ISBN:
9780134753119
Author:
Sheldon Ross
Publisher:
PEARSON
