Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
the resulting zero-ends (homogeneous) heat boundary value problem c² a²u (10) 0 < x 0, Ət (11) u(0,t) = 0_and_u(L,t)=0, t>0, u(x, 0) = f(x) — u₁(x), 0 < x < L. (12) Let u₂(x, t) be the solution of (10)-(12). According to (4) and (5), we have (13) where An ди = (15) cn and L' - Əx², u₂(x, t): = 2 bn = f(f(x) - ∞ n=1 bne -X²/2t u1 (r) T2 - T₁ L NTT sin -X, L (14) Now the solution of (6)–(8) is obtained by adding to u₂(x, t) the steady-state solution u₁(x) as follows: -x+ T₁)) sin x dx. u(x, t) = u₁(x) + u₂(x, t).

the resulting zero-ends (homogeneous) heat boundary value problem c² a²u (10) 0 < x 0, Ət (11) u(0,t) = 0_and_u(L,t)=0, t>0, u(x, 0) = f(x) — u₁(x), 0 < x < L. (12) Let u₂(x, t) be the solution of (10)-(12). According to (4) and (5), we have (13) where An ди = (15) cn and L' - Əx², u₂(x, t): = 2 bn = f(f(x) - ∞ n=1 bne -X²/2t u1 (r) T2 - T₁ L NTT sin -X, L (14) Now the solution of (6)–(8) is obtained by adding to u₂(x, t) the steady-state solution u₁(x) as follows: -x+ T₁)) sin x dx. u(x, t) = u₁(x) + u₂(x, t).

Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
See similar textbooks
icon
Related questions
Question
100%

please also show solution satisfies boundry conditions, intial conditions, and the PDE. Thanks!

the resulting zero-ends (homogeneous) heat boundary value problem = c² 0²u Əx², (10) du Ət 0 < x < L, t> 0, (11) u(0, t) = 0 and u(L, t) = 0, t> 0, (12) u(x, 0) = f(x) — u₁(x), 0<x< L. Let u₂(x, t) be the solution of (10)-(12). According to (4) and (5), we have (13) where An = cn and L' u₂(x, t) = n=1 bne -X²2/t u₁(x) Nπ sin -X, L T₂ - T₁ bn = ²/ fő (f(x) - ( -x+ T₁)) sin x dx. L (14) Now the solution of (6)-(8) is obtained by adding to u₂(x, t) the steady-state solution u₁(x) as follows: (15) u(x, t) = u₁(x) + u₂(x, t).
Transcribed Image Text:the resulting zero-ends (homogeneous) heat boundary value problem = c² 0²u Əx², (10) du Ət 0 < x < L, t> 0, (11) u(0, t) = 0 and u(L, t) = 0, t> 0, (12) u(x, 0) = f(x) — u₁(x), 0<x< L. Let u₂(x, t) be the solution of (10)-(12). According to (4) and (5), we have (13) where An = cn and L' u₂(x, t) = n=1 bne -X²2/t u₁(x) Nπ sin -X, L T₂ - T₁ bn = ²/ fő (f(x) - ( -x+ T₁)) sin x dx. L (14) Now the solution of (6)-(8) is obtained by adding to u₂(x, t) the steady-state solution u₁(x) as follows: (15) u(x, t) = u₁(x) + u₂(x, t).
In Exercises 11-14, solve the nonhomogeneous boundary value problem (6)–(8) for the given data. 11. u(0, t) = 100, u(1, t) = 0, ƒ(x) = 30 sin(πx), L = 1, c = 1.
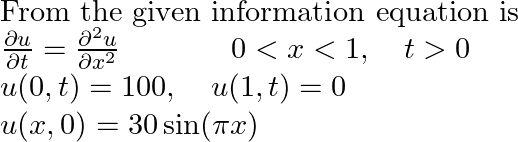
Transcribed Image Text:In Exercises 11-14, solve the nonhomogeneous boundary value problem (6)–(8) for the given data. 11. u(0, t) = 100, u(1, t) = 0, ƒ(x) = 30 sin(πx), L = 1, c = 1.
Expert Solution
Step 1

Advanced Math homework question answer, step 1, image 1

steps

Step by step

Solved in 3 steps with 3 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
Basic Technical Mathematics
Basic Technical Mathematics
Advanced Math
ISBN:
9780134437705
Author:
Washington
Publisher:
PEARSON
Topology
Topology
Advanced Math
ISBN:
9780134689517
Author:
Munkres, James R.
Publisher:
Pearson,
SEE MORE TEXTBOOKS