The mean value theorem. If f(x) is continuous in [a, b] and differentiable in (a, b), then there exists a point ě in (a, b) such that f(b)- f(a) - f'(E) a <
Equations and Inequations
Equations and inequalities describe the relationship between two mathematical expressions.
Linear Functions
A linear function can just be a constant, or it can be the constant multiplied with the variable like x or y. If the variables are of the form, x2, x1/2 or y2 it is not linear. The exponent over the variables should always be 1.
6.14) my professor says I have to explain the steps in the solved problems in the picture. Not just copy eveything down from the text.
![The mean value theorem. If f(x) is continuous in [a, b] and differentiable in (a, b), then there exists
a point č in (a, b) such that
f(b)- f(a) - f'E) a<5 <b
(16)
b-a
Rolle's theorem is the special case of this where f(a) = f(b) = 0.
The result (16) can be written in various alternative forms; for example, if x and x, are in (a, b),
then
f(x) = f(xo) +f'(Š)(x– x) between x, and x
We can also write result (16) with b = a + h, in which case = a + Oh, where 0< 0 < 1.
(17)
The mean value theorem is also called the law of the mean.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fad530511-9758-42d9-9994-894bc4eea3f3%2Fc9eddfe4-2e9e-40f0-b184-af37bc68fe01%2Fpavugx_processed.jpeg&w=3840&q=75)

Let us suppose that the graph of the function is
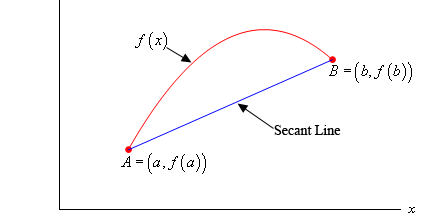
Then the equation of the secant line represented by red in the graph that goes through the two points is
We will now define a new function as the difference between and the secant line i.e.
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 1 images









