Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
Solve this set of nonlinear equation by hand (Newton's Method): f₁(x, y) = 4y² + 1.02x³ f₂(x, y) = -2.1x² + y² Use the initial values (1,0), to solve this problem by hand. Show only four steps by hand.

Solve this set of nonlinear equation by hand (Newton's Method): f₁(x, y) = 4y² + 1.02x³ f₂(x, y) = -2.1x² + y² Use the initial values (1,0), to solve this problem by hand. Show only four steps by hand.

Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
See similar textbooks
icon
Related questions
Question

Numerical Methods

Please can you solve this question?

Quoted from LORAN (LOng RAnge Navigation) system The LORAN (LOng RAnge Navigation) system calculates the position of a boat at sea using signals from fixed transmitters. From the time differences of the incoming signals, the boat obtains differences of distances to the transmitters. This leads to two equations each representing hyperbolas defined by the differences of distance of two points (foci). An example of such equations are: f₁(x, y) = f₂(x, y) = y² 300-186² (y-500)² 2792 + x2 186² = 1 (x-300)² (500²-279)² = 1 Solving two quadratic equations with two unknowns, would require solving a 4 degree polynomial equation. We could do this by hand, but for a navigational system to work well, it must do the calculations automatically and numerically. We note that the Global Positioning System (GPS) works on similar principles and must do similar computations. Solve this set of nonlinear equation by hand (Newton's Method): f₁(x, y) = 4y² + 1.02x³ f₂(x, y) = −2.1x² + y³ Use the initial values (1,0), to solve this problem by hand. Show only four steps by hand.
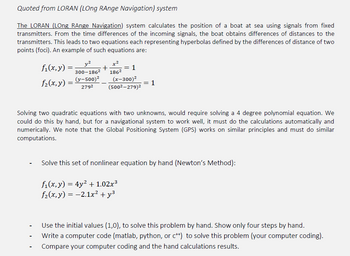
Transcribed Image Text:Quoted from LORAN (LOng RAnge Navigation) system The LORAN (LOng RAnge Navigation) system calculates the position of a boat at sea using signals from fixed transmitters. From the time differences of the incoming signals, the boat obtains differences of distances to the transmitters. This leads to two equations each representing hyperbolas defined by the differences of distance of two points (foci). An example of such equations are: f₁(x, y) = f₂(x, y) = y² 300-186² (y-500)² 2792 + x2 186² = 1 (x-300)² (500²-279)² = 1 Solving two quadratic equations with two unknowns, would require solving a 4 degree polynomial equation. We could do this by hand, but for a navigational system to work well, it must do the calculations automatically and numerically. We note that the Global Positioning System (GPS) works on similar principles and must do similar computations. Solve this set of nonlinear equation by hand (Newton's Method): f₁(x, y) = 4y² + 1.02x³ f₂(x, y) = −2.1x² + y³ Use the initial values (1,0), to solve this problem by hand. Show only four steps by hand.
Expert Solution
steps

Step by step

Solved in 2 steps with 2 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question

- Write a computer code (matlab, python, or c++) to solve this problem (your computer coding).
- Compare your computer coding and the hand calculations results.

Quoted from LORAN (LOng RAnge Navigation) system The LORAN (LOng RAnge Navigation) system calculates the position of a boat at sea using signals from fixed transmitters. From the time differences of the incoming signals, the boat obtains differences of distances to the transmitters. This leads to two equations each representing hyperbolas defined by the differences of distance of two points (foci). An example of such equations are: f₁(x, y) f₂(x, y) = = y² 300-186² (y-500)² 2792 + = 1 186² (x-300)² (500²-279)² = 1 Solving two quadratic equations with two unknowns, would require solving a 4 degree polynomial equation. We could do this by hand, but for a navigational system to work well, it must do the calculations automatically and numerically. We note that the Global Positioning System (GPS) works on similar principles and must do similar computations. Solve this set of nonlinear equation by hand (Newton's Method): f₁(x, y) = 4y² +1.02x³ f₂(x, y) = -2.1x² + y² Use the initial values (1,0), to solve this problem by hand. Show only four steps by hand. Write a computer code (matlab, python, or c++) to solve this problem (your computer coding). Compare your computer coding and the hand calculations results.
Transcribed Image Text:Quoted from LORAN (LOng RAnge Navigation) system The LORAN (LOng RAnge Navigation) system calculates the position of a boat at sea using signals from fixed transmitters. From the time differences of the incoming signals, the boat obtains differences of distances to the transmitters. This leads to two equations each representing hyperbolas defined by the differences of distance of two points (foci). An example of such equations are: f₁(x, y) f₂(x, y) = = y² 300-186² (y-500)² 2792 + = 1 186² (x-300)² (500²-279)² = 1 Solving two quadratic equations with two unknowns, would require solving a 4 degree polynomial equation. We could do this by hand, but for a navigational system to work well, it must do the calculations automatically and numerically. We note that the Global Positioning System (GPS) works on similar principles and must do similar computations. Solve this set of nonlinear equation by hand (Newton's Method): f₁(x, y) = 4y² +1.02x³ f₂(x, y) = -2.1x² + y² Use the initial values (1,0), to solve this problem by hand. Show only four steps by hand. Write a computer code (matlab, python, or c++) to solve this problem (your computer coding). Compare your computer coding and the hand calculations results.
Solution
Bartleby Expert
SEE SOLUTION
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
Basic Technical Mathematics
Basic Technical Mathematics
Advanced Math
ISBN:
9780134437705
Author:
Washington
Publisher:
PEARSON
Topology
Topology
Advanced Math
ISBN:
9780134689517
Author:
Munkres, James R.
Publisher:
Pearson,
SEE MORE TEXTBOOKS