Homework Help is Here – Start Your Trial Now!
Science
Advanced Physics
The initial wave function of a particle in an infinite square well of width L is given by: 25 (x,0) = √√√₂+ ( 46 (x) + 24 (X) ] 42 and 44 ts are second and fourth stationary states. Energy is given by En = n²E a) Find 4(x, t) b) Find c) Find expectation value of H. C)

The initial wave function of a particle in an infinite square well of width L is given by: 25 (x,0) = √√√₂+ ( 46 (x) + 24 (X) ] 42 and 44 ts are second and fourth stationary states. Energy is given by En = n²E a) Find 4(x, t) b) Find c) Find expectation value of H. C)

icon
Related questions
Question
100%

If you could help me with solution 

The initial wave function of a particl in an infinite square well of width L is given by: 25 (x,0) = √3/2² ( 4₂ (X) + 24 (X) ] Is 4₂ and 44 ks are second and fourth stationary states, Energy is given by En = h²E₁ a) Find 4(x, t) b) Find <p> c) Find expectation value of H.
Transcribed Image Text:The initial wave function of a particl in an infinite square well of width L is given by: 25 (x,0) = √3/2² ( 4₂ (X) + 24 (X) ] Is 4₂ and 44 ks are second and fourth stationary states, Energy is given by En = h²E₁ a) Find 4(x, t) b) Find <p> c) Find expectation value of H.
Expert Solution
Step 1

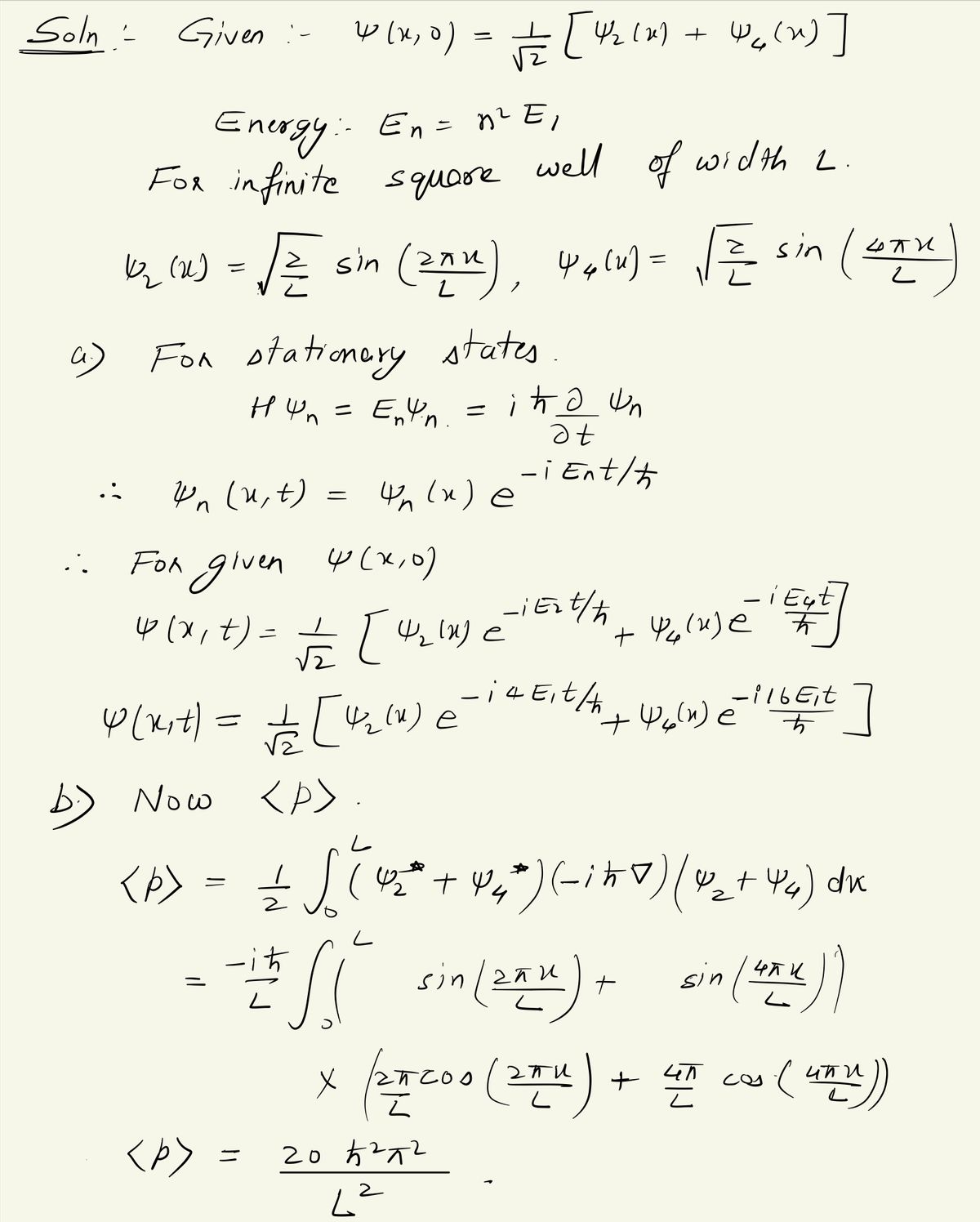
Advanced Physics homework question answer, step 1, image 1

steps

Step by step

Solved in 2 steps with 2 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer