tane =
Trigonometry (11th Edition)
11th Edition
ISBN:9780134217437
Author:Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Chapter1: Trigonometric Functions
Section: Chapter Questions
Problem 1RE:
1. Give the measures of the complement and the supplement of an angle measuring 35°.
Related questions
Question
100%
Hi I need help on question #2 of my homework :)
![**Trigonometric Identities Exploration**
1. \(\text{CSC}(\theta) = -\frac{\sqrt{5}}{2}\)
2. \(\text{CSC}(\theta) = \frac{1}{\sin \theta}\)
\[
\sin \theta = \frac{2}{\sqrt{5}}
\]
3. \(\cos^2 \theta = 1 - \sin^2 \theta = 1 - \left(\frac{2}{\sqrt{5}}\right)^2\)
\[
= 1 - \frac{4}{5} = \frac{5}{5} - \frac{4}{5} = \frac{1}{5}
\]
**Diagram Explanation:**
- A circle is depicted with an angle in the fourth quadrant (labeled as "IV"). Indicators show that cosine (\(\cos\)) and secant (\(\sec\)) are positive in this quadrant.
4. \(\tan \theta = \frac{\sin \theta}{\cos \theta} = \frac{\frac{2}{\sqrt{5}}}{\frac{1}{\sqrt{5}}} = 2\)
\[
\tan \theta = -2 \quad (\text{as indicated in the diagram})
\]
---
2. **Problem to Solve:**
**Find the equivalent of \(\frac{\sec^2 \theta - 1}{\sec 2\theta}\). (Answer should contain \(\sin \theta\))**](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F680f6dd3-4833-4993-8ba2-89ca8a8acd7c%2F0163865f-c330-4972-86ea-e1671ccf2246%2Ftzg3u3g.jpeg&w=3840&q=75)
Transcribed Image Text:**Trigonometric Identities Exploration**
1. \(\text{CSC}(\theta) = -\frac{\sqrt{5}}{2}\)
2. \(\text{CSC}(\theta) = \frac{1}{\sin \theta}\)
\[
\sin \theta = \frac{2}{\sqrt{5}}
\]
3. \(\cos^2 \theta = 1 - \sin^2 \theta = 1 - \left(\frac{2}{\sqrt{5}}\right)^2\)
\[
= 1 - \frac{4}{5} = \frac{5}{5} - \frac{4}{5} = \frac{1}{5}
\]
**Diagram Explanation:**
- A circle is depicted with an angle in the fourth quadrant (labeled as "IV"). Indicators show that cosine (\(\cos\)) and secant (\(\sec\)) are positive in this quadrant.
4. \(\tan \theta = \frac{\sin \theta}{\cos \theta} = \frac{\frac{2}{\sqrt{5}}}{\frac{1}{\sqrt{5}}} = 2\)
\[
\tan \theta = -2 \quad (\text{as indicated in the diagram})
\]
---
2. **Problem to Solve:**
**Find the equivalent of \(\frac{\sec^2 \theta - 1}{\sec 2\theta}\). (Answer should contain \(\sin \theta\))**
Expert Solution
Step 1
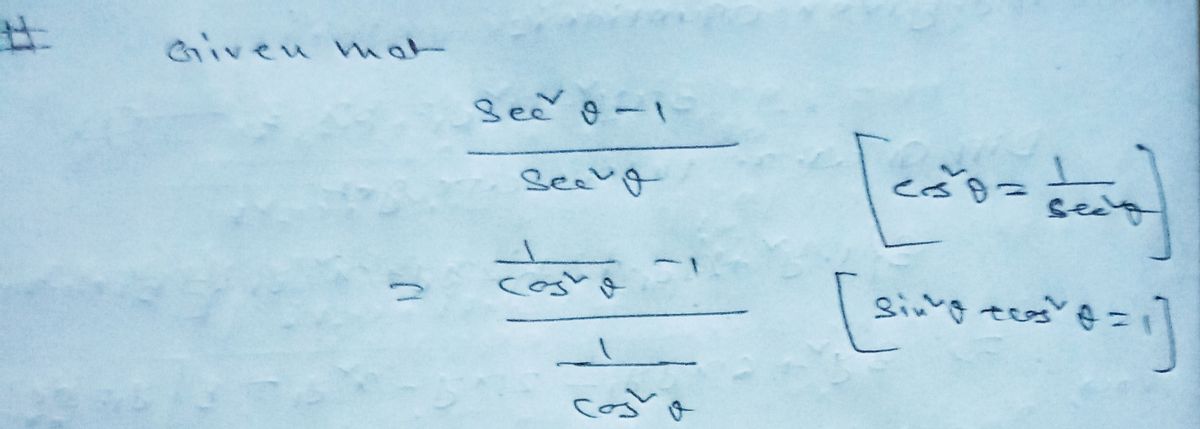
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Trigonometry (11th Edition)
Trigonometry
ISBN:
9780134217437
Author:
Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:
PEARSON

Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781305652224
Author:
Charles P. McKeague, Mark D. Turner
Publisher:
Cengage Learning


Trigonometry (11th Edition)
Trigonometry
ISBN:
9780134217437
Author:
Margaret L. Lial, John Hornsby, David I. Schneider, Callie Daniels
Publisher:
PEARSON

Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781305652224
Author:
Charles P. McKeague, Mark D. Turner
Publisher:
Cengage Learning


Trigonometry (MindTap Course List)
Trigonometry
ISBN:
9781337278461
Author:
Ron Larson
Publisher:
Cengage Learning