Suppose water is leaking from a tank through a circular hole of area A, at its bottom. When water leaks through a hole, friction and contraction of the stream near the hole reduce the volume of water leaving the tank per second to cA√ 2gh, where c (0 < < < 1) is an empirical constant.A tank in the form of a right-circular cone standing on end, vertex down, is leaking water through a circular hole in its bottom. (Assume the removed apex of the cone is of negligible height and volume.)(a) Suppose the tank is 20 feet high and has radius 8 feet and the circular hole has radius 2 inches. The differential equation governing the height h in feet of water leaking from a tank after t seconds is56h3/2-In this model, friction and contraction of the water at the hole are taken into account with c = 0.6, and g is taken to be 32 ft/s². See the figure below.dhdt= -dh==dt8 ftAw-0.6.iIf the tank is initially full, how long will it take the tank to empty? 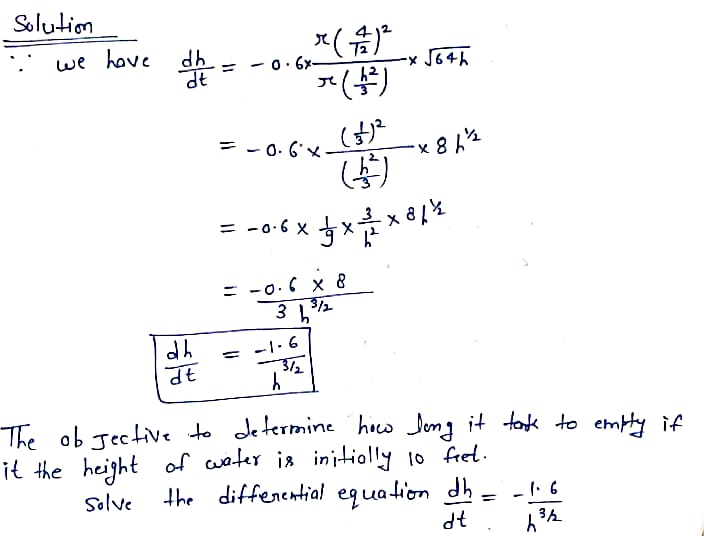(Round your answer to two decimal places.)14.31✓ minutes(b) Suppose the tank has a vertex angle of 60° and the circular hole has radius 4 inches. Determine the differential equation governing the heighth of water. Use c = 0.6 and g = 32 ft/s².421220 ftcircular holeπ1²3-√64hIf the height of the water is initially 10 feet, how long will it take the tank to empty? (Round your answer to two decimal places.)X min

Answered: Image /qna-images/answer/9ed05674-8cad-432a-aba8-75a2534d2809.jpg

Answered: Suppose water is leaking from a tank…

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

A rectangular box expands by the time. Its length is 3 cm more than its width. Its height is 2 times its width. Find the box dimensions at the moment when its volume is increasing at 0.96 cm /min and its width is increasing at 0.02 cm/min. of Select one: ition O a. width = 3 cm, height = 6 cm, length 6 cm O b. width 1 cm, height 2 cm, length 4 cm Oc width 2 cm, height 4 cm, length 5 cm O d. width 4 cm, height = 8 cm, length 7 cm
1. Suppose that the volume V of a certain cone can be expressed as V =nh, where h is the height of the cone. a) By differentiating V = nh with respect to time t, derive an equation that relates h, dh/dt, and dV/dt as the cone shrinks. b) At a certain moment, the height of the cone is decreasing at 2 centimeters per second (cm/sec), and its height is 6 cm. At that same moment, what is the rate of change of the volume of the cone? Do not round your answer. Use the correct unit for your answer. At a certain moment, the height of the cone is 8 centimeters, and its volume is c) decreasing at 160 cm3/sec. At that same moment, what is the rate of change of the height of the cone? Do not round your answer. Use the correct unit for your answer.
Suppose that a drop of mist is a perfect sphere and that, through condensation, the drop picks up moisture at a rate proportional to its surface area. Show that under these circumstances the drop’s radius increases at a constant rate.
Ex. 1. A solid sphere moves through quiescent frictionless liquid whose boundaries are at a distance from it great compared with its radius. Prove that at each instant the motion in the liquid depends only on the position and velocity of the sphere at that instant. Prove that the liquid streams past the sides of the sphere with half the velocity of the sphere.
10.
A particle is moving in a straight line with the velocity v(t) =1– t, 0
(1) A mass weighing 4 lb stretches a spring 2 in. Suppose that the mass us displaced an additional 6 in. and then released. The mass is in a medium that exerts a viscous resistance of 6 lb when the mass has a velocity of 3 ft/sec. Set up the initial value problem that describes the motion of the mass.
Find the maximum rate of change of f at the given point and the direction in which it occurs. f(x, y) = 2 sin(xy), (0, 4) maximum rate of change direction vector
Tank B, a 30-litre tank, initially contains 6 grams of salt in 15 litres of water. At t = 0, saltwater with a concentration of 1 gram of salt per litre is poured from Tank A into Tank B at a rate of 2 litres per minute, while the well mixed solution leaves the bottom of Tank B at a rate of one litre per minute. a) derive the IVP that models the rate of change of the quantity of salt in Tank B with respect to time,b) solve the IVP, andc) determine the quantity and concentration of salt in Tank B at the instant Tank B is full

Recommended textbooks for you

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Mathematics For Machine Technology

Advanced Math

ISBN:

9781337798310

Author:

Peterson, John.

Publisher:

Cengage Learning,

Basic Technical Mathematics

Advanced Math

ISBN:

9780134437705

Author:

Washington

Publisher:

PEARSON

Topology

Advanced Math

ISBN:

9780134689517

Author:

Munkres, James R.

Publisher:

Pearson,

SEE MORE TEXTBOOKS

Suppose water is leaking from a tank through a circular hole of area A, at its bottom. When water leaks through a hole, friction and contraction of the stream near the hole reduce the volume of water leaving the tank per second to cAV 2gh, where c (0