Suppose that T: R → Rm is defined by T(2) A for each of the following matrices below: = D = 0 0 0 -1 0 0 0.5 [40] E = 0 0 02 [201] F = 10 3 01 (a) Rewrite T: R" → R" with correct numbers for m and n for each transformation. What is the domain and codomain of each transformation? (b) Find some way to explain in words and/or graphically what each transformation does as it takes vectors from R to Rm. You might find it t helpful to try out a few input vectors and see what their image is under the transformation. This might be difficult, but an honest effort will give you credit. (c) For the transformation, can you get any output vector? (Any vector in Rm) i. If so, explain why you can get any vector in Rm. ii. If not, give an example of an output vector you can't get with the transformation and explain why.
Suppose that T: R → Rm is defined by T(2) A for each of the following matrices below: = D = 0 0 0 -1 0 0 0.5 [40] E = 0 0 02 [201] F = 10 3 01 (a) Rewrite T: R" → R" with correct numbers for m and n for each transformation. What is the domain and codomain of each transformation? (b) Find some way to explain in words and/or graphically what each transformation does as it takes vectors from R to Rm. You might find it t helpful to try out a few input vectors and see what their image is under the transformation. This might be difficult, but an honest effort will give you credit. (c) For the transformation, can you get any output vector? (Any vector in Rm) i. If so, explain why you can get any vector in Rm. ii. If not, give an example of an output vector you can't get with the transformation and explain why.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![Suppose that T: R" → Rm is defined by T() = A for each of the following matrices below:
[40]
E = 0 0
02
0
D = 0 -1
0
0
0 0.5
[201]
F = 10 3 01
(a) Rewrite T: R" → Rm with correct numbers for m and n for each transformation. What is the domain
and codomain of each transformation?
(b) Find some way to explain in words and/or graphically what each transformation does as it takes vectors
from R to Rm. You might find it t helpful to try out a few input vectors and see what their image is
under the transformation. This might be difficult, but an honest effort will give you credit.
(c) For the transformation, can you get any output vector? (Any vector in R™)
i. If so, explain why you can get any vector in Rm.
ii. If not, give an example of an output vector you can't get with the transformation and explain why.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F992f5037-f1cc-4483-8aa3-731b184958ad%2Fd8ccd4f0-73ee-46e8-85f3-79bc3b7d2433%2F2nx1ay_processed.png&w=3840&q=75)
Transcribed Image Text:Suppose that T: R" → Rm is defined by T() = A for each of the following matrices below:
[40]
E = 0 0
02
0
D = 0 -1
0
0
0 0.5
[201]
F = 10 3 01
(a) Rewrite T: R" → Rm with correct numbers for m and n for each transformation. What is the domain
and codomain of each transformation?
(b) Find some way to explain in words and/or graphically what each transformation does as it takes vectors
from R to Rm. You might find it t helpful to try out a few input vectors and see what their image is
under the transformation. This might be difficult, but an honest effort will give you credit.
(c) For the transformation, can you get any output vector? (Any vector in R™)
i. If so, explain why you can get any vector in Rm.
ii. If not, give an example of an output vector you can't get with the transformation and explain why.
Expert Solution
Step 1: Here
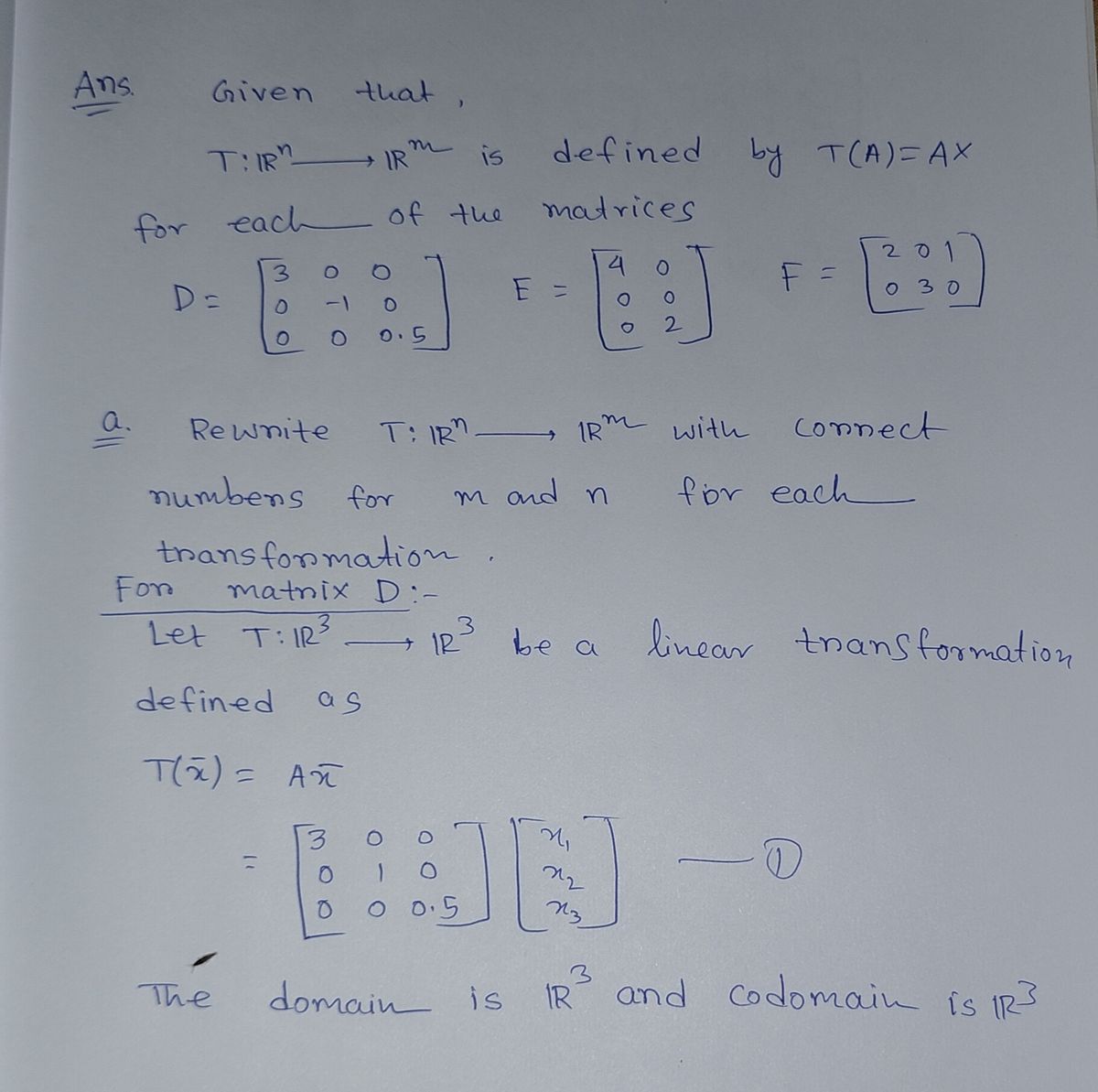
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

