Suppose that a machine performs n independent number of trials in the making of bulbs. Each trial results in a successful bulb production with probability p, or fails with probability q = 1 - p (Note that this is similar to inspecting items independently being produced by a machine for defective (failure) versus non-defective (success) items). What is the probability of having a. Exactly one success in 4 trials? What is this value when p = 0.3? b. At most 5 successes in 10 trials? What is this value when p = 0.1?
Addition Rule of Probability
It simply refers to the likelihood of an event taking place whenever the occurrence of an event is uncertain. The probability of a single event can be calculated by dividing the number of successful trials of that event by the total number of trials.
Expected Value
When a large number of trials are performed for any random variable ‘X’, the predicted result is most likely the mean of all the outcomes for the random variable and it is known as expected value also known as expectation. The expected value, also known as the expectation, is denoted by: E(X).
Probability Distributions
Understanding probability is necessary to know the probability distributions. In statistics, probability is how the uncertainty of an event is measured. This event can be anything. The most common examples include tossing a coin, rolling a die, or choosing a card. Each of these events has multiple possibilities. Every such possibility is measured with the help of probability. To be more precise, the probability is used for calculating the occurrence of events that may or may not happen. Probability does not give sure results. Unless the probability of any event is 1, the different outcomes may or may not happen in real life, regardless of how less or how more their probability is.
Basic Probability
The simple definition of probability it is a chance of the occurrence of an event. It is defined in numerical form and the probability value is between 0 to 1. The probability value 0 indicates that there is no chance of that event occurring and the probability value 1 indicates that the event will occur. Sum of the probability value must be 1. The probability value is never a negative number. If it happens, then recheck the calculation.
Suppose that a machine performs n independent number of trials in the making of bulbs. Each trial results in a successful bulb production with
a. Exactly one success in 4 trials? What is this value when p = 0.3?
b. At most 5 successes in 10 trials? What is this value when p = 0.1?
Binomial distribution:
The binomial distribution gives the probability of number of successes out of n trials in a series of Bernoulli trials.
The probability mass function (pmf) of a binomial random variable X is given as:
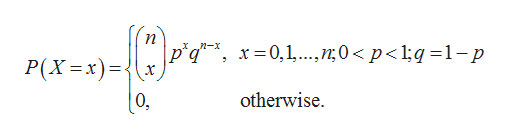
a.Calculating the probability of getting exactly 1 success in 4 trials:
Here, p = 0.30 and n = 4.
The probability of getting exactly 1 success in 4 trials is obtained as follows:
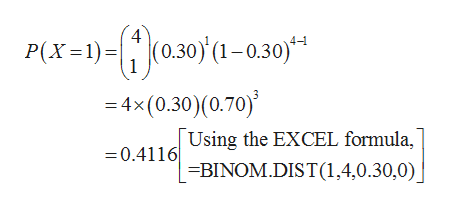
Step by step
Solved in 3 steps with 3 images




