Suppose a bag contains 12 marbles. Of these, 3 are orange and are numbered from 1 to 3. The other 9 marbles are yellow and are numbered from 1 to 9. (a) Suppose you pick one marble from the bag at random. Find the probability that the marble is orange or that it is numbered 2. O 0.027 0.333 0.499 0.603 (b) Suppose you pick two marbles from the bag at random without replacement. Find the probability that both marbles are orange. 0.045 0.251 0.709 0.839 (c) Suppose you pick 4 marbles from the bag at random with replacement. Find the probability that you pick at least one orange marble. 0.436 0.527 0.684 0.707 (d) Suppose you pick one marble from the bag at random. Find the expected value of its number. 0.287 0.797 4.25 7.443
Suppose a bag contains 12 marbles. Of these, 3 are orange and are numbered from 1 to 3. The other 9 marbles are yellow and are numbered from 1 to 9. (a) Suppose you pick one marble from the bag at random. Find the probability that the marble is orange or that it is numbered 2. O 0.027 0.333 0.499 0.603 (b) Suppose you pick two marbles from the bag at random without replacement. Find the probability that both marbles are orange. 0.045 0.251 0.709 0.839 (c) Suppose you pick 4 marbles from the bag at random with replacement. Find the probability that you pick at least one orange marble. 0.436 0.527 0.684 0.707 (d) Suppose you pick one marble from the bag at random. Find the expected value of its number. 0.287 0.797 4.25 7.443
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question

Transcribed Image Text:## Probability Exercise: Marble Selection
### Problem Statement
Suppose a bag contains 12 marbles. Of these, 3 are orange and numbered from 1 to 3. The other 9 marbles are yellow and numbered from 1 to 9.
### Questions
#### (a) Probability of Orange or Number 2
Suppose you pick one marble from the bag at random. Find the probability that the marble is orange or that it is numbered 2.
- ○ 0.027
- ○ 0.333
- ○ 0.499
- ○ 0.603
#### (b) Two Orange Marbles
Suppose you pick two marbles from the bag at random **without replacement**. Find the probability that both marbles are orange.
- ○ 0.045
- ○ 0.251
- ○ 0.709
- ○ 0.839
#### (c) At Least One Orange Marble
Suppose you pick 4 marbles from the bag at random **with replacement**. Find the probability that you pick at least one orange marble.
- ○ 0.436
- ○ 0.527
- ○ 0.684
- ○ 0.707
#### (d) Expected Value of Marble Number
Suppose you pick one marble from the bag at random. Find the expected value of its number.
- ○ 0.287
- ○ 0.797
- ○ 4.25
- ○ 7.443
### Explanation of Concepts
#### Calculating Probability
- The probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes.
#### With and Without Replacement
- **With replacement** means each marble is returned to the bag before the next draw, maintaining the total number of marbles.
- **Without replacement** means each marble is not returned, reducing the total number of marbles for subsequent draws.
#### Expected Value
- The expected value is a weighted average of all possible values, reflecting the average outcome if the experiment is repeated many times.
Expert Solution
Step 1: Mention the given information
Note: Since you have posted a question with multiple sub-parts, we will solve first three subparts for you. To get remaining sub-part solved please repost the complete question and mention the sub-parts to be solved.
Given that a bag contains 12 marbles. Of these, 3 are orange and 9 marbles are yellow.
Let O denotes Orange and Y represents Yellow.
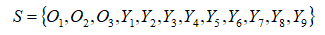
Step by step
Solved in 5 steps with 4 images

Recommended textbooks for you

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc

Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning

Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON

The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman