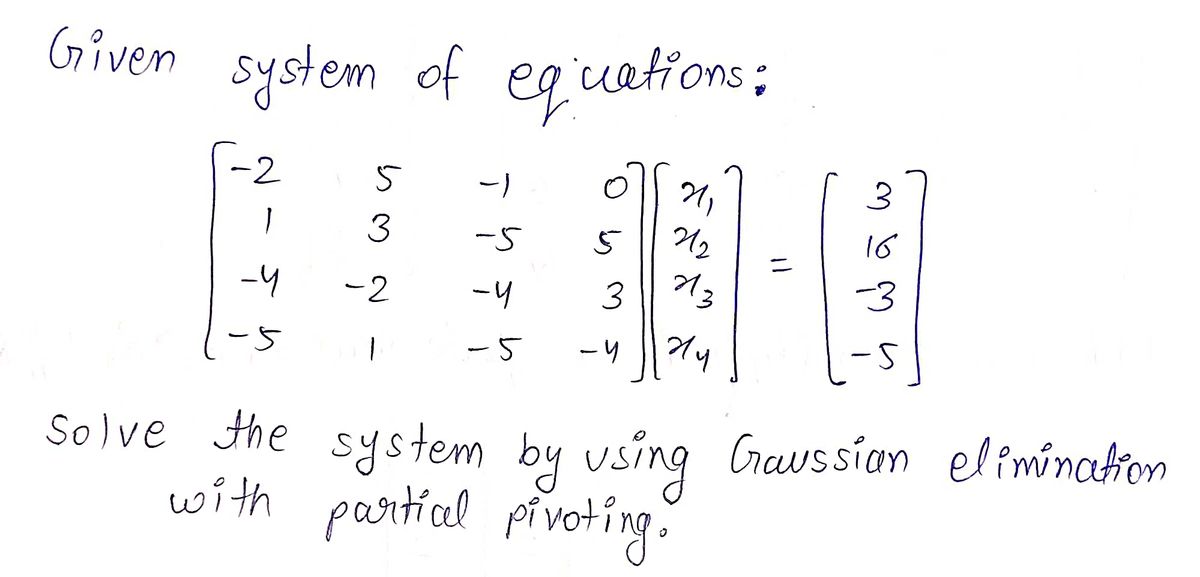
Solve the following system of linear equations, using Gaussian elimination with partial pivoting 3 5 16 18-8 3 -2 5 −1 1 3 -5 -4 -2 -4 L-5 1 -5 your work, explaining each step with words so that your work is easy to follow.
Solve the following system of linear equations, using Gaussian elimination with partial pivoting 3 5 16 18-8 3 -2 5 −1 1 3 -5 -4 -2 -4 L-5 1 -5 your work, explaining each step with words so that your work is easy to follow.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![**Solve the following system of linear equations, using Gaussian elimination with partial pivoting.**
\[
\begin{bmatrix}
-2 & 5 & -1 & 0 \\
1 & 3 & -5 & 5 \\
-4 & -2 & -4 & 3 \\
-5 & 1 & -5 & -4 \\
\end{bmatrix}
\begin{Bmatrix}
x_1 \\
x_2 \\
x_3 \\
x_4 \\
\end{Bmatrix}
=
\begin{Bmatrix}
3 \\
16 \\
-3 \\
-5 \\
\end{Bmatrix}
\]
**Explain your work, detailing each step to ensure clarity.**
**Step 1: Set Up the Augmented Matrix**
The first step in Gaussian elimination is to convert the system of equations to an augmented matrix. This combines the coefficient matrix with the constants from the right side of the equation:
\[
\begin{bmatrix}
-2 & 5 & -1 & 0 & | & 3 \\
1 & 3 & -5 & 5 & | & 16 \\
-4 & -2 & -4 & 3 & | & -3 \\
-5 & 1 & -5 & -4 & | & -5 \\
\end{bmatrix}
\]
**Step 2: Apply Partial Pivoting**
To ensure numerical stability, we use partial pivoting. This involves rearranging the rows so that the largest absolute value in the first column is in the top row.
**Step 3: Gaussian Elimination Process**
1. **Forward Elimination:**
- Use row operations to create zeros below the pivot positions (the leading coefficients of each row).
2. **Backward Substitution:**
- Once the matrix is in row-echelon form, use back-substitution to find the solution to the system.
**Step 4: Iterate and Explain**
1. Swap necessary rows for partial pivoting.
2. Perform row operations: multiply, replace, and add rows to eliminate variables below the pivot.
3. Continue this process until the matrix is in upper triangular form.
4. Use back-substitution to solve for the variables \(x_1, x_2, x_3, \) and \(x_4\).
At each step, clearly explain the row operations and](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F814c1a84-19e4-4bdf-9de9-08c645d411b5%2Fcb265874-5c3f-4526-b7c6-410b98e18568%2Futbnlci_processed.png&w=3840&q=75)
Transcribed Image Text:**Solve the following system of linear equations, using Gaussian elimination with partial pivoting.**
\[
\begin{bmatrix}
-2 & 5 & -1 & 0 \\
1 & 3 & -5 & 5 \\
-4 & -2 & -4 & 3 \\
-5 & 1 & -5 & -4 \\
\end{bmatrix}
\begin{Bmatrix}
x_1 \\
x_2 \\
x_3 \\
x_4 \\
\end{Bmatrix}
=
\begin{Bmatrix}
3 \\
16 \\
-3 \\
-5 \\
\end{Bmatrix}
\]
**Explain your work, detailing each step to ensure clarity.**
**Step 1: Set Up the Augmented Matrix**
The first step in Gaussian elimination is to convert the system of equations to an augmented matrix. This combines the coefficient matrix with the constants from the right side of the equation:
\[
\begin{bmatrix}
-2 & 5 & -1 & 0 & | & 3 \\
1 & 3 & -5 & 5 & | & 16 \\
-4 & -2 & -4 & 3 & | & -3 \\
-5 & 1 & -5 & -4 & | & -5 \\
\end{bmatrix}
\]
**Step 2: Apply Partial Pivoting**
To ensure numerical stability, we use partial pivoting. This involves rearranging the rows so that the largest absolute value in the first column is in the top row.
**Step 3: Gaussian Elimination Process**
1. **Forward Elimination:**
- Use row operations to create zeros below the pivot positions (the leading coefficients of each row).
2. **Backward Substitution:**
- Once the matrix is in row-echelon form, use back-substitution to find the solution to the system.
**Step 4: Iterate and Explain**
1. Swap necessary rows for partial pivoting.
2. Perform row operations: multiply, replace, and add rows to eliminate variables below the pivot.
3. Continue this process until the matrix is in upper triangular form.
4. Use back-substitution to solve for the variables \(x_1, x_2, x_3, \) and \(x_4\).
At each step, clearly explain the row operations and
Expert Solution
Step 1: To Solve:
Step by step
Solved in 3 steps with 4 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

