S 140 120 100 1 2 4 /// ||\\\\\\\ /// ||\\\\\\| /// ||\\\\\\\ | /// ||\\\\\\|
Let S represent sales of a new product (in thousands of units), let L represent the maximum level of sales (in thousands of units), and let t represent time (in months). The rate of change of S with respect to t is proportional to the product of S and L − S.
(a) Write the
Verify that S =L/( 1 + Ce−kt).
(b) At what time is the growth in sales increasing most rapidly?
(c) Use a graphing utility to graph the sales function.
(d) Sketch the solution from part (a) on the slope field below. To print an enlarged copy of the graph, go to MathGraphs.com. Assume the estimated aximum level of sales is correct. Use the slope field to describe the shape of the solution curves for sales when, at some period of time, sales exceed L.

Introduction :
Sales in math deals with rough estimating at times and it gives that big picture view needed to make major business decisions in selling efforts.
Given :
Let represent sales of a new product (in thousands of units), let represent the maximum level of sales (in thousands of units), and let represent time (in months). The rate of change of with respect to is proportional to the product of and .
Objective :
To write the differential equation for the sales model when when , and when .
and verify that .
To determine at what time is the growth in sales increasing most rapidly?
To use a graphing utility to graph the sales function.
To sketch the solution from part on the slope field below.

Differentiating S with respect to t
 .
.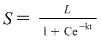 is a solution because
is a solution because Differentiating S with respect to t
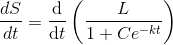
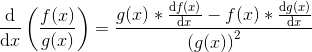
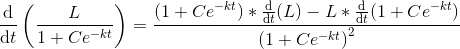
L is constant.
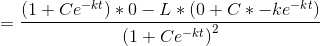
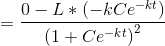
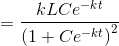
we divide and multiply by L

We split the whole equation into
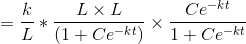
k/L is a constant . Let it be together denoted by a constant k1
Adding 1 and subtracting 1 for simplification
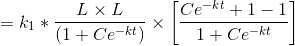

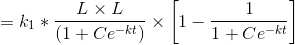
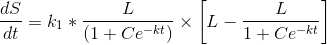
Now for particular solution
L= 100 remains constant
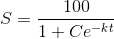
1. When L = 100 , S = 10 , t=0
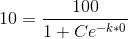
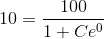
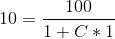
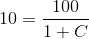
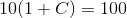
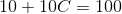
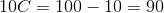

Substituting value of C in main solution , the particular solution is
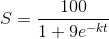
When L = 100 , S = 20 , t=1
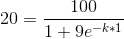
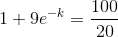
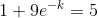
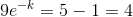
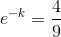
Taking natural logarithm on both sides
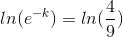
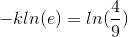
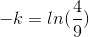
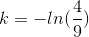
Substituting value in previous equation
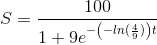
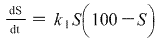
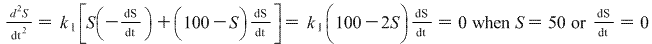 .
.
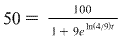
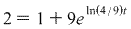
 (This is an inflection point)
(This is an inflection point)
Step by step
Solved in 3 steps with 45 images