Exercise 3. Read Example 3 (p. 739). Explain how the geometric meaning of the cross product helps solve this example.We won’t be covering the last two subsections, but they might be worth taking a look at. The subsection “Torque” (p. 739 – 740) covers a physical application of the cross product. While the last subsection “Triple or Box Product” (p. 740 – 741) goes over a geometric way that the dot product and cross product interact with each other. TorqueComponent of Fperpendicular to r.Its length is | F sin 8.FIGURE 12.33 The torque vectordescribes the tendency of the force F todrive the bolt forward.TorqueWhen we turn a bolt by applying a force F to a wrench (Figure 12.33), we produce atorque that causes the bolt to rotate. The torque vector points in the direction of the axisof the bolt according to the right-hand rule (so the rotation is counterclockwise whenviewed from the tip of the vector). The magnitude of the torque depends on how far out onthe wrench the force is applied and on how much of the force is perpendicular to thewrench at the point of application. The number we use to measure the torque's magnitudeis the product of the length of the lever arm r and the scalar component of F perpendicularto r. In the notation of Figure 12.33,Magnitude of torque vector = |r||F| sin,or r X F. If we let n be a unit vector along the axis of the bolt in the direction of thetorque, then a complete description of the torque vector is r X F, orTorque vector = r X F = (|r||F|sin) n.Recall that we defined u X v to be 0 when u and v are parallel. This is consistent with thetorque interpretation as well. If the force F in Figure 12.33 is parallel to the wrench, mean-ing that we are trying to turn the bolt by pushing or pulling along the line of the wrench'shandle, the torque produced is zero. Chapter 12 Vectors and Geometry of SpaceSolution The vector PÒ X PR is perpendicular to the plane because it is perpendicularto both vectors. 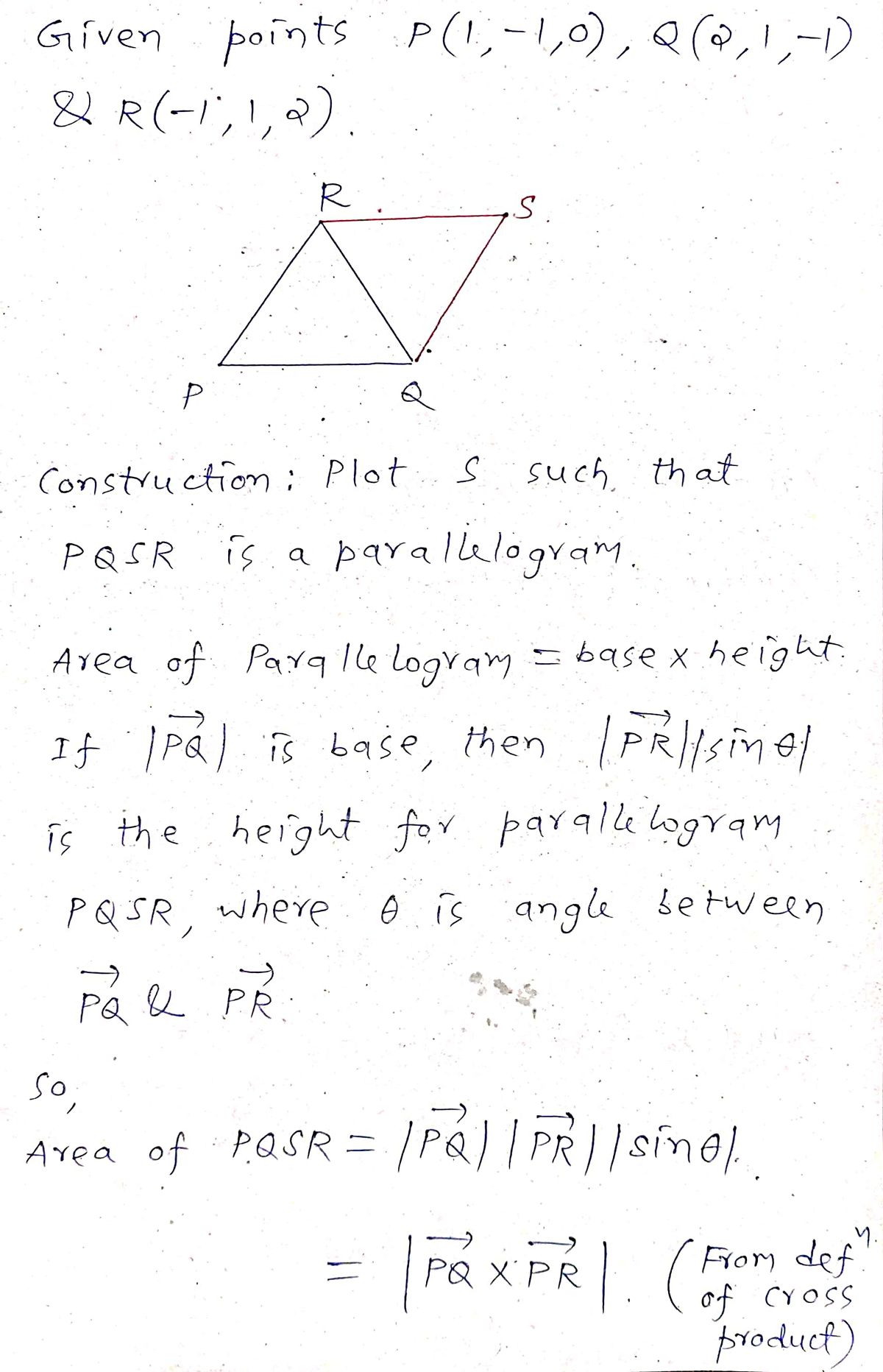In terms of components,PÒ = (2-1)i + (1 + 1)j + (−1 − 0)k = i + 2j - kPR = (1 - 1)i + (1 + 1)j + (2 − 0)k = −2i + 2j + 2kPO X PR =1 2 -1-2 22= 61 + 6k.=12.4 The Cross Product 739Solution The area of the parallelogram determined by P, Q, and R isPÒ X PR| = |6i + 6k-11---12 2+2EXAMPLE 3 Find the area of the triangle with vertices P(1,-1, 0), Q(2, 1,-1), andR(-1, 1, 2) (Figure 12.32).V(6)¹ + (6)² = √2-36-6√/2.The triangle's area is half of this, or 3√₂.n =PÒ X PRPÒ X PR26i + 6k6V/22EXAMPLE 4 Find a unit vector perpendicular to the plane of P(1,-1,0), Q(2, 1,-1),and R(-1, 1, 2).kSolution Since PQX PR is perpendicular to the plane, its direction n is a unit vectorperpendicular to the plane. Taking values from Examples 2 and 3, we haveValues from Example 2= √2/12/²+1/2 kFor ease in calculating the cross product using determinants, we usually write vectorsin the form v = v₁ + ₂ + uşk rather than as ordered triples v = (V₁, V₂, U₂).

Answered: Image /qna-images/answer/e8dcd5a2-a4ad-4c61-a92a-97d1567aa26d.jpg

Answered: Read Example 3 (p. 739). Explain how…

Math

Advanced Math

Read Example 3 (p. 739). Explain how the geometric meaning of the cross product helps solve this example.

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

Question 17 Find the vertex of the Quadratic y =x? –x + The correct answer isn't listed O (, 13 100 ㅇ (-동, ) 5 107 4' 32 O ( ) 5 59 4' 32 (금, 끓) 43 5 100 1 179 20' 880
2.2(2) Please help me answer and explain this question! Determines the Cartesian equation of the plan that is parallel to the plan 2x - 3y + z - 1=0 and passes through the point (-1, -5, -2).
Graph the image of square TUVW after a rotation 90° counterclockwise around the origin. 104 8- 9 -6- -4- 2- -10 -8 -6 -4 -2 2 4 6 8 00 --2- -4- W V -6- T U -8- -10 10 X
Find the parabolically terminated cubic spline through the data points (0,1), (1,1), (2,1), (3,1), (4,1). I am unsure how to figure this out.. or what to do... how do you solve a problem like this.
Derive equations to describe displacement of a cam that has the following characteristics in the dimensional form: a. It declines with a parabolic motion from a dwell to another dwell b. Total lift of the cam is 1 inches. C. Section I of the cam is for 0<0
Question 7 A rancher wants to fence in an area of 1,500,000 square feet in a rectangular field and then divide it in half with a fence down the middle parallel to one side. What is the shortest length of fence that the rancher can use? Submit Question Question 8 Find the point on the line 5x + 3y +3=0 which is closest to the point (-2,-2). Submit Question Jump to Answer Question 9 Jump to Answer Determine two positive values such that the sum of the first number and five times the second number is 425 and whose product is a maximum. Enter the solutions using a comma-separated list. Determine the maximum value.
Help me answer this question ASAP please!
Many smartphones are now able to use information sent out by GPS satellites (of which there are about nine overhead at any one time) in a similar way. Since the satellites are moving around in space, and therefore can’t be located on a two-dimensional map of the Earth, we now need to consider three-dimensional geometry. How many satellites are needed to be sure of the location of a smartphone? Explain your findings