Homework Help is Here – Start Your Trial Now!
Math
Advanced Math
Prove that 2" < n! for all positive integers n such that n > 4.

Prove that 2" < n! for all positive integers n such that n > 4.

Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
See similar textbooks
icon
Related questions
Question

need this asap please show all the work and write out the prove step by step pleaseee

 

**Mathematical Induction Problem:** **Prove that** \[ 2^n < n! \] **for all positive integers \( n \) such that \( n \geq 4 \).** ### Explanation: This mathematical statement asserts that for any integer \( n \) that is 4 or greater, the value of \( 2^n \) (two raised to the power of \( n \)) is less than \( n! \) (the factorial of \( n \)). The proof typically involves using mathematical induction, a method of mathematical proof typically used to establish a given statement for all natural numbers. ### Steps of Proof: 1. **Base Case:** Verify the inequality for the initial value \( n = 4 \). 2. **Inductive Step:** - Assume the statement holds for some integer \( k \geq 4 \). That is, assume \( 2^k < k! \). - Prove that the inequality also holds for \( k+1 \), i.e., \( 2^{k+1} < (k+1)! \). ### Inductive Process: To complete the proof, each of these steps would need to be verified. The base case typically requires plugging the initial value into both sides of the inequality. The inductive step involves algebraic manipulation and logical reasoning to show the inequality holds for \( k+1 \) based on the assumption that it holds for \( k \).
Transcribed Image Text:**Mathematical Induction Problem:** **Prove that** \[ 2^n < n! \] **for all positive integers \( n \) such that \( n \geq 4 \).** ### Explanation: This mathematical statement asserts that for any integer \( n \) that is 4 or greater, the value of \( 2^n \) (two raised to the power of \( n \)) is less than \( n! \) (the factorial of \( n \)). The proof typically involves using mathematical induction, a method of mathematical proof typically used to establish a given statement for all natural numbers. ### Steps of Proof: 1. **Base Case:** Verify the inequality for the initial value \( n = 4 \). 2. **Inductive Step:** - Assume the statement holds for some integer \( k \geq 4 \). That is, assume \( 2^k < k! \). - Prove that the inequality also holds for \( k+1 \), i.e., \( 2^{k+1} < (k+1)! \). ### Inductive Process: To complete the proof, each of these steps would need to be verified. The base case typically requires plugging the initial value into both sides of the inequality. The inductive step involves algebraic manipulation and logical reasoning to show the inequality holds for \( k+1 \) based on the assumption that it holds for \( k \).
Expert Solution
Step 1

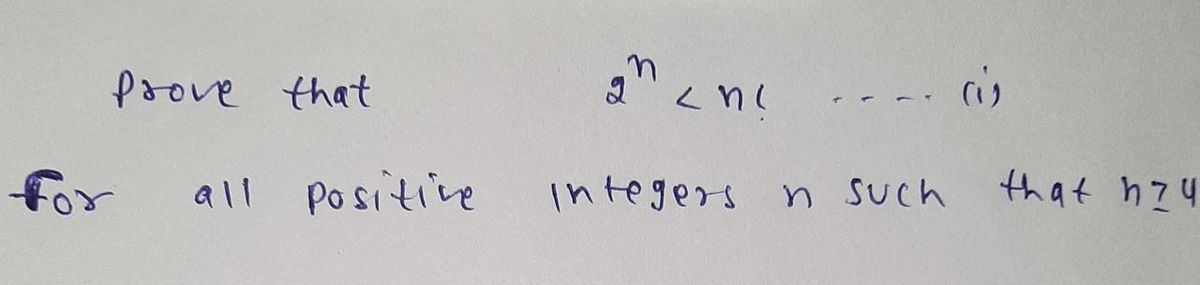
Advanced Math homework question answer, step 1, image 1

steps

Step by step

Solved in 3 steps with 3 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
Basic Technical Mathematics
Basic Technical Mathematics
Advanced Math
ISBN:
9780134437705
Author:
Washington
Publisher:
PEARSON
Topology
Topology
Advanced Math
ISBN:
9780134689517
Author:
Munkres, James R.
Publisher:
Pearson,
SEE MORE TEXTBOOKS