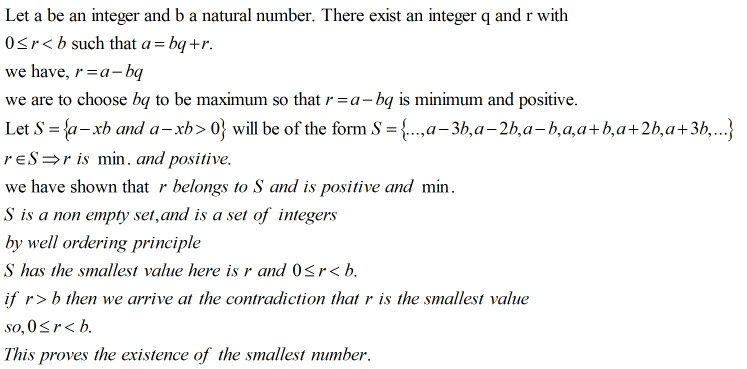
Proposition 0.1. Let a be an integer and b a natural number. There is an integers q and r with 0
Proposition 0.1. Let a be an integer and b a natural number. There is an integers q and r with 0
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question

Transcribed Image Text:Proposition 0.1. Let a be an integer and b a natural number. There is an integers q and
r with 0 <r < b such that:
a = bq +r
Here q is called the quotient and r is called the remainder. Here's a hint: think about
all possible q and r 2 0 that make the equation hold (without the assumption r < b) and
then use well ordering to find the smallest such r.
This proposition is often called the division algorithm because it is tells you exactly what
one gets from old fashioned long division of natural numbers - a quotient and a remainder.
However there's a subtlety here - the proposition says that q and r exist, but not that they
are unique - in other words the division problem might have more than one right answer.
Obviously that's not what we expect. Here's how that is phrased precisely (make sure you
understand why, then prove it):
Proposition 0.2. Let b a natural number and q1, q2, r1, r2 integers with 0 < ri < b and
0 < r2 < b such that qib+ r1 = q2b+ r2 then qi = q2 and ri = r2.
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

