Elementary Geometry For College Students, 7e
7th Edition
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Alexander, Daniel C.; Koeberlein, Geralyn M.
ChapterP: Preliminary Concepts
SectionP.CT: Test
Problem 1CT
Related questions
Question
How to solve this question step by step

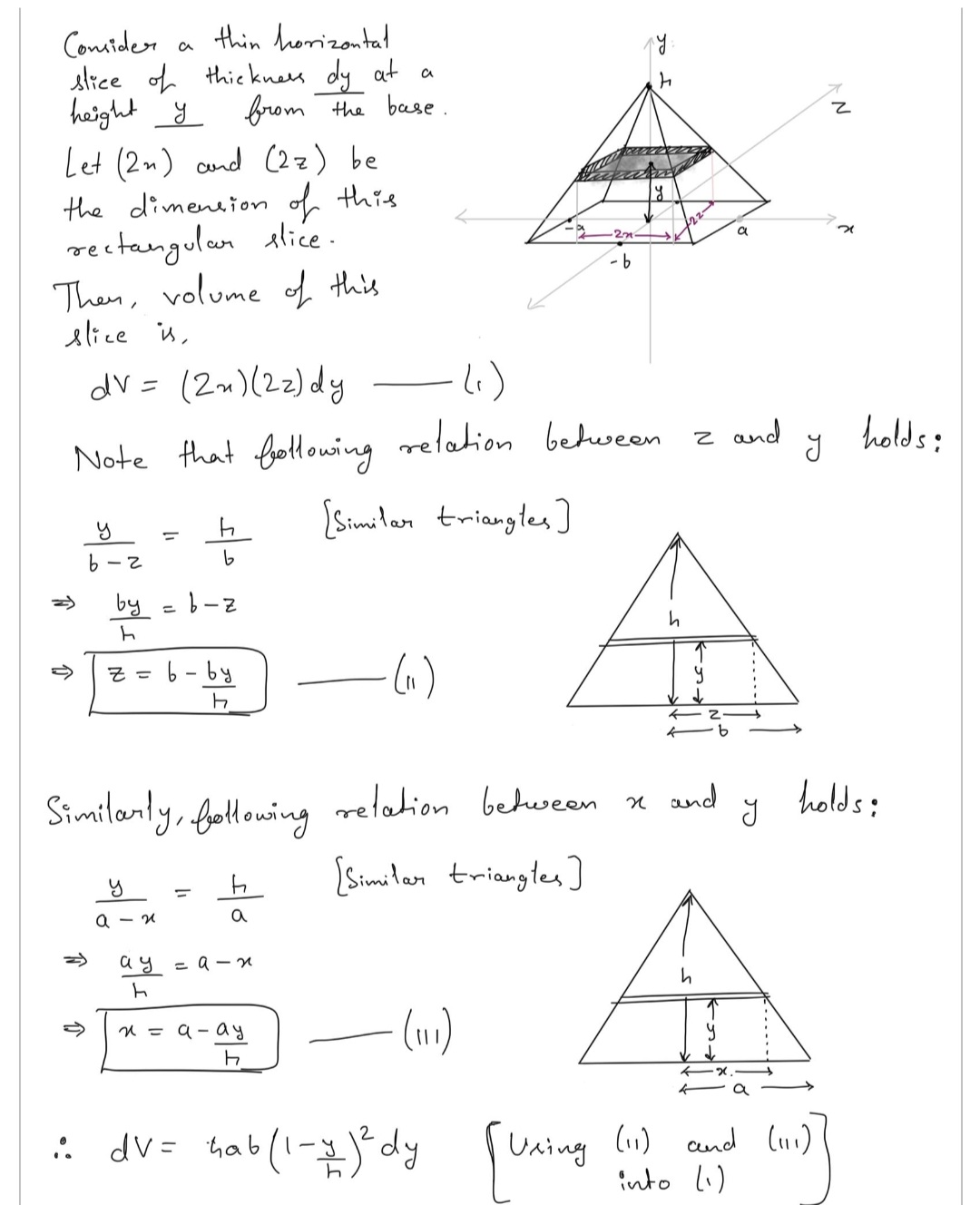
Transcribed Image Text:**Problem 7.9** - Locate the centroid of the pyramid shown by integration.
In the diagram, a pyramid is presented within a 3D coordinate system. The pyramid has a square base and triangular faces converging at an apex. The coordinates are represented by axes labeled \(x\), \(y\), and \(z\).
Key elements:
- The base of the pyramid is shown on the \(xz\)-plane, parallel to the \(x\) and \(z\) axes.
- Dimensions of the base are labeled as \(2a\) along the \(z\)-axis and \(2b\) along the \(x\)-axis.
- The height of the pyramid, from the base to the apex along the \(y\)-axis, is labeled \(h\).
- The shape has been divided into smaller sections to possibly indicate integration steps for finding the centroid.
This problem involves calculating the centroid using integration, which involves dividing the pyramid into elemental volumes, calculating the coordinates for each elemental volume, and then integrating these coordinates over the entire volume of the pyramid.
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,

Elementary Geometry for College Students
Geometry
ISBN:
9781285195698
Author:
Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:
Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,

Elementary Geometry for College Students
Geometry
ISBN:
9781285195698
Author:
Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:
Cengage Learning