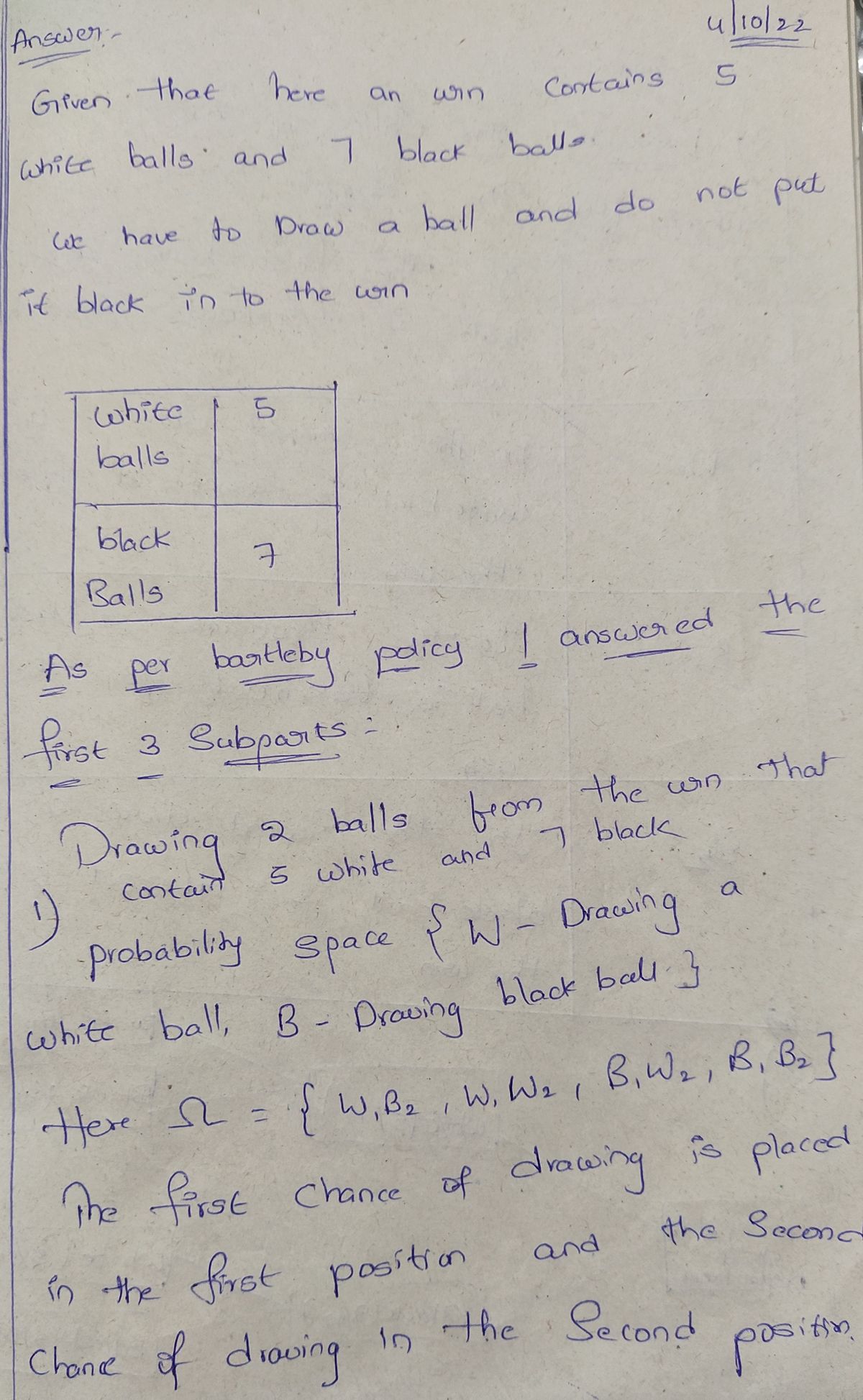
Problem 2 An urn contains 5 white balls and 7 black balls. Draw a ball and do not put it back into the urn. Then draw a second ball. (1) Define an appropriate probability space (n,A,P) to describe the experiment. (2) Describe the following events as subsets of the sample space : W₁ = "The first ball is white" B₁: "The first ball is black" W₂: "The second ball is white" B₂: "The second ball is black" (3) Compute P(W₂ | W₁), P(W₂ | B₁), and P(W₂). (4) Are W₁ and W₂ independent? Justify your claim mathematically!
Problem 2 An urn contains 5 white balls and 7 black balls. Draw a ball and do not put it back into the urn. Then draw a second ball. (1) Define an appropriate probability space (n,A,P) to describe the experiment. (2) Describe the following events as subsets of the sample space : W₁ = "The first ball is white" B₁: "The first ball is black" W₂: "The second ball is white" B₂: "The second ball is black" (3) Compute P(W₂ | W₁), P(W₂ | B₁), and P(W₂). (4) Are W₁ and W₂ independent? Justify your claim mathematically!
Related questions
Question
Please write a formal mathematical definition for the entire probability space not just omega

Transcribed Image Text:Problem 2
An urn contains 5 white balls and 7 black balls. Draw a
ball and do not put it back into the urn. Then draw a second ball.
(1) Define an appropriate probability space (2,A,P) to describe the experiment.
(2) Describe the following events as subsets of the sample space :
W₁ = "The first ball is white"
B₁: "The first ball is black"
W₂: "The second ball is white"
B₂: "The second ball is black"
(3) Compute P(W₂ | W1₁), P(W₂ | B₁), and P(W₂).
(4) Are W₁ and W₂ independent? Justify your claim mathematically!
Expert Solution
Step 1
Step by step
Solved in 3 steps with 3 images
