Problem 1. Water boils inside of a small pipe, at a temperature T₁. Heat is generated at a rate of R(in units of W/m³) throughout the pipe material. The outside temperature is T. and there is a naturalconvection heat transfer coefficient, h around the outer wall. (r= inside diameter, ro= outsidediameter)(a) Derive an expression for the dimensionless temperature distribution, O=(T-T)/(Ti-T.), asa function of the dimensionless radius, Ç=r/r。 and C=r;/ro; a heat generation number,T=Rr.²/k(Ti-Too); and the Biot number.(b) Plot this result for the case 2/3, Bi=1, and for several values of T. And discuss theresults.roRh

Answered: Problem 1. Water boils inside of a…

Introduction to Chemical Engineering Thermodynamics

8th Edition

ISBN:9781259696527

Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart

Publisher:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart

Chapter1: Introduction

Section: Chapter Questions

Problem 1.1P

See similar textbooks

Related questions

Question

Problem 1. Water boils inside of a small pipe, at a temperature T₁. Heat is generated at a rate of R
(in units of W/m³) throughout the pipe material. The outside temperature is T. and there is a natural
convection heat transfer coefficient, h around the outer wall. (r= inside diameter, ro= outside
diameter)
(a) Derive an expression for the dimensionless temperature distribution, O=(T-T)/(Ti-T.), as
a function of the dimensionless radius, Ç=r/r。 and C=r;/ro; a heat generation number,
T=Rr.²/k(Ti-Too); and the Biot number.
(b) Plot this result for the case 2/3, Bi=1, and for several values of T. And discuss the
results.
ro
R
h

Expert Solution

Step 1

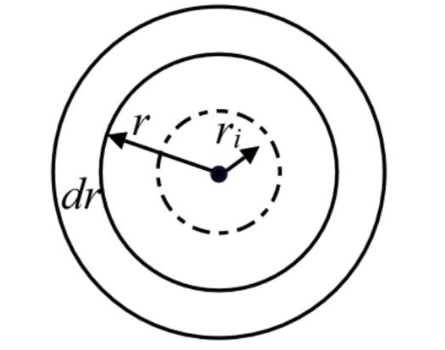
From energy balance at distance r from the centre

$q_{g e n r a t e d} = q_{c o n d u c t e d} q A_{g} L = - K A \frac{d T}{d r} a r e a o f c i r c l e = π (r^{2} - {r_{0}}^{2}) area of cylinder = 2 πrL hence q π (r^{2} - {r_{0}}^{2}) = - K 2 πrL \frac{dT}{dr}$

therefore

$\frac{d T}{d r} = - (\frac{q r}{2 k} - \frac{q {r_{o}}^{2}}{2 k r})$

Now by integrating above equation we will get

$T (r) = - (\frac{q}{4 k} r^{2} - \frac{q {r^{2}}_{0}}{2 k} \ln r + c) n o w a p p l y b o u n d a r y c o n d i t i o n a t r = r_{o}, T = T_{o} h e n c e c = - T_{o} - \frac{q}{4 k} {r_{o}}^{2} + \frac{q {r^{2}}_{0}}{2 k} \ln r_{o} h e n c e T (r) = - (\frac{q}{4 k} r^{2} - \frac{q {r^{2}}_{0}}{2 k} \ln r - T_{o} - \frac{q}{4 k} {r_{o}}^{2} + \frac{q {r^{2}}_{0}}{2 k} \ln r_{o})$