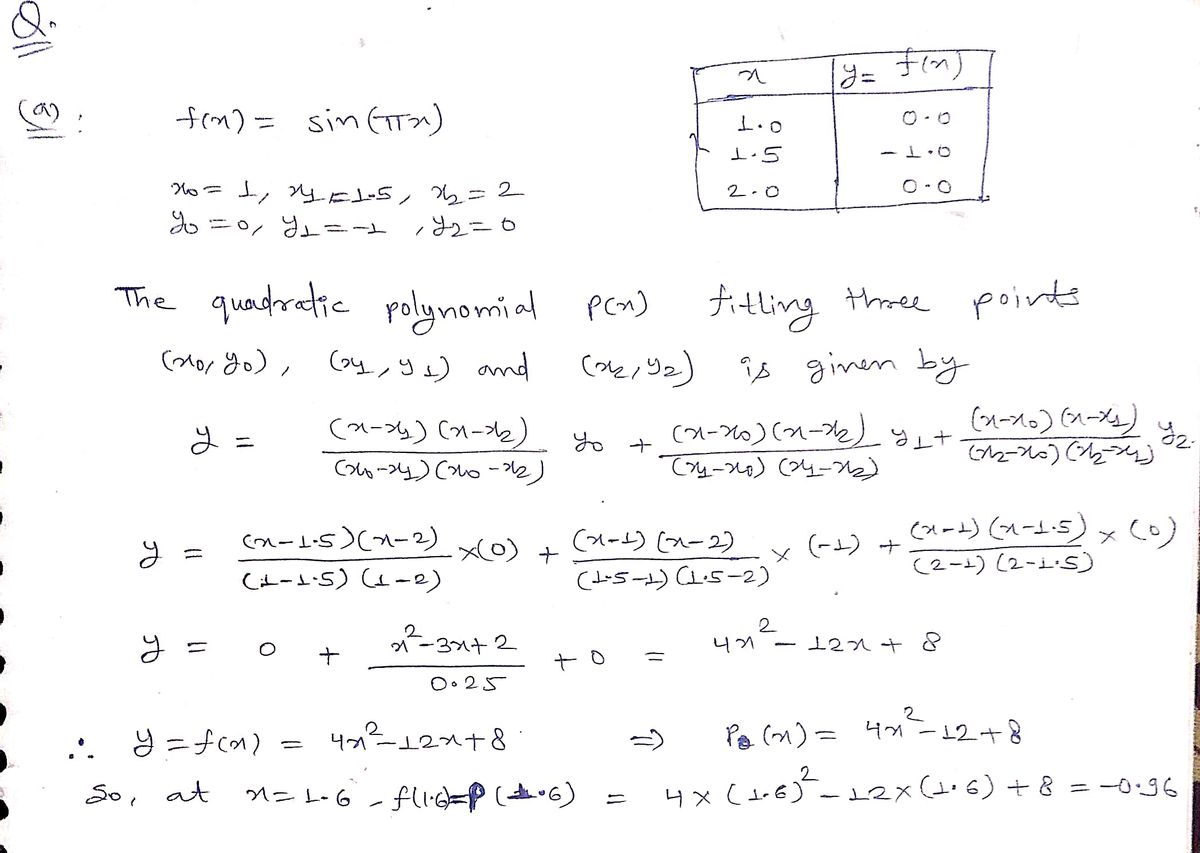
Pk) = )(K- (-) = a. A table of values for f(r) = sin(xx) is: 1. * f(x) 1.0 0.0 1.5 -1.0 2.0 0.0 Use quadratic interpolation to estimate f(1.6). Rk) = (K-2) 4x²_12x +8 (15-1) (15-2) (16) = 0.6 (-0,4) ) = (-0 6.5 (-05) '(4)=(-0,96 b. Use the Lagrange error formula to obtain a reasonable bound on the error in your estimate pa(1.6) of f(1.6). -t' car (1€) 3! 0,124 c. Calculate the exact error f(1.6) - Pa(1.6).
Pk) = )(K- (-) = a. A table of values for f(r) = sin(xx) is: 1. * f(x) 1.0 0.0 1.5 -1.0 2.0 0.0 Use quadratic interpolation to estimate f(1.6). Rk) = (K-2) 4x²_12x +8 (15-1) (15-2) (16) = 0.6 (-0,4) ) = (-0 6.5 (-05) '(4)=(-0,96 b. Use the Lagrange error formula to obtain a reasonable bound on the error in your estimate pa(1.6) of f(1.6). -t' car (1€) 3! 0,124 c. Calculate the exact error f(1.6) - Pa(1.6).
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Answer is given BUT need full detailed steps and process since I don't understand the concept.
![### Interpolation and Error Estimation for \( f(x) = \sin(\pi x) \)
#### 1. Table of Values
For the function \( f(x) = \sin(\pi x) \), the given data points are:
\[
\begin{array}{c|c}
x & f(x) \\
\hline
0 & 0 \\
1.0 & 0.0 \\
1.5 & -1.0 \\
2.0 & 0.0 \\
\end{array}
\]
#### a. Quadratic Interpolation
To estimate \( f(1.6) \), use the quadratic interpolation polynomial:
\[
p_2(x) = \frac{(x-1)(x-2)}{(1.5-1)(1.5-2)}(-1) = 4x^2 - 12x + 8
\]
Calculating for \( x = 1.6 \):
\[
p_2(1.6) = 0.6 \cdot (-0.6) \cdot (1) = -0.96
\]
#### b. Error Estimation Using Lagrange Formula
To find a reasonable error bound in the estimate \( p_2(1.6) \), use the Lagrange error formula:
\[
f(x) - p_2(x) = \frac{f^{(3)}(\xi)}{3!}(x-1)(x-1.5)(x-2)
\]
Estimating the bound:
\[
\left| \frac{-\pi^3 \cos(\pi \xi)}{6} \right|\leq \frac{\pi^3}{6} (0.024) \approx 0.124
\]
Here, \( 1 < \xi < 2 \).
#### c. Exact Error Calculation
Calculate \( f(1.6) \) using \( f(1.6) = \sin(1.6\pi) \):
\[
f(1.6) = -0.951
\]
The exact error is:
\[
f(1.6) - p_2(1.6) = -0.951 - (-0.96) = 0.009
\]
### Summary
A quadratic interpolation was used to estimate \( f](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fcb460c0c-d029-4e90-a450-1d82490780a1%2F14c69d43-db38-4586-bf3e-dbf760bcf2ec%2F07ejkp3_processed.png&w=3840&q=75)
Transcribed Image Text:### Interpolation and Error Estimation for \( f(x) = \sin(\pi x) \)
#### 1. Table of Values
For the function \( f(x) = \sin(\pi x) \), the given data points are:
\[
\begin{array}{c|c}
x & f(x) \\
\hline
0 & 0 \\
1.0 & 0.0 \\
1.5 & -1.0 \\
2.0 & 0.0 \\
\end{array}
\]
#### a. Quadratic Interpolation
To estimate \( f(1.6) \), use the quadratic interpolation polynomial:
\[
p_2(x) = \frac{(x-1)(x-2)}{(1.5-1)(1.5-2)}(-1) = 4x^2 - 12x + 8
\]
Calculating for \( x = 1.6 \):
\[
p_2(1.6) = 0.6 \cdot (-0.6) \cdot (1) = -0.96
\]
#### b. Error Estimation Using Lagrange Formula
To find a reasonable error bound in the estimate \( p_2(1.6) \), use the Lagrange error formula:
\[
f(x) - p_2(x) = \frac{f^{(3)}(\xi)}{3!}(x-1)(x-1.5)(x-2)
\]
Estimating the bound:
\[
\left| \frac{-\pi^3 \cos(\pi \xi)}{6} \right|\leq \frac{\pi^3}{6} (0.024) \approx 0.124
\]
Here, \( 1 < \xi < 2 \).
#### c. Exact Error Calculation
Calculate \( f(1.6) \) using \( f(1.6) = \sin(1.6\pi) \):
\[
f(1.6) = -0.951
\]
The exact error is:
\[
f(1.6) - p_2(1.6) = -0.951 - (-0.96) = 0.009
\]
### Summary
A quadratic interpolation was used to estimate \( f
Expert Solution
Step 1
Step by step
Solved in 3 steps with 3 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

