Over the past year, the average interest rate for so-called jumbo loans-$523,750 and up in the Boston area-has fallen from 6 percent to about 5 percent for a 30- year, fixed-rate mortgage. That translates into a monthly savings of about $375 on a $600,000 loan. [R223] (a) What monthly payment will retire the loan when the interest rate is 6%? (b) What monthly payment will retire the loan when the interest rate is 5%? (c) Is the newspaper's claim of a $375 monthly saving correct?
Over the past year, the average interest rate for so-called jumbo loans-$523,750 and up in the Boston area-has fallen from 6 percent to about 5 percent for a 30- year, fixed-rate mortgage. That translates into a monthly savings of about $375 on a $600,000 loan. [R223] (a) What monthly payment will retire the loan when the interest rate is 6%? (b) What monthly payment will retire the loan when the interest rate is 5%? (c) Is the newspaper's claim of a $375 monthly saving correct?
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![**Educational Content: Understanding Jumbo Loan Interest Rate Savings**
Over the past year, the average interest rate for so-called jumbo loans—$523,750 and up in the Boston area—has fallen from 6 percent to about 5 percent for a 30-year, fixed-rate mortgage. This translates into a monthly savings of about $375 on a $600,000 loan. [R223]
(a) What monthly payment will retire the loan when the interest rate is 6%?
(b) What monthly payment will retire the loan when the interest rate is 5%?
(c) Is the newspaper’s claim of a $375 monthly saving correct?
---
**Analysis and Explanation**
This text highlights the impact of changing interest rates on monthly mortgage payments for jumbo loans. Jumbo loans are substantial home loans that exceed the standard limits set by government-backed entities.
- **Interest Rate Decline**: The interest rate for these loans has decreased by 1%, from 6% to 5%, leading to significant monthly savings.
- **30-Year Fixed-Rate Mortgage**: This type of mortgage means the interest rate remains the same throughout the 30-year term, providing predictability for budgeting.
- **Monthly Savings**: The text suggests the change in interest rate results in a $375 monthly saving on a $600,000 loan, prompting questions on the accuracy of this claim.
**Calculations**
To verify this claim, one would calculate the monthly payments for both interest rates using the formula for monthly mortgage payments:
\[ M = P \frac{r(1+r)^n}{(1+r)^n - 1} \]
Where:
- \( M \) is the monthly payment.
- \( P \) is the principal loan amount ($600,000 in this case).
- \( r \) is the monthly interest rate (annual rate divided by 12).
- \( n \) is the number of payments (30 years × 12 months).
These calculations will determine if the actual savings match the claimed $375 per month. Such exercises help enhance financial literacy, emphasizing how even small changes in interest rates can greatly affect overall costs.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fbc8d5fe8-c51e-4914-8d45-6bcdd10da2fd%2Ff52ec93a-054b-47a9-b3ea-6df38364c086%2F15do0ds_processed.jpeg&w=3840&q=75)
Transcribed Image Text:**Educational Content: Understanding Jumbo Loan Interest Rate Savings**
Over the past year, the average interest rate for so-called jumbo loans—$523,750 and up in the Boston area—has fallen from 6 percent to about 5 percent for a 30-year, fixed-rate mortgage. This translates into a monthly savings of about $375 on a $600,000 loan. [R223]
(a) What monthly payment will retire the loan when the interest rate is 6%?
(b) What monthly payment will retire the loan when the interest rate is 5%?
(c) Is the newspaper’s claim of a $375 monthly saving correct?
---
**Analysis and Explanation**
This text highlights the impact of changing interest rates on monthly mortgage payments for jumbo loans. Jumbo loans are substantial home loans that exceed the standard limits set by government-backed entities.
- **Interest Rate Decline**: The interest rate for these loans has decreased by 1%, from 6% to 5%, leading to significant monthly savings.
- **30-Year Fixed-Rate Mortgage**: This type of mortgage means the interest rate remains the same throughout the 30-year term, providing predictability for budgeting.
- **Monthly Savings**: The text suggests the change in interest rate results in a $375 monthly saving on a $600,000 loan, prompting questions on the accuracy of this claim.
**Calculations**
To verify this claim, one would calculate the monthly payments for both interest rates using the formula for monthly mortgage payments:
\[ M = P \frac{r(1+r)^n}{(1+r)^n - 1} \]
Where:
- \( M \) is the monthly payment.
- \( P \) is the principal loan amount ($600,000 in this case).
- \( r \) is the monthly interest rate (annual rate divided by 12).
- \( n \) is the number of payments (30 years × 12 months).
These calculations will determine if the actual savings match the claimed $375 per month. Such exercises help enhance financial literacy, emphasizing how even small changes in interest rates can greatly affect overall costs.
Expert Solution
Step 1
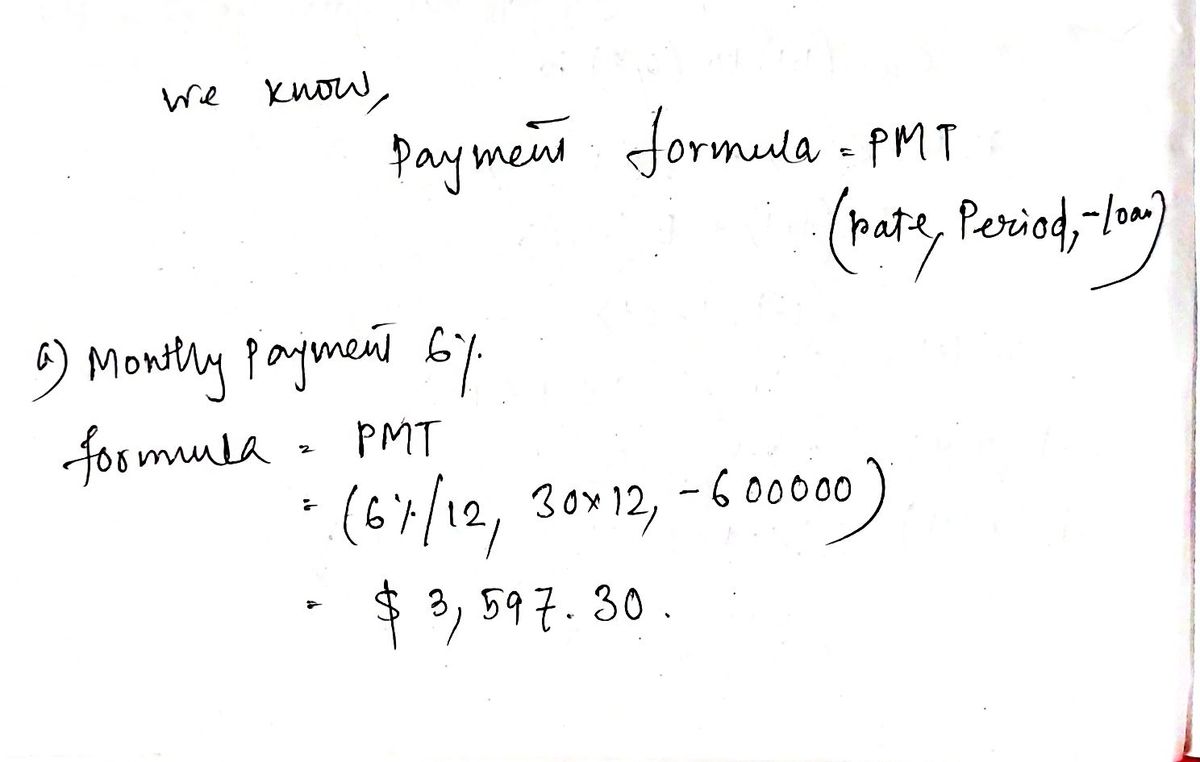
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

