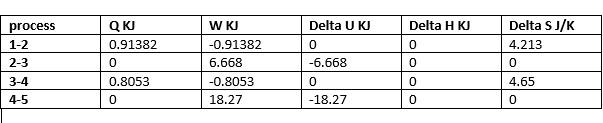
ndergoes isothermal expansion to 5 L followed by adiabatic expansion to 7 L. The gas is then sothermally compressed to back to 4 L and adiabatically compressed back to 2 L. b. Complete the table below Q (kl) W (kJ) Δυ (kJ) AH (kJ) AS (J/K) Process 10 2 2 0 3 3 0 4 4 0 1 C. Calculate the maximum efficiency for this cycle.
ndergoes isothermal expansion to 5 L followed by adiabatic expansion to 7 L. The gas is then sothermally compressed to back to 4 L and adiabatically compressed back to 2 L. b. Complete the table below Q (kl) W (kJ) Δυ (kJ) AH (kJ) AS (J/K) Process 10 2 2 0 3 3 0 4 4 0 1 C. Calculate the maximum efficiency for this cycle.
Introduction to Chemical Engineering Thermodynamics
8th Edition
ISBN:9781259696527
Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart
Publisher:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart
Chapter1: Introduction
Section: Chapter Questions
Problem 1.1P
Related questions
Question
One mole of a monatomic ideal gas
the finished graph is the graph from part a

Transcribed Image Text:State
P (atm)
V (L)
т (к)
1
216.87
2
3.6
216.87
2.05
173.09
4
3.59
4
173.09
11.42
2
275.4
357

Transcribed Image Text:1. One mole of a monatomic ideal gas is held initially at a pressure of 6 atm and 3 L. The gas
undergoes isothermal expansion to 5 L followed by adiabatic expansion to 7 L. The gas is then
isothermally compressed to back to 4 L and adiabatically compressed back to 2 L.
b. Complete the table below
Process
Q (kJ)
w (kJ)
Δυ (kJ)
AH (kJ)
AS (J/K)
10 2
2 0 3
3 0 4
4 0 1
C.
Calculate the maximum efficiency for this cycle.
Expert Solution
Step 1
Step 2
Step by step
Solved in 3 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, chemical-engineering and related others by exploring similar questions and additional content below.Recommended textbooks for you

Introduction to Chemical Engineering Thermodynami…
Chemical Engineering
ISBN:
9781259696527
Author:
J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart
Publisher:
McGraw-Hill Education

Elementary Principles of Chemical Processes, Bind…
Chemical Engineering
ISBN:
9781118431221
Author:
Richard M. Felder, Ronald W. Rousseau, Lisa G. Bullard
Publisher:
WILEY

Elements of Chemical Reaction Engineering (5th Ed…
Chemical Engineering
ISBN:
9780133887518
Author:
H. Scott Fogler
Publisher:
Prentice Hall

Introduction to Chemical Engineering Thermodynami…
Chemical Engineering
ISBN:
9781259696527
Author:
J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart
Publisher:
McGraw-Hill Education

Elementary Principles of Chemical Processes, Bind…
Chemical Engineering
ISBN:
9781118431221
Author:
Richard M. Felder, Ronald W. Rousseau, Lisa G. Bullard
Publisher:
WILEY

Elements of Chemical Reaction Engineering (5th Ed…
Chemical Engineering
ISBN:
9780133887518
Author:
H. Scott Fogler
Publisher:
Prentice Hall


Industrial Plastics: Theory and Applications
Chemical Engineering
ISBN:
9781285061238
Author:
Lokensgard, Erik
Publisher:
Delmar Cengage Learning

Unit Operations of Chemical Engineering
Chemical Engineering
ISBN:
9780072848236
Author:
Warren McCabe, Julian C. Smith, Peter Harriott
Publisher:
McGraw-Hill Companies, The