Item 6 Learning Goal: To understand standing waves, including calculation of A and f, and to learn the physical meaning behind some musical terms. The columns in the figure (Eigure 1) show the instantaneous shape of a vibrating guitar string drawn every 1 ms. The guitar string is 60 cm long. The left column shows the guitar string shape as a sinusoidal traveling wave passes through it. Notice that the shape is sinusoidal at all times and specific features, such as the crest indicated with the arrow, travel along the string to the right at a constant speed. The right column shows snapshots of the sinusoidal standing wave formed when this sinusoidal traveling wave passes through an identically shaped wave moving in the opposite direction on the same guitar string. The string is momentarily flat when the underlying traveling waves are exactly out of phase. The shape is sinusoidal with twice the original amplitude when the underlying waves are momentarily in phase. This pattern is called a standing wave because no wave features travel down the length of the string. Figure Time Traveling Wave 0 ms 1 ms 2 ms 3 ms I x= 0cm 118/8 Standing Wave II x= x= 60 cm 0 cm 60 cm 1 of 3 > ▼ Standing waves on a guitar string form when waves traveling down the string reflect off a point where the string is tied down or pressed against the fingerboard. The entire series of distortions may be superimposed on a single figure, like this (Eigure 2), indicating different moments in time using traces of different colors or line styles. Part A What is the wavelength of the standing wave shown on the guitar string? Express your answer in centimeters. ▸ View Available Hint(s) A = 40 cm V Previous Answers Correct Nodes are locations in the standing wave pattern where the string doesn't move at all, and hence the traces on the figure intersect. In between nodes are antinodes, where the string moves up and down the most. This figure(Figure 3) shows the first three standing wave patterns that fit on any string with length L tied down at both ends. A pattern's number n is the number of antinodes it contains. The wavelength of the nth pattern is denoted A. The nth pattern has n half-wavelengths along the length of the string, so n = L Thus the wavelength of the nth pattern is Y Part B A₁ = This standing wave pattern has three antinodes, at x = 10 cm, 30 cm, and 50 cm. The pattern also has four nodes, at z = 0 cm, 20 cm, 40 cm, and 60 cm. Notice that the spacing between adjacent antinodes is only half of one wavelength, not one full wavelength. The same is true for the spacing between adjacent nodes. What is the wavelength of the longest wavelength standing wave pattern that can fit on this guitar string? Express your answer in centimeters. ▸ View Available Hint(s) Submit -VE| ΑΣΦ| < 6 of 15 > 4 WC ? A₁ = 2L cm Review
Item 6 Learning Goal: To understand standing waves, including calculation of A and f, and to learn the physical meaning behind some musical terms. The columns in the figure (Eigure 1) show the instantaneous shape of a vibrating guitar string drawn every 1 ms. The guitar string is 60 cm long. The left column shows the guitar string shape as a sinusoidal traveling wave passes through it. Notice that the shape is sinusoidal at all times and specific features, such as the crest indicated with the arrow, travel along the string to the right at a constant speed. The right column shows snapshots of the sinusoidal standing wave formed when this sinusoidal traveling wave passes through an identically shaped wave moving in the opposite direction on the same guitar string. The string is momentarily flat when the underlying traveling waves are exactly out of phase. The shape is sinusoidal with twice the original amplitude when the underlying waves are momentarily in phase. This pattern is called a standing wave because no wave features travel down the length of the string. Figure Time Traveling Wave 0 ms 1 ms 2 ms 3 ms I x= 0cm 118/8 Standing Wave II x= x= 60 cm 0 cm 60 cm 1 of 3 > ▼ Standing waves on a guitar string form when waves traveling down the string reflect off a point where the string is tied down or pressed against the fingerboard. The entire series of distortions may be superimposed on a single figure, like this (Eigure 2), indicating different moments in time using traces of different colors or line styles. Part A What is the wavelength of the standing wave shown on the guitar string? Express your answer in centimeters. ▸ View Available Hint(s) A = 40 cm V Previous Answers Correct Nodes are locations in the standing wave pattern where the string doesn't move at all, and hence the traces on the figure intersect. In between nodes are antinodes, where the string moves up and down the most. This figure(Figure 3) shows the first three standing wave patterns that fit on any string with length L tied down at both ends. A pattern's number n is the number of antinodes it contains. The wavelength of the nth pattern is denoted A. The nth pattern has n half-wavelengths along the length of the string, so n = L Thus the wavelength of the nth pattern is Y Part B A₁ = This standing wave pattern has three antinodes, at x = 10 cm, 30 cm, and 50 cm. The pattern also has four nodes, at z = 0 cm, 20 cm, 40 cm, and 60 cm. Notice that the spacing between adjacent antinodes is only half of one wavelength, not one full wavelength. The same is true for the spacing between adjacent nodes. What is the wavelength of the longest wavelength standing wave pattern that can fit on this guitar string? Express your answer in centimeters. ▸ View Available Hint(s) Submit -VE| ΑΣΦ| < 6 of 15 > 4 WC ? A₁ = 2L cm Review
College Physics
11th Edition
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Raymond A. Serway, Chris Vuille
Chapter1: Units, Trigonometry. And Vectors
Section: Chapter Questions
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...
Related questions
Question

Transcribed Image Text:Figure
bod
0 10 20 30
x (cm)
40 50 60
<
2 of 3
>

Transcribed Image Text:Item 6
Learning Goal:
To understand standing waves, including calculation of X and f, and to
learn the physical meaning behind some musical terms.
The columns in the figure (Figure 1) show the instantaneous shape of a
vibrating guitar string drawn every 1 ms. The guitar string is 60 cm long.
The left column shows the guitar string shape as a sinusoidal traveling wave
passes through it. Notice that the shape is sinusoidal at all times and
specific features, such as the crest indicated with the arrow, travel along the
string to the right at a constant speed.
The right column shows snapshots of the sinusoidal standing wave formed
when this sinusoidal traveling wave passes through an identically shaped
wave moving in the opposite direction on the same guitar string. The string
is momentarily flat when the underlying traveling waves are exactly out of
phase. The shape is sinusoidal with twice the original amplitude when the
underlying waves are momentarily in phase. This pattern is called a
standing wave because no wave features travel down the length of the
string.
Figure
Time Traveling Wave
0 ms
1 ms
2 ms
3 ms
I
X=
0 cm
X
Standing Wave
I
X=
X=
60 cm 0 cm
·X
X
I
X=
60 cm
1 of 3
Part A
Standing waves on a guitar string form when waves traveling down the string reflect off a point where the string is tied down or pressed against the fingerboard. The entire series of
distortions may be superimposed on a single figure, like this (Figure 2), indicating different moments in time using traces of different colors or line styles.
What is the wavelength of the standing wave shown on the guitar string?
Express your answer in centimeters.
► View Available Hint(s)
λ = 40 cm
Submit
Previous Answers
Correct
Nodes are locations in the standing wave pattern where the string doesn't move at all, and hence the traces on the figure intersect. In between nodes are antinodes,
where the string moves up and down the most.
This figure(Figure 3) shows the first three standing wave patterns that fit on any string with length L tied down at both ends. A pattern's number n is the number of antinodes it
contains. The wavelength of the nth pattern is denoted An. The nth pattern has n half-wavelengths along the length of the string, so
Thus the wavelength of the nth pattern is
Part B
λι =
This standing wave pattern has three antinodes, at x = 10 cm, 30 cm, and 50 cm. The pattern also has four nodes, at x = 0 cm, 20 cm, 40 cm, and 60 cm. Notice
that the spacing between adjacent antinodes is only half of one wavelength, not one full wavelength. The same is true for the spacing between adjacent nodes.
Submit
—| ΑΣΦ
?
nt:
What is the wavelength of the longest wavelength standing wave pattern that can fit on this guitar string?
Express your answer in centimeters.
► View Available Hint(s)
cm
Xn
6 of 15 >
= L
=
2L
n
Review
Expert Solution
Step 1
A)
The total length of the wave is .
So, the wavelength of the standing wave on the guitar string will be as follows.
Trending now
This is a popular solution!
Step by step
Solved in 2 steps

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
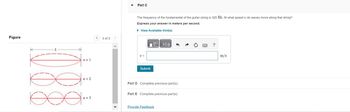
Transcribed Image Text:Figure
1000
n = 1
n=2
n=3
<
3 of 3
▼
Part C
The frequency of the fundamental of the guitar string is 320 Hz. At what speed v do waves move along that string?
Express your answer in meters per second.
► View Available Hint(s)
V =
Submit
Π| ΑΣΦ
Part D Complete previous part(s)
Part E Complete previous part(s)
Provide Feedback
?
m/s
Solution
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Recommended textbooks for you

College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning

University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON

Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press

College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning

University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON

Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press

Physics for Scientists and Engineers
Physics
ISBN:
9781337553278
Author:
Raymond A. Serway, John W. Jewett
Publisher:
Cengage Learning

Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:
9780321820464
Author:
Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:
Addison-Wesley

College Physics: A Strategic Approach (4th Editio…
Physics
ISBN:
9780134609034
Author:
Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:
PEARSON