Let n = 13 and let o E S13 be defined by o(1) = 12, o(2) = 13, o(3) = 3, o (6) = 9, o (7) = 5, o (8) = 10, o(11)=7, o(12)=8, o (13) = 2. o (4) = 1, o (9) = 6, o (5) = 11, o (10) = 4,
Let n = 13 and let o E S13 be defined by o(1) = 12, o(2) = 13, o(3) = 3, o (6) = 9, o (7) = 5, o (8) = 10, o(11)=7, o(12)=8, o (13) = 2. o (4) = 1, o (9) = 6, o (5) = 11, o (10) = 4,
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
This is an example of cyclic decomposition, I dont really get how each permutation goes to the other so if able please show how they get to those answers, thank you in advance.

Transcribed Image Text:Let n = 13 and let o E S₁3 be defined by
o(1) = 12,
o (2) = 13,
o (3) = 3,
o (7) = 5,
0 (8) = 10,
σ (6) = 9,
o(11) = 7,
o(12) = 8,
o (13) = 2.
o (4) = 1,
=
= 6,
σ(9)
o (5) = 11,
o (10) = 4,
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 3 steps with 3 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
how is the permutation of one equal to 12? and so on? The following table is given underneath the example above but I really still dont have a clue
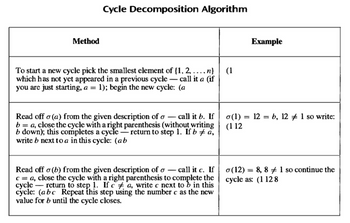
Transcribed Image Text:Method
Cycle Decomposition Algorithm
(1
To start a new cycle pick the smallest element of {1, 2, .... n}
which has not yet appeared in a previous cycle - call it a (if
you are just starting, a = 1); begin the new cycle: (a
Read off o(a) from the given description of o-call it b. If
b = a, close the cycle with a right parenthesis (without writing
b down); this completes a cycle return to step 1. If b + a,
write b next to a in this cycle: (ab
Read off o (b) from the given description of o-call it c. If
c = a, close the cycle with a right parenthesis to complete the
cycle return to step 1. If ca, write c next to b in this
cycle: (abc Repeat this step using the number c as the new
value for b until the cycle closes.
Example
σ(1) = 12 = b, 12 #1 so write:
(112
o(12) = 8,8 #1 so continue the
cycle as: (1128
Solution
Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

