Answered: Let {Xi} be i.i.d with the pdf f(x; θ)…

Algebra & Trigonometry with Analytic Geometry

13th Edition

ISBN:9781133382119

Author:Swokowski

Publisher:Swokowski

Chapter7: Analytic Trigonometry

Section7.6: The Inverse Trigonometric Functions

Problem 92E

See similar textbooks

Related questions

Topic Video

Question

Let {Xi} be i.i.d with the pdf

f(x; θ) =c/x^(θ+1) x > 1,

where θ > 0 is the unknown parameter.

(a) Determine c in term of θ.

(b) Given a sample {xi}i=1,...,n, find the MLE estimator θm= θm(x1, . . . , xn) for θ.

(c)Let Z =X1^(-θ)+...+Xn^(-θ),

Prove that θm and Z are independent.

Definition Definition Probability of occurrence of a continuous random variable within a specified range. When the value of a random variable, Y, is evaluated at a point Y=y, then the probability distribution function gives the probability that Y will take a value less than or equal to y. The probability distribution function formula for random Variable Y following the normal distribution is: F(y) = P (Y ≤ y) The value of probability distribution function for random variable lies between 0 and 1.

Expert Solution

Step 1

Given information:

Let X₁, X₂, X₃,…, X_n be independently and identically distributed random variables. The probability density function is as given below:

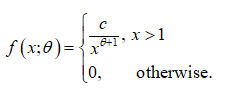
Trending now

This is a popular solution!

Step by step

Solved in 3 steps with 4 images

SEE SOLUTION Check out a sample Q&A here

Knowledge Booster

$Chain Rule$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.

Let {Xi} be i.i.d with the pdf f(x; θ) =c/x^(θ+1) x > 1, where θ > 0 is the unknown parameter. (a) Determine c in term of θ. (b) Given a sample {xi}i=1,...,n, fi