Let ø = $(x), u = u(x), and T = T(x) be differentiable scalar, vector, and tensor fields, where x is the position vector. Show that
Arc Length
Arc length can be thought of as the distance you would travel if you walked along the path of a curve. Arc length is used in a wide range of real applications. We might be interested in knowing how far a rocket travels if it is launched along a parabolic path. Alternatively, if a curve on a map represents a road, we might want to know how far we need to drive to get to our destination. The distance between two points along a curve is known as arc length.
Line Integral
A line integral is one of the important topics that are discussed in the calculus syllabus. When we have a function that we want to integrate, and we evaluate the function alongside a curve, we define it as a line integral. Evaluation of a function along a curve is very important in mathematics. Usually, by a line integral, we compute the area of the function along the curve. This integral is also known as curvilinear, curve, or path integral in short. If line integrals are to be calculated in the complex plane, then the term contour integral can be used as well.
Triple Integral
Examples:

![The equation shown is a vector calculus identity involving the divergence of a product of a scalar function (φ) and a tensor field (T). It can be expressed as follows:
**Equation:**
\[
\text{div} \, (\phi \mathbf{T}) = \mathbf{T}^\mathrm{T} \, \text{grad} \, \phi + \phi \, \text{div} \, \mathbf{T}
\]
**Description:**
- **div**: Represents the divergence operator. It is a measure of the rate at which a vector field spreads out from a given point.
- **φ (phi)**: Denotes a scalar function.
- **T**: Represents a tensor field.
- **Tᵀ**: Denotes the transpose of the tensor T.
- **grad φ**: Represents the gradient of φ, which is a vector field that points in the direction of the greatest rate of increase of φ and whose magnitude is the greatest rate of change.
**Explanation:**
This identity expands the divergence of the product of a scalar and a tensor into two terms:
1. The first term, \( \mathbf{T}^\mathrm{T} \, \text{grad} \, \phi \), represents the interaction between the transpose of the tensor field and the gradient of the scalar field.
2. The second term, \( \phi \, \text{div} \, \mathbf{T} \), denotes the product of the scalar field and the divergence of the tensor field.
This relationship is often used in mathematical physics and engineering, particularly in continuum mechanics, to simplify and solve equations involving complex field interactions.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2Fedadeb2e-cdac-43d1-98ad-015a8ab931d0%2F37d63f7c-c471-4510-86f4-da6afa1535ba%2Fim0v6qi_processed.png&w=3840&q=75)
According to the given information,
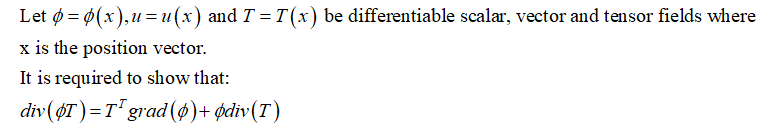
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images









