Homework Help is Here – Start Your Trial Now!
Math
Geometry
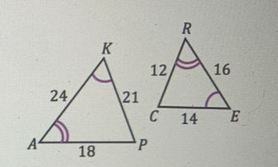
K Example 2: Are the 2 polygons similar? 12 If they are, write a similarity statement. 24 21 C 14 Hint: The easiest way to match up the sides is to compare the numbers. Make the first fraction with the 2 smallest 18 numbers (1 from each triangle). Then make a fraction with the 2 largest numbers. Hint: You can also compare the decimal numbers when finding the scale factor. 4.8 and 8.4 10 For example: if you had sides with the following numbers both equal 0.57142.. - 17.5 That means the 2 fractions have the same scale factor.

K Example 2: Are the 2 polygons similar? 12 If they are, write a similarity statement. 24 21 C 14 Hint: The easiest way to match up the sides is to compare the numbers. Make the first fraction with the 2 smallest 18 numbers (1 from each triangle). Then make a fraction with the 2 largest numbers. Hint: You can also compare the decimal numbers when finding the scale factor. 4.8 and 8.4 10 For example: if you had sides with the following numbers both equal 0.57142.. - 17.5 That means the 2 fractions have the same scale factor.

Elementary Geometry For College Students, 7e
Elementary Geometry For College Students, 7e
7th Edition
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Alexander, Daniel C.; Koeberlein, Geralyn M.
ChapterP: Preliminary Concepts
SectionP.CT: Test
Problem 1CT
See similar textbooks
icon
Related questions
Question
Example 2: Are the 2 polygons similar? If they are, write a similarity statement. **Hint:** The easiest way to match up the sides is to compare the numbers. Make the first fraction with the 2 smallest numbers (1 from each triangle). Then make a fraction with the 2 largest numbers. **Hint:** You can also compare the decimal numbers when finding the scale factor. For example: if you had sides with the following numbers \(\frac{4.8}{8.4}\) and \(\frac{10}{17.5}\) both equal 0.57142... That means the 2 fractions have the same scale factor. **Diagram Details:** - The first triangle, \(\triangle AKP\), has sides labeled with lengths 24, 21, and 18. - The second triangle, \(\triangle RCE\), has sides labeled with lengths 12, 16, and 14. - Both triangles have corresponding angles marked.
Transcribed Image Text:Example 2: Are the 2 polygons similar? If they are, write a similarity statement. **Hint:** The easiest way to match up the sides is to compare the numbers. Make the first fraction with the 2 smallest numbers (1 from each triangle). Then make a fraction with the 2 largest numbers. **Hint:** You can also compare the decimal numbers when finding the scale factor. For example: if you had sides with the following numbers \(\frac{4.8}{8.4}\) and \(\frac{10}{17.5}\) both equal 0.57142... That means the 2 fractions have the same scale factor. **Diagram Details:** - The first triangle, \(\triangle AKP\), has sides labeled with lengths 24, 21, and 18. - The second triangle, \(\triangle RCE\), has sides labeled with lengths 12, 16, and 14. - Both triangles have corresponding angles marked.
Expert Solution
Step 1

Here we have to state the following polygon is similar.

Given:-

Geometry homework question answer, step 1, image 1

 

steps

Step by step

Solved in 2 steps with 1 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Recommended textbooks for you
Elementary Geometry For College Students, 7e
Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,
Elementary Geometry for College Students
Elementary Geometry for College Students
Geometry
ISBN:
9781285195698
Author:
Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:
Cengage Learning
SEE MORE TEXTBOOKS