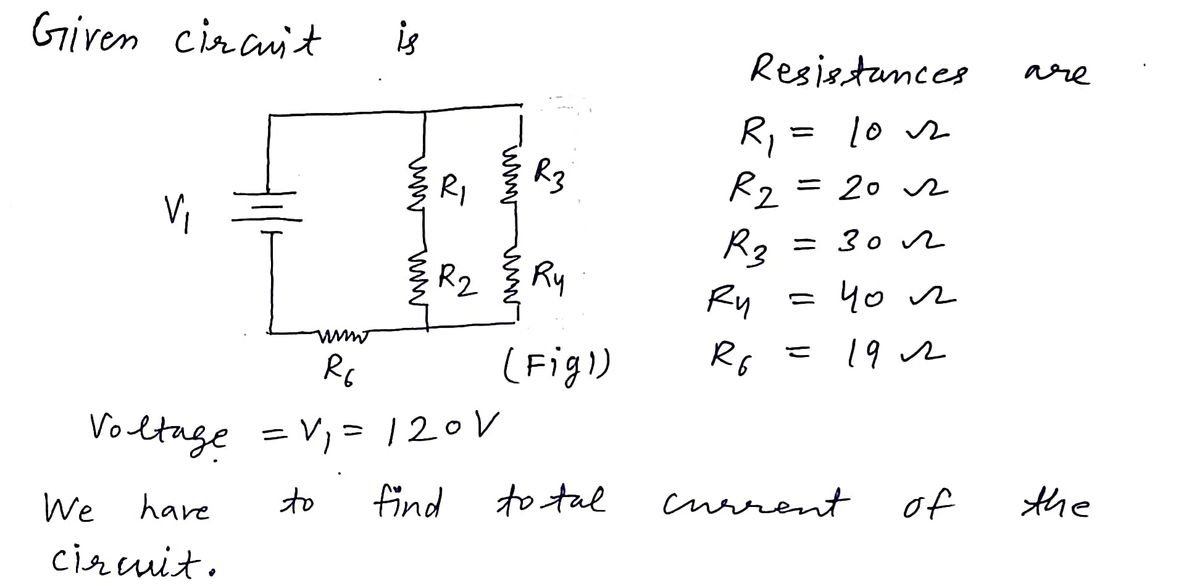
In this problem, a circuit with a number of resistors connected in series and parallel connection with a supply DC voltage is shown in the figure. The supply voltage of, \( V_{\text{Supply}} = 120 \, \text{V} \), resistances, \( R_1 = 10\Omega \), \( R_2 = 20\Omega \), \( R_3 = 30\Omega \), and \( R_4 = 40\Omega \), \( R_6 = 19\Omega \) (\( R_5 \) is not connected). What is the value of the total current of the circuit in amps?### Diagram Explanation:The diagram depicts a circuit configuration with:- A voltage supply labeled as \( 120 \, \text{V} \).- Various resistors labeled with resistances: - \( R_1: 10\Omega \) - \( R_2: 20\Omega \) - \( R_3: 30\Omega \) - \( R_4: 40\Omega \) - \( R_6: 19\Omega \)Note: The resistor \( R_5 \) is indicated, but it is stated to be not connected, therefore it does not impact the circuit’s current or resistance calculations.The circuit displays resistors arranged in a combination of series and parallel configurations, affecting the total resistance and current calculation.### Calculation:To calculate the total current, we need to determine the equivalent resistance of the circuit and use Ohm's Law:\[ I_{\text{total}} = \frac{V_{\text{Supply}}}{R_{\text{equivalent}}} \]1. Identify series and parallel groups of resistors.2. Calculate the equivalent resistance for each group.3. Sum the resistances to find the total resistance.4. Use the voltage supply value to find the total current.

Answered: Image /qna-images/answer/0391a2ad-7dbe-48fe-8967-c2e768d3ce14.jpg

Answered: In this problem, a circuit with number…

Similar questions

A battery with voltage V is connected to an Ohmic resistor resulting in a current of I flowing in the circuit. If the voltage is tripled what is the resulting current?
Several resistors in a circuit have a theoretical combined resistance of 107 ohms. When the resistance is found through measurements of voltage and current in the circuit, the value is 99 ohms. What is the percent error? 0.075% O 8.08% O 0.081% 7.18 %
A resistor is placed in a circuit with an adjustable voltage source. The voltage across and the current through the resistor and the measurements are shown below. Estimate the resistance of the resistor.
Inew A circuit with a resistance R = 73 Q is connected to a battery with potential difference across the terminals of AV = 8.5 V. Input an expression for the current passing through the circuit, I. What is the current in milliamps, mA? If the resistance of the circuit was increased by a factor of ten, Rnew = 10R, what is the new current in milliamps, mA?
In the circuit shown below, Vs = 60 V, R1 = 7 Q, R2 =10 V, R3 = 6 Q and R4 = 6 Q. The Thevenin equivalent of this circuit consists of a voltage source Vth and series resistance RTh. FInd Vth and Rth and hence determine the current that will flow through a 50 Q resistor connected between points A and B. Give your answer in Amps to three decimal places. RTh I R3 R2 TA Vs VTh B Next > < Previous R4 R1 That -A B
R, 125 2 R, 24.0 V 360 2 Consider the circuit above. If R1 = 144 ohms and R2 = 176 ohms, what is the equivalent resistance in ohms of the circuit? (Enter answer as a number with 1 digit right of the decimal. Do not enter unit.)
The combined electrical resistance R of two resistors R1 and R2, connected in parallel, is given by the equation below, where R, R1, and R2 are measured in ohms. R1 and R2 are increasing at rates of 0.9 and 2.0 ohms per second, respectively. 1 = 1 + 1 At what rate is R changing when R1 = 37 ohms and R2 = 87 ohms? (Round your answer to three decimal places.)
I need a labled schematic on paper of the circuit showing + and - terminals of the battery, the direction of any current flowing through the circuit, and any voltage drop across any resistors. I also need the form of the equations dealing with I, R (or 1/R), and V equations filled in with the appropriate numbers and answer with units for this problem. Problem: The same two resistors are connected in parallel to a voltage source. The voltage source (∆Vtot) is a 48-V source and the resistor values are 6.4 Ω (R1) and 3.9 Ω (R2). Determine the equivalent resistance of the circuit. Determine the current in the circuit. Determine the voltage drop across each individual resistor.
Applying Kirchoff's Rules If in the circuit shown, V1 = 4V, V2 = 12V, R1 = 3 Ohms, R2 = 1 Ohm, and R3 = 3 Ohms, the current I₁ is amps, the current I2 is amps, the current I3 is decimal point.) + V1 R2 amps. (Provide answer with one digit to the right of the R3 I2 13 R1 www V2 +
9geu in as Gavin Ritche R2 R1 20 V 15 Q What is the equivalent resistance of the parallel circuit if R2 is 17 ohms? Answer: Next page
R₁ R₂ ww R 6 R 5
You have a circuit made of a 7 V source and a 1.5k resistor. What is the currentthrough the resistor and the voltage drop across the resistor?

SEE MORE QUESTIONS