Homework Help is Here – Start Your Trial Now!
Science
Physics
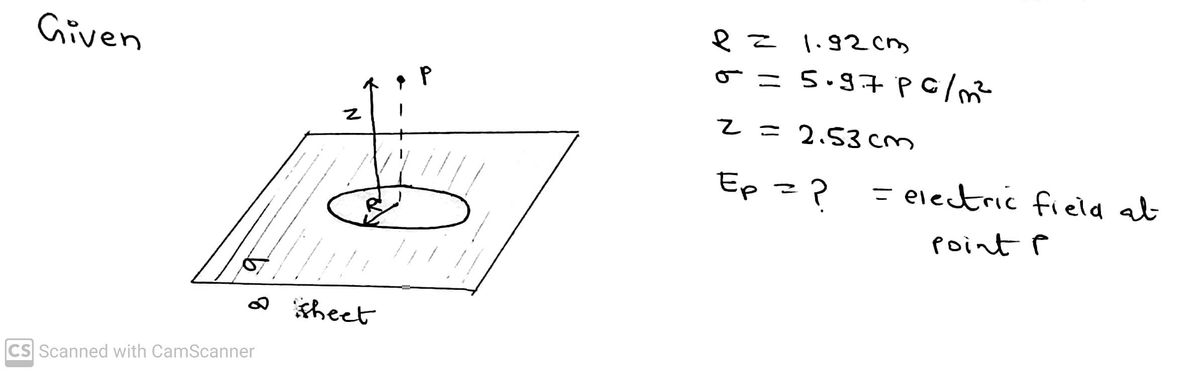
In the figure a small circular hole of radius R= 1.92 cm has been cut in the middle of an infinite, flat, nonconducting surface that has a uniform charge density o = 5.97 pC/m?. Az axis, with its origin at the hole's center, is perpendicular to the surface. What is the magnitude of the electric field at point Pat z = 2.53 cm? (Hint: See equation E = 1 - and use superposition.) 2e0 2 + R Number i 0.2687 Units N/C or V/m

In the figure a small circular hole of radius R= 1.92 cm has been cut in the middle of an infinite, flat, nonconducting surface that has a uniform charge density o = 5.97 pC/m?. Az axis, with its origin at the hole's center, is perpendicular to the surface. What is the magnitude of the electric field at point Pat z = 2.53 cm? (Hint: See equation E = 1 - and use superposition.) 2e0 2 + R Number i 0.2687 Units N/C or V/m

College Physics
College Physics
11th Edition
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Raymond A. Serway, Chris Vuille
Chapter1: Units, Trigonometry. And Vectors
Section: Chapter Questions
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...
See similar textbooks
icon
Related questions
Question
**Description of the Image for Educational Website** The image presents a physics problem involving an electric field on an infinite, flat, nonconducting surface with a small circular hole. Here's a breakdown of the elements involved: ### Problem Statement: - **Surface Characteristics:** - An infinite flat, nonconducting surface. - Uniform charge density, \(\sigma = 5.97 \, \text{pC/m}^2\). - **Hole Specifications:** - Circular hole with a radius, \(R = 1.92 \, \text{cm}\). - **Axis and Point Specification:** - A z-axis is set with its origin at the center of the hole, perpendicular to the surface. - The point \(P\) is located at \(z = 2.53 \, \text{cm}\). ### Objective: - To determine the magnitude of the electric field at point \(P\). - Equation provided for calculation: \[ E = \frac{\sigma}{2\varepsilon_0} \left( 1 - \frac{z}{\sqrt{z^2 + R^2}} \right) \] - Recommendations to use the method of superposition are given in the hint. ### Graphical Description: - The illustration depicts a green plane representing the nonconducting surface. - A dotted circular line indicates the hole in the surface. - The z-axis is marked, showing its perpendicular orientation to the flat plane, with a point \(P\) identified above the plane along this axis. ### Solution: - The input field shows a calculated value of the electric field magnitude at point \(P\): \(0.2687 \, \text{N/C or V/m}\). This setup is used to explore concepts of electric fields, charge distribution, and the effects of geometry on field calculations in electrostatics.
Transcribed Image Text:**Description of the Image for Educational Website** The image presents a physics problem involving an electric field on an infinite, flat, nonconducting surface with a small circular hole. Here's a breakdown of the elements involved: ### Problem Statement: - **Surface Characteristics:** - An infinite flat, nonconducting surface. - Uniform charge density, \(\sigma = 5.97 \, \text{pC/m}^2\). - **Hole Specifications:** - Circular hole with a radius, \(R = 1.92 \, \text{cm}\). - **Axis and Point Specification:** - A z-axis is set with its origin at the center of the hole, perpendicular to the surface. - The point \(P\) is located at \(z = 2.53 \, \text{cm}\). ### Objective: - To determine the magnitude of the electric field at point \(P\). - Equation provided for calculation: \[ E = \frac{\sigma}{2\varepsilon_0} \left( 1 - \frac{z}{\sqrt{z^2 + R^2}} \right) \] - Recommendations to use the method of superposition are given in the hint. ### Graphical Description: - The illustration depicts a green plane representing the nonconducting surface. - A dotted circular line indicates the hole in the surface. - The z-axis is marked, showing its perpendicular orientation to the flat plane, with a point \(P\) identified above the plane along this axis. ### Solution: - The input field shows a calculated value of the electric field magnitude at point \(P\): \(0.2687 \, \text{N/C or V/m}\). This setup is used to explore concepts of electric fields, charge distribution, and the effects of geometry on field calculations in electrostatics.
Expert Solution
Step 1

Physics homework question answer, step 1, image 1

steps

Step by step

Solved in 2 steps with 2 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Similar questions
Recommended textbooks for you
College Physics
College Physics
Physics
ISBN:
9781305952300
Author:
Raymond A. Serway, Chris Vuille
Publisher:
Cengage Learning
University Physics (14th Edition)
University Physics (14th Edition)
Physics
ISBN:
9780133969290
Author:
Hugh D. Young, Roger A. Freedman
Publisher:
PEARSON
Introduction To Quantum Mechanics
Introduction To Quantum Mechanics
Physics
ISBN:
9781107189638
Author:
Griffiths, David J., Schroeter, Darrell F.
Publisher:
Cambridge University Press
Physics for Scientists and Engineers
Physics for Scientists and Engineers
Physics
ISBN:
9781337553278
Author:
Raymond A. Serway, John W. Jewett
Publisher:
Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:
9780321820464
Author:
Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:
Addison-Wesley
College Physics: A Strategic Approach (4th Editio…
College Physics: A Strategic Approach (4th Editio…
Physics
ISBN:
9780134609034
Author:
Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:
PEARSON
SEE MORE TEXTBOOKS