In recent years, many states have enacted tougher voter identification laws requiring a government issued photo ID. Proponents claim that these laws are necessary to reduce voter fraud. Opponents claim that voter fraud is rare and that since as many as 11% of eligible voters do not have the required identification, these tougher laws will discourage millions of minorities and low-income Americans from voting. The Washington Post conducted a national poll (July 7-19, 2012) and found that 1558 out of 2064 adults supported the tougher voter ID laws. 1)Construct a 95% confidence interval for the proportion of adults who approve of tougher voter ID laws. Document your work in the space provided below. Document how you computed the margin of error and the confidence interval in the space provided below. Round all numbers to 4 decimal places. 2)Is it likely that three-fourths of adults approve of these new laws?
In recent years, many states have enacted tougher voter identification laws requiring a government issued photo ID. Proponents claim that these laws are necessary to reduce voter fraud. Opponents claim that voter fraud is rare and that since as many as 11% of eligible voters do not have the required identification, these tougher laws will discourage millions of minorities and low-income Americans from voting. The Washington Post conducted a national poll (July 7-19, 2012) and found that 1558 out of 2064 adults supported the tougher voter ID laws.
1)Construct a 95% confidence interval for the proportion of adults who approve of tougher voter ID laws. Document your work in the space provided below. Document how you computed the margin of error and the confidence interval in the space provided below. Round all numbers to 4 decimal places.
2)Is it likely that three-fourths of adults approve of these new laws?
(1)
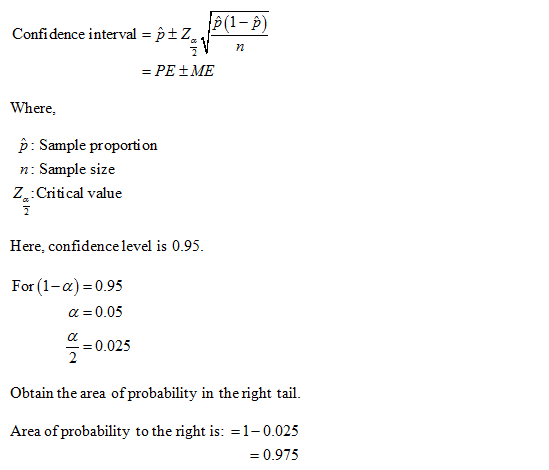
Obtain the 95% confidence interval for the proportion of adults who approve of tougher voter ID laws.
The 95% confidence interval for the proportion of adults who approve of tougher voter ID laws is obtained below as follows:
From the information, given that n=2064,x=1558.
The formula for the confidence interval for the proportion is,
Use EXCEL Procedure for finding the critical value of z.
Follow the instruction to obtain the critical value of z:
- Open EXCEL
- Go to Formula bar.
- In formula bar enter the function as“=NORMINV”
- Enter the probability as 0.975
- Enter the mean as 0 and the Standard deviation as 1.
- Click enter
EXCEL output:
From the EXCEL output, the critical value of z at the 95% confidence level is 1.96.
Step by step
Solved in 2 steps with 2 images









