Homework Help is Here – Start Your Trial Now!
Math
Statistics
If the coefficient B₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If B₁ = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that B₁ = 0 use the test statistic t = (b₁ - 0) /sp. Critical values or P-values can be found using the t distribution with n - (k+1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error sp, is often provided by software. For example, see the accompanying technology display, which shows that sp, = 0.070306916 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the technology display to test the claim that B₁ = 0. Also test the claim that B₂ = 0. What do the results imply about the regression equation? Technology Output Parameter estimates: Parameter Estimate Std. Err. - 147.38571 12.118648 0.75317073 0.070306916 1.0419968 0.030863713 Intercept Height Waist Alternative DF+ T-Stat #0 150 12.161894 #0 150 #0 150 10.712612 33.761226 P-value - X <0.0001 <0.0001 <0.0001 ression coefficient

If the coefficient B₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If B₁ = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that B₁ = 0 use the test statistic t = (b₁ - 0) /sp. Critical values or P-values can be found using the t distribution with n - (k+1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error sp, is often provided by software. For example, see the accompanying technology display, which shows that sp, = 0.070306916 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the technology display to test the claim that B₁ = 0. Also test the claim that B₂ = 0. What do the results imply about the regression equation? Technology Output Parameter estimates: Parameter Estimate Std. Err. - 147.38571 12.118648 0.75317073 0.070306916 1.0419968 0.030863713 Intercept Height Waist Alternative DF+ T-Stat #0 150 12.161894 #0 150 #0 150 10.712612 33.761226 P-value - X <0.0001 <0.0001 <0.0001 ression coefficient

MATLAB: An Introduction with Applications
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
See similar textbooks
icon
Related questions
Question

78

If the coefficient B₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If B₁ = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that B₁ = 0 use the test statistic t = (b₁ - 0) /sp. Critical values or P-values can be found using the t distribution with n - (k+1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error sp, is often provided by software. For example, see the accompanying technology display, which shows that sp, = 0.070306916 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the technology display to test the claim that B₁ = 0. Also test the claim that B₂ = 0. What do the results imply about the regression equation? Technology Output Parameter estimates: Intercept Parameter Estimate Std. Err. - 147.38571 12.118648 0.75317073 0.070306916 1.0419968 0.030863713 Height Waist Alternative DF+ T-Stat #0 150 12.161894 #0 150 #0 150 10.712612 33.761226 P-value - X <0.0001 <0.0001 <0.0001 ression coefficient
Transcribed Image Text:If the coefficient B₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If B₁ = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that B₁ = 0 use the test statistic t = (b₁ - 0) /sp. Critical values or P-values can be found using the t distribution with n - (k+1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error sp, is often provided by software. For example, see the accompanying technology display, which shows that sp, = 0.070306916 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the technology display to test the claim that B₁ = 0. Also test the claim that B₂ = 0. What do the results imply about the regression equation? Technology Output Parameter estimates: Intercept Parameter Estimate Std. Err. - 147.38571 12.118648 0.75317073 0.070306916 1.0419968 0.030863713 Height Waist Alternative DF+ T-Stat #0 150 12.161894 #0 150 #0 150 10.712612 33.761226 P-value - X <0.0001 <0.0001 <0.0001 ression coefficient
Expert Solution
trending now

Trending now

This is a popular solution!

steps

Step by step

Solved in 3 steps

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question

78

 

Answer was incorrect

If the coefficient B₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If B₁ = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that B₁ = 0 use the test statistic t= (b₁-0) /sp. Critical values or P-values can be found using the t distribution with n - (k+ 1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error sp, is often provided by software. For example, see the accompanying technology display, which shows that sp, = 0.070306916 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the technology display to test the claim that B₁ = 0. Also test the claim that B₂ = 0. What do the results imply about the regression equation? Click the icon to view the technology output. Test the claim that B₁ = 0. For Ho: B₁ = 0, the test statistic is t= 10.328 and the P-value is 0, so b₁ = 0.725 should be kept. (Round to three decimal places as needed.) reject Ho and conclude that the regression coefficient
Transcribed Image Text:If the coefficient B₁ has a nonzero value, then it is helpful in predicting the value of the response variable. If B₁ = 0, it is not helpful in predicting the value of the response variable and can be eliminated from the regression equation. To test the claim that B₁ = 0 use the test statistic t= (b₁-0) /sp. Critical values or P-values can be found using the t distribution with n - (k+ 1) degrees of freedom, where k is the number of predictor (x) variables and n is the number of observations in the sample. The standard error sp, is often provided by software. For example, see the accompanying technology display, which shows that sp, = 0.070306916 (found in the column with the heading of "Std. Err." and the row corresponding to the first predictor variable of height). Use the technology display to test the claim that B₁ = 0. Also test the claim that B₂ = 0. What do the results imply about the regression equation? Click the icon to view the technology output. 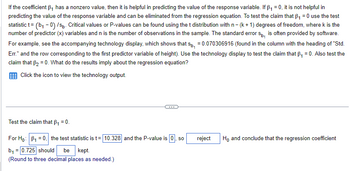Test the claim that B₁ = 0. For Ho: B₁ = 0, the test statistic is t= 10.328 and the P-value is 0, so b₁ = 0.725 should be kept. (Round to three decimal places as needed.) reject Ho and conclude that the regression coefficient
Solution
Bartleby Expert
SEE SOLUTION
Recommended textbooks for you
MATLAB: An Introduction with Applications
MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc
Probability and Statistics for Engineering and th…
Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning
Statistics for The Behavioral Sciences (MindTap C…
Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning
Elementary Statistics: Picturing the World (7th E…
Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON
The Basic Practice of Statistics
The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman
Introduction to the Practice of Statistics
Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman
SEE MORE TEXTBOOKS