Identify which congruence theorem applies in each case. a. Is AAMD = ARMC? b. Is AWOH = AWAT? c. Is AGAS = AIOL? D. R. 15 cm A H. 64° 64° 15 cm 46° 72 72° G 46° d. Is AAWL = AKLW? e. Is AHOW= ATAW? f. Is ABOX ACAR? W A
Identify which congruence theorem applies in each case. a. Is AAMD = ARMC? b. Is AWOH = AWAT? c. Is AGAS = AIOL? D. R. 15 cm A H. 64° 64° 15 cm 46° 72 72° G 46° d. Is AAWL = AKLW? e. Is AHOW= ATAW? f. Is ABOX ACAR? W A
Elementary Geometry For College Students, 7e
7th Edition
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Alexander, Daniel C.; Koeberlein, Geralyn M.
ChapterP: Preliminary Concepts
SectionP.CT: Test
Problem 1CT
Related questions
Question
Identify which congruence theorem applies in each case

Transcribed Image Text:### Identifying Triangle Congruence Theorems
For each pair of triangles, determine which congruence theorem applies, if any:
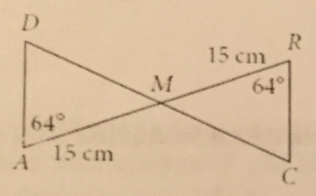
#### a. Is \(\triangle AMD \equiv \triangle RMC\)?
- **Diagram**: Two triangles sharing a vertex (\(M\)), forming an "X" shape.
- \(\angle AMD\) and \(\angle RMC\) both measure \(64^\circ\).
- \(AD = RC = 15 \, \text{cm}\).
#### b. Is \(\triangle WOH \equiv \triangle WAT\)?
- **Diagram**: Two triangles sharing the vertex \(W\).
- Vertical angles \(\angle WOH\) and \(\angle WAT\) are marked as equal.
#### c. Is \(\triangle GAS \equiv \triangle MOL\)?
- **Diagram**: Two independent triangles.
- Angles \(\angle G\) and \(\angle O\) both measure \(72^\circ\).
- Angles \(\angle S\) and \(\angle L\) both measure \(40^\circ\).
#### d. Is \(\triangle AWL \equiv \triangle KLW\)?
- **Diagram**: Two triangles sharing a side \(WL\).
#### e. Is \(\triangle AHOW \equiv \triangle TAWP\)?
- **Diagram**: Two triangles sharing a common side \(AW\).
#### f. Is \(\triangle BOX \equiv \triangle CAR\)?
- **Diagram**: Two triangles with equal marking on sides \(BO\) and \(AC\) and \(OX\) and \(AR\).
For each pair of triangles, apply the appropriate congruence theorem: Side-Angle-Side (SAS), Side-Side-Side (SSS), Angle-Side-Angle (ASA), or Angle-Angle-Side (AAS), if applicable.
Expert Solution
Step 1
Note:
Since you have posted questions with multiple subparts. We will solve the first three subparts for you. To get the remaining subparts to be solved please post the complete question and specify the number of subparts.
(a)
The figure given is
Step by step
Solved in 4 steps with 3 images

Recommended textbooks for you

Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,

Elementary Geometry for College Students
Geometry
ISBN:
9781285195698
Author:
Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:
Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:
9781337614085
Author:
Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:
Cengage,

Elementary Geometry for College Students
Geometry
ISBN:
9781285195698
Author:
Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:
Cengage Learning