Homework Help is Here – Start Your Trial Now!
Math
Algebra
How do you determine which value will become λ₁ vs λ₂? Why isn’t λ₁ = 2 and λ₂ = 4 in this example?

How do you determine which value will become λ₁ vs λ₂? Why isn’t λ₁ = 2 and λ₂ = 4 in this example?

Algebra and Trigonometry (6th Edition)
Algebra and Trigonometry (6th Edition)
6th Edition
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:Robert F. Blitzer
ChapterP: Prerequisites: Fundamental Concepts Of Algebra
Section: Chapter Questions
Problem 1MCCP: In Exercises 1-25, simplify the given expression or perform the indicated operation (and simplify,...
See similar textbooks
icon
Related questions
Question
How do you determine which value will become λ₁ vs λ₂? Why isn’t λ₁ = 2 and λ₂ = 4 in this example?
0 We first need the eigenvalues and eigenvectors: = det (A - XI₂) = 1 1 3-X = (A - 3)² - 1 = A²-6A +8 = (A-2) (X-4). 3-X This gives us the distinct, real eigenvalues λ₁ = 4 and X₂ = 2.
Transcribed Image Text:0 We first need the eigenvalues and eigenvectors: = det (A - XI₂) = 1 1 3-X = (A - 3)² - 1 = A²-6A +8 = (A-2) (X-4). 3-X This gives us the distinct, real eigenvalues λ₁ = 4 and X₂ = 2.
Expert Solution
steps

Step by step

Solved in 2 steps

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question

So when constructing a matrix using the eigenvectors, the order of the eigenvectors doesn't matter?

In the example they get P = ( [1, -1] , [ 1, 1]), because they put the eigenvector of eigenvalue 4 first and eigenvector of eigenvalue 2 second.

How would I know to take eigen value 4 first and not second to calculate eigenvectors?

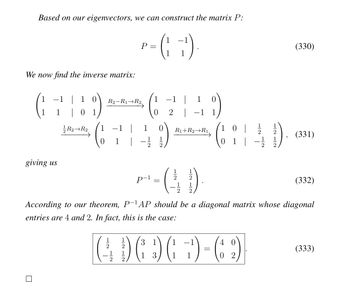
Based on our eigenvectors, we can construct the matrix P: P-(1-7) P= We now find the inverse matrix: 1 giving us -1 | | 0 1 R₂ R2 1 R2-R1 R2. R₁+R₂ 6 0 P-1 = -1 | T R1+R2 R1, 21 2 12 12 2, 0 1 3 (4D6969-69) -1 3/ 1 IN 12 12 2 1 (330) (331) According to our theorem, P-¹AP should be a diagonal matrix whose diagonal entries are and 2. In fact, this is the case: (332) (333)
Transcribed Image Text:Based on our eigenvectors, we can construct the matrix P: P-(1-7) P= We now find the inverse matrix: 1 giving us -1 | | 0 1 R₂ R2 1 R2-R1 R2. R₁+R₂ 6 0 P-1 = -1 | T R1+R2 R1, 21 2 12 12 2, 0 1 3 (4D6969-69) -1 3/ 1 IN 12 12 2 1 (330) (331) According to our theorem, P-¹AP should be a diagonal matrix whose diagonal entries are and 2. In fact, this is the case: (332) (333)
Solution
Bartleby Expert
SEE SOLUTION
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:
9780134463216
Author:
Robert F. Blitzer
Publisher:
PEARSON
Contemporary Abstract Algebra
Contemporary Abstract Algebra
Algebra
ISBN:
9781305657960
Author:
Joseph Gallian
Publisher:
Cengage Learning
Linear Algebra: A Modern Introduction
Linear Algebra: A Modern Introduction
Algebra
ISBN:
9781285463247
Author:
David Poole
Publisher:
Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:
9780135163078
Author:
Michael Sullivan
Publisher:
PEARSON
Introduction to Linear Algebra, Fifth Edition
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:
9780980232776
Author:
Gilbert Strang
Publisher:
Wellesley-Cambridge Press
College Algebra (Collegiate Math)
College Algebra (Collegiate Math)
Algebra
ISBN:
9780077836344
Author:
Julie Miller, Donna Gerken
Publisher:
McGraw-Hill Education
SEE MORE TEXTBOOKS