For the following information, determine whether a normal sampling distribution can be used, where p is the population proportion, a is the level of significance, p is the sample proportion, and n is the sample size. If it can be used, test the claim. Claim: p20.25; a = 0.08. Sample statistics: p= 0.20, n = 90 Let q = 1-p and let q = 1-p. A normal sampling distribution be used here, since 5 and
Percentage
A percentage is a number indicated as a fraction of 100. It is a dimensionless number often expressed using the symbol %.
Algebraic Expressions
In mathematics, an algebraic expression consists of constant(s), variable(s), and mathematical operators. It is made up of terms.
Numbers
Numbers are some measures used for counting. They can be compared one with another to know its position in the number line and determine which one is greater or lesser than the other.
Subtraction
Before we begin to understand the subtraction of algebraic expressions, we need to list out a few things that form the basis of algebra.
Addition
Before we begin to understand the addition of algebraic expressions, we need to list out a few things that form the basis of algebra.
I'm completly lost on the problem. Can you please help me. I'm confused om P and P

Determine whether the normal sampling distribution can be used or not.
The normal sampling distribution can be used or not is determined below as follows:

The value for and are both greater than 5, that is both the conditions are satisfied. Since both conditions are satisfied, this implies that all the assumptions have been satisfied.
Therefore, it can be concluded that
A normal sampling distribution can be used here, since np ≥ 5 and nq ≥ 5.
State the hypotheses.
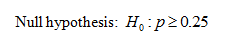
That is, there is no evidence that the true population proportion is less than 0.25.
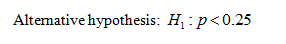
That is, there is evidence that the true population proportion is less than 0.25.
Trending now
This is a popular solution!
Step by step
Solved in 4 steps with 5 images









