Find the remainder when 819054 is divided by 13.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
![**Problem Statement:**
Find the remainder when \(8^{19054}\) is divided by 13.
**Explanation:**
This problem involves finding the remainder of a large power when divided by a number. It can often be solved using modular arithmetic techniques such as Fermat's Little Theorem, which states that if \(p\) is a prime number and \(a\) is an integer not divisible by \(p\), then \(a^{p-1} \equiv 1 \ (\text{mod} \ p)\).
Here, since 13 is a prime number, we can apply this theorem. Specifically:
1. Verify that 13 is a prime and does not divide 8.
2. Apply Fermat's Little Theorem:
\[
a^{p-1} \equiv 1 \ (\text{mod} \ p)
\]
For \(a = 8\) and \(p = 13\):
\[
8^{12} \equiv 1 \ (\text{mod} \ 13)
\]
3. Break down \(8^{19054}\) by expressing its exponent in terms of multiples of 12.
Please refer to mathematical methods such as modular arithmetic or Fermat's Little Theorem for further steps in solving such problems.](/v2/_next/image?url=https%3A%2F%2Fcontent.bartleby.com%2Fqna-images%2Fquestion%2F2a4399a9-5724-42c8-89a0-9bc27dd1a0f2%2F1371bbb0-42c5-4504-9936-e4237b3455d5%2Fc5m04p_processed.png&w=3840&q=75)
Transcribed Image Text:**Problem Statement:**
Find the remainder when \(8^{19054}\) is divided by 13.
**Explanation:**
This problem involves finding the remainder of a large power when divided by a number. It can often be solved using modular arithmetic techniques such as Fermat's Little Theorem, which states that if \(p\) is a prime number and \(a\) is an integer not divisible by \(p\), then \(a^{p-1} \equiv 1 \ (\text{mod} \ p)\).
Here, since 13 is a prime number, we can apply this theorem. Specifically:
1. Verify that 13 is a prime and does not divide 8.
2. Apply Fermat's Little Theorem:
\[
a^{p-1} \equiv 1 \ (\text{mod} \ p)
\]
For \(a = 8\) and \(p = 13\):
\[
8^{12} \equiv 1 \ (\text{mod} \ 13)
\]
3. Break down \(8^{19054}\) by expressing its exponent in terms of multiples of 12.
Please refer to mathematical methods such as modular arithmetic or Fermat's Little Theorem for further steps in solving such problems.
Expert Solution
Step 1
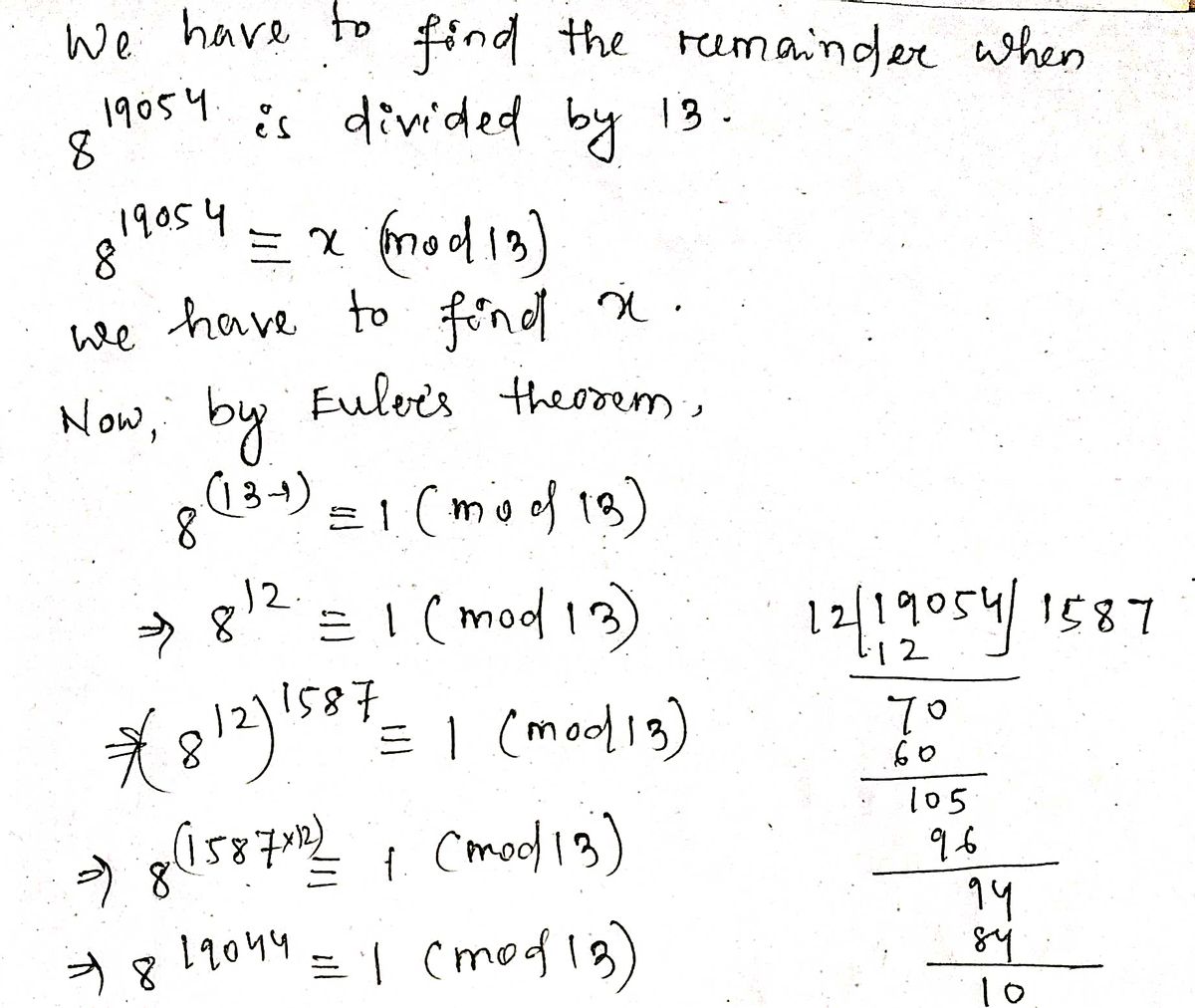
Step by step
Solved in 2 steps with 2 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

